The area of the region bounded by the curve y=2x−x2 and the line y=x is
A
2e∫1√logeydy
B
2e−1∫−1ex2dx
C
1∫−1(e−ex2)dx
D
21∫0√xexdx
Video Solution
Text Solution
The correct Answer is:A, B, C, D
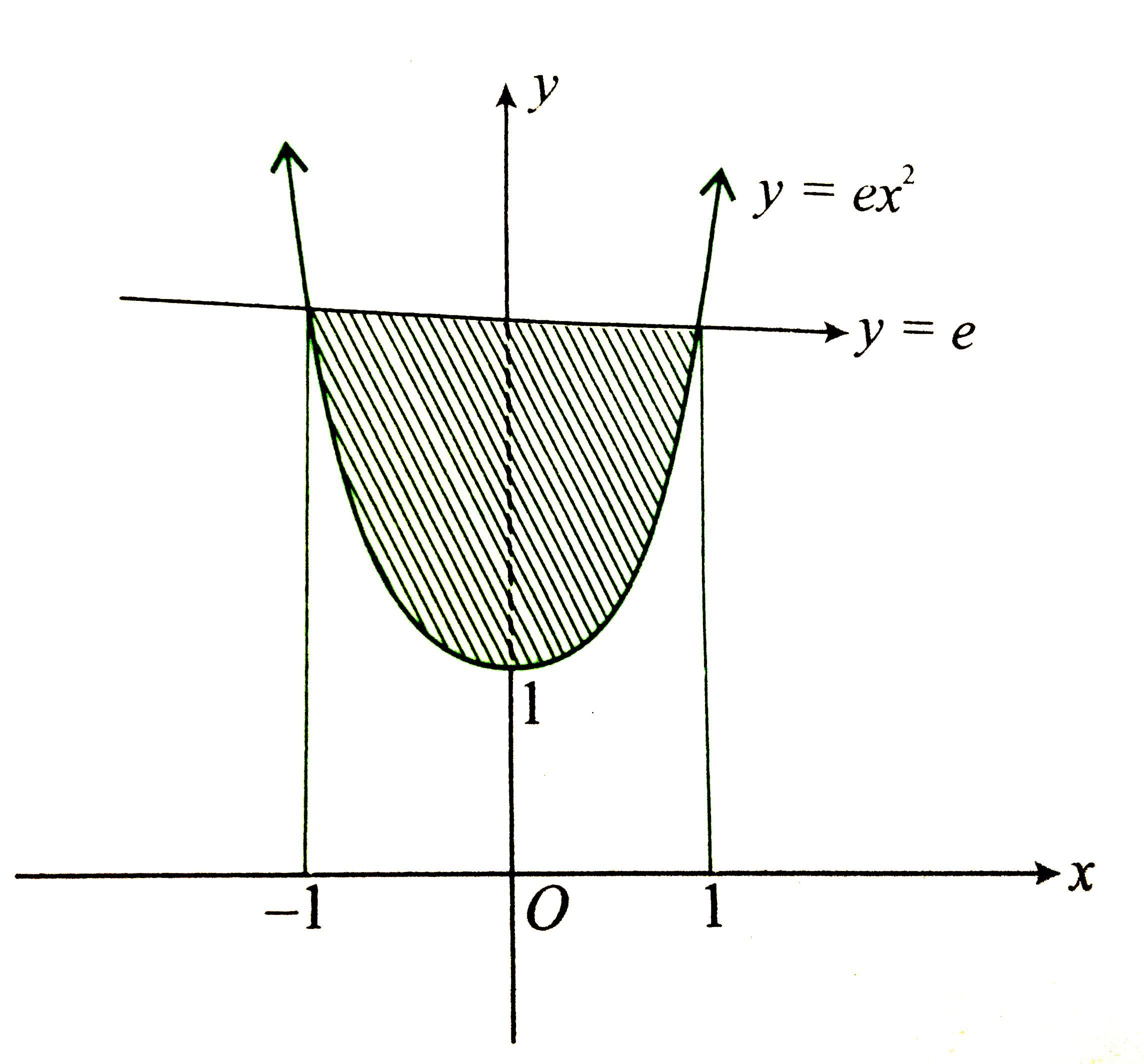
Area, A=1∫−1(e−ex2)dx=2e−1∫−1ex2dx
y=ex2
∴ logey=x2
∴ x=±√logey
∴ Area, A=2e∫1√logeydy
Now put logey=t.
∴ y=et
∴ dy=etdt
So, area A=21∫0√t etdt
|
Topper's Solved these Questions
- AREABook:CENGAGEChapter:AREAExercise:Exercise (Comprehension)
Explore 21 Videos
- AREABook:CENGAGEChapter:AREAExercise:Exercise (Matrix)
Explore 4 Videos
- AREABook:CENGAGEChapter:AREAExercise:Exercise (Single)
Explore 40 Videos
- APPLICATIONS OF DERIVATIVESBook:CENGAGEChapter:APPLICATIONS OF DERIVATIVESExercise:Question Bank
Explore 29 Videos
- AREA UNDER CURVESBook:CENGAGEChapter:AREA UNDER CURVESExercise:Question Bank
Explore 20 Videos
Similar Questions
Knowledge Check
The area of the region bounded by the curve y=x2 and the line y=16 is :
A323B2563C643D1283The area bounded by the curve y=2x−x2 and the line y = - x is
A32 sq unitsB93 sq unitsC92 sq unitsDNone of theseThe area of the region bounded by the curve y=x+1 and the lines x=2,x=3, is
A72sq unitsB92 sq unitC112 sq unitD132 sq units
Find the area of the region bounded by the curve y=x2and the line y=4.
View SolutionThe area of the region bounded by the curve y = x + 1 and the lines x=2,x=3, is
View SolutionArea of the region bounded by the curve y2= 4x , y-axis and the line y = 3 is (A) 2 (B) 94 (C) 93 (D) 92
View SolutionThe area of the region bounded by the curve y=x2−2 and line y = 2 , is equal to
View SolutionThe area of the plane region bounded by the curve x=y2−2 and the line y = - x is (in sq units)
View Solution