Let A(k) be the area bounded by the curves y=x2−3 and y=kx+2 The range of A(k) is (10√53,∞) The range of A(k) is (20√53,∞) If function k→A(k) is defined for k∈[−2,∞ ), then A(k) is many-one function. The value of k for which area is minimum is 1.
The range of A(k) is [10√53,∞)
The range of A(k) is [20√53,∞)
If function k→A(k) is defined for k∈[−2,∞), then A(k) is many-one function
The value of k for which area is minimum is 1
The correct Answer is:B, C
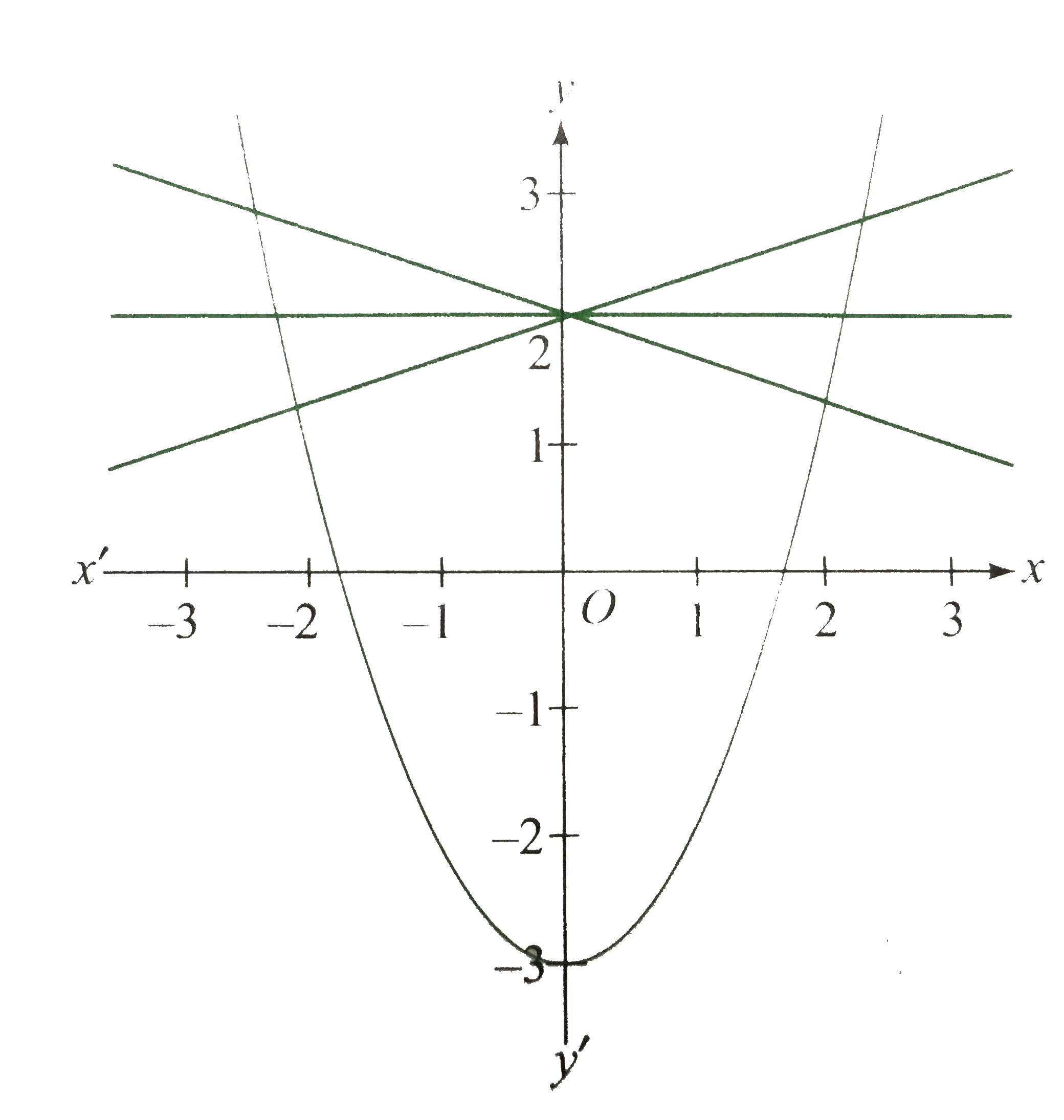
Line y=kx+2 passes through fixed point (0,2) for different value of k.
Also, it is obvious that minimum A(k) occurs when k=0, as when line is rotated from this position about point (0,2), the increased part of area is more than the decreased part of area. Therefore,
Minimum area =2√5∫0(2−(x2−3))dx
=2√5∫0(5−x2)dx
=2[5x−x33]√50
=2[5√5−5√53]
=20√53 sq. units
Topper's Solved these Questions
- AREABook:CENGAGEChapter:AREAExercise:Exercise (Comprehension)
Explore 21 Videos
- AREABook:CENGAGEChapter:AREAExercise:Exercise (Matrix)
Explore 4 Videos
- AREABook:CENGAGEChapter:AREAExercise:Exercise (Single)
Explore 40 Videos
- APPLICATIONS OF DERIVATIVESBook:CENGAGEChapter:APPLICATIONS OF DERIVATIVESExercise:Question Bank
Explore 29 Videos
- AREA UNDER CURVESBook:CENGAGEChapter:AREA UNDER CURVESExercise:Question Bank
Explore 20 Videos
Similar Questions
Let A(k) be the area bounded by the curves y=x2−3 and y=kx+2 The range of A(k) is (10√53,∞) The range of A(k) is (20√53,∞) If function k→A(k) is defined for k∈[−2,∞ ), then A(k) is many-one function. The value of k for which area is minimum is 1.
View SolutionLet A(k) be the area bounded by the curves y = x2−3 and y = kx + 2. If the range of A(k) is [p,∞), then find [ p], where [] represents the greatest integer function.
View Solution
Knowledge Check
Let A(k) be the area bounded by the curves y=x2+2x−3andy=kx+1. Then
Athe value of k for which A(k) is least is 2Bthe value of k for which A(k) is least is 3/2Cleast value of A(k) is 32/3Dleast value of A(k) is 64/3If the area bounded by the curves {(x,y)∣x2−y+1≥0}and{(x,y)∣x+y−3≥0} is k square units, then the value of 3k is equal to
A27B9C92D272The area bounded by the curve y=x2(x−1)2 with the x - axis is k sq. units. Then the value of 60 k is equal to
A1B2C12D14
If the area bounded by the curve y+x2=8x and the line y=12 is K sq. units, then the vlaue of 3K10 is
View SolutionThe area bounded by the curve x2=ky,k>0 and the line y=3 is 12unit2. Then k is (A) 3 (B) 3√3 (C) 34 (D) none of these
View SolutionThe area (in sq. units) of the region bounded by the curves y=2−x2andy=|x| is k, then the value of 3k is
View SolutionStatement I- The area of bounded by the curves y=x2−3 and y=kx+2 is least if k=0.
Statement II- The area bounded by the curves y=x2−3 and y=kx+2is√k2+20.
View SolutionWhat is the value of k if the area bounded by the curve y=sinkx,y=0,x=π/(3k) is 3 sq. unit?
View Solution