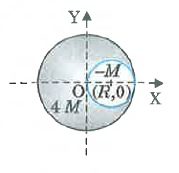
A disc of radis R is cut out from a larger disc of radius 2R in such a way that the edge of the hole touches the edge of the disc. Locate the centre of mass of the residual disc.

Video Solution

Text Solution

Verified by Experts

Mass can be assumed to be uniformly distributed proportional to area. Area of original dise is four times to that of the hole.
Let us select centre of disc as origin and X-axis along its line of symmetry as shown in figure. We can assume negative mass for the hole. Given state of system can be described as mass 4M kept at (0,0) and M at (R,0). We know that centre of mass lies on X-axis and coordinate can be written as follows:
$x = \frac{3 M \times 0 + (- M) \times R}{4 M - M} = \frac{R}{3}$
Hence centre of mass of the system lies at a distance R/3 towards left of its centre.

Updated on:21/07/2023

A disc of radius r is cut from a larger disc of radius 4r in such a way that the edge of the hole touches the edge of the disc. The centre of mass of the residual disc will be a distance from centre of larger disc :-
A $\frac{r}{5}$
B $\frac{r}{4}$
C $\frac{r}{2}$
D $\frac{r}{3}$
A circular hole is cut from a disc of radius 6 cm in such a way that the radius of the hole is 1 cm and the centre of 3 cm from the centre of the disc. The distance of the centre of mass of the remaining part from the centre of the original disc is
A3/35 cm
B1/35 cm
C3/10 cm
D7/35 cm
A circular disc of radius R is removed from a bigger circular disc of radius 2R such that the circumference of the discs coincoid . The centre of mass of the new disc is $α R$ from the centre of the bigger disc . the value of $α$ is
A $\frac{1}{3}$
B $\frac{1}{2}$
C $\frac{1}{6}$
D $\frac{1}{4}$