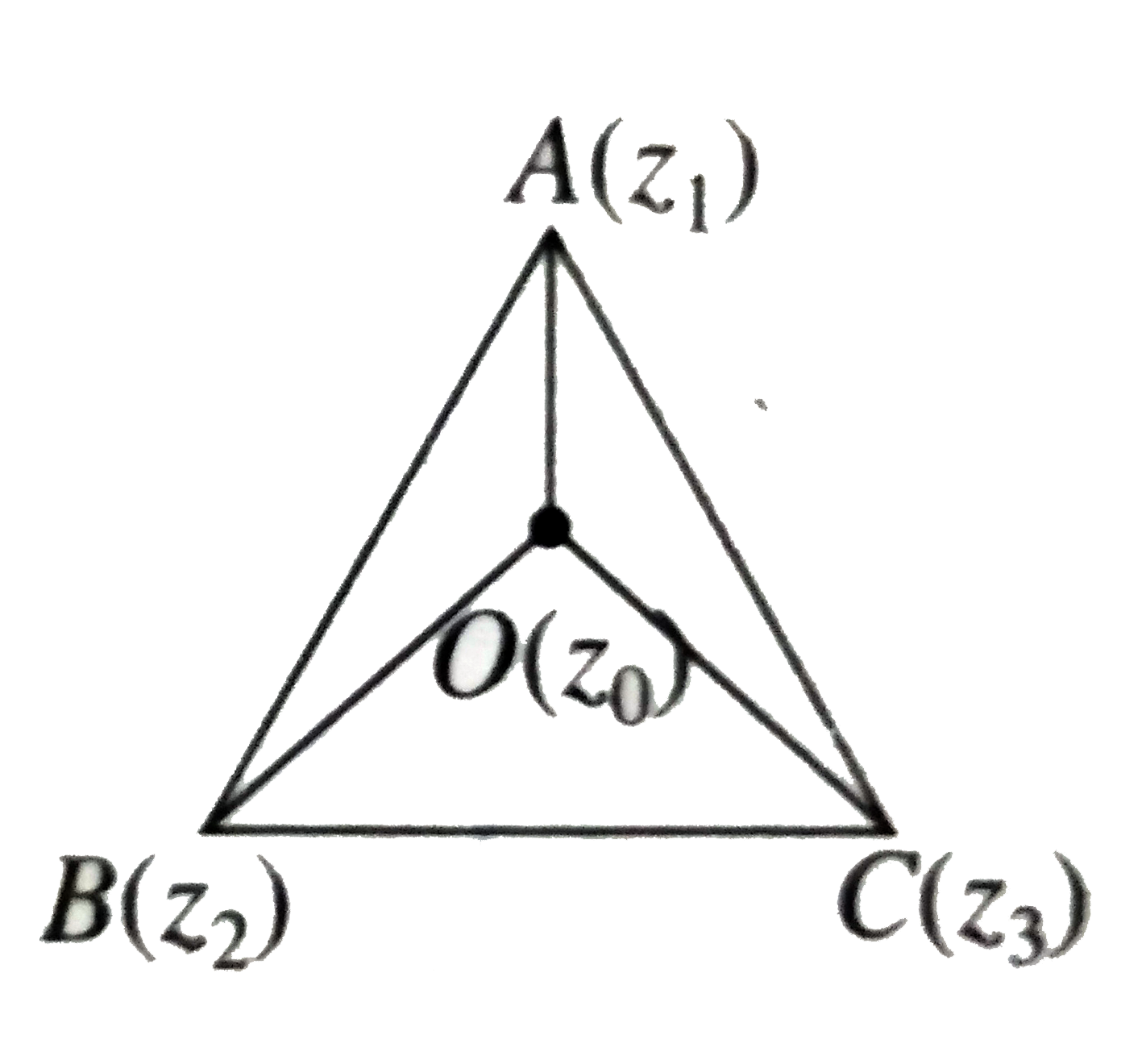
If z1, z2 and z3 are the vertices of ΔABC, which is not right angled triangle taken in anti-clock wise direction and z0 is the circumcentre, then (z0−z1z0−z2)sin2Asin2B+(z0−z3z0−z2)sin2Csin2B is equal to
0
1
−1
2
The correct Answer is:C
(c) Takin rotation at 'O' ltbr> z0−z1z0−z2=cos2C−isin2C
z0−z3z0−z2=cos2A+isin2A
Now (z0−z1z0−z2)sin2Asin2B+(z0−z3z0−z2)sin2Csin2B
=sin2Acos2C−isin2Asin2C
=+cos2Asin2C+isin2Asin2Csin2B
=sin(2A+2C)sin2B=−1
Topper's Solved these Questions
- COMPLEX NUMBERSBOOK - CENGAGECHAPTER - COMPLEX NUMBERSEXERCISE - Matching Column
1 Videos
- COMPLEX NUMBERSBOOK - CENGAGECHAPTER - COMPLEX NUMBERSEXERCISE - Comprehension
11 Videos
- COMPLEX NUMBERSBOOK - CENGAGECHAPTER - COMPLEX NUMBERSEXERCISE - Question Bank
30 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
32 Videos
- CONIC SECTIONSBOOK - CENGAGECHAPTER - CONIC SECTIONSEXERCISE - Solved Examples And Exercises
91 Videos
Similar Questions
If z1,z2,z3 are the vertices A,B,C of a right angled triangle taken in counte-clockwise direction with right angle at B and ACBC=√5 ,then (z3−z2)=
View SolutionIf z1,z2andz3 are the vertices of a right angledtriangle in Argand plane such that |z1−z2|=3,|z1−z3|=5andz2 is the vertex with the right angle then
View Solution
Knowledge Check
If z1,z2andz3 be the vertices of ΔABC, taken in anti-clock wise direction and z0 be the circumstance, then (z0−z1z0−z2)sin2Asin2B+(z0−z3z0−z2)sin2Csin2B is equal to
A0B1C−1D2If z1,z2,z3 are the vertices of an isoscles triangle right angled at z2, then
Az21+2z22+z23=2z2(z1+z3)Bz21+z22+z23=2z2(z1+z3)Cz21+z22+2z23=2z2(z1+z3)D2z21+z22+z23=2z2(z1+z3)The tangents at z1,z2 on the circle |z−z0|=a, meet at z3. Then (z3−z1z0−z1)(z0−z2z3−z2) is equal to
A0B1C−1D2
If |z1−z0|=|z2−z0|=a and amp(z2−z0z0−z1)=π2 , then find z0
View SolutionComplex numbers of z1,z2,z3 are the vertices A, B, C respectively, of on isosceles right-angled triangle with right angle at C. show that (z1−z2)2 = 2(z1−z3)(z3−z2)
View Solutionz1,z2,z3 are the vertices of an equilateral triangle taken in counter clockwise direction. If its circumcenter is at (1−2i) and (z1=2+i), then z2=
View SolutionIf the tangents at z1, z2 on the circle |z−z0|=r intersect at z3, then (z3−z1)(z0−z2)(z0−z1)(z3−z2) equals
View SolutionIf z1,z2andz3 represent the vertices of an equilateral triangle such that |z1|=|z2|=|z3|, then
View Solution
