A particle moves in a circular path such that its speed v varies with distance s as v=α√s where α is a positive constant. If the acceleration of the particle after traversing a distance s is [α2√x+s2R2] find x.
α2√14−s2R2
α2√14+s2R2
α√12+s2R2
α2√12+s2R2
The correct Answer is:B
a=√a2t+a2t=√(dvdt)2+(v2R)2
Similar Questions
The speed (v) of a particle moving in a circle of radius R varies with distance s as v=ks where k is a positive constant.Calculate the total acceleration of the particle
View SolutionA particle of mass m moves in a circle of radius R in such a way that its speed (v) varies with distance (s) as v=a√s where a is a constant. Calcualte the acceleration and force on the particle.
View Solution
Knowledge Check
A particle moves in a circular path such that its speed v varies with distance s as v=α√s, where α is a positive constant. Find the acceleration of the particle after traversing a distance s.
Aα√14+s2R2Bα2√14+sRCα√14+sRDα2√14+s2R2The v−s and v2−s graph are given for two particles. Find the accelerations of the particles at s=0.
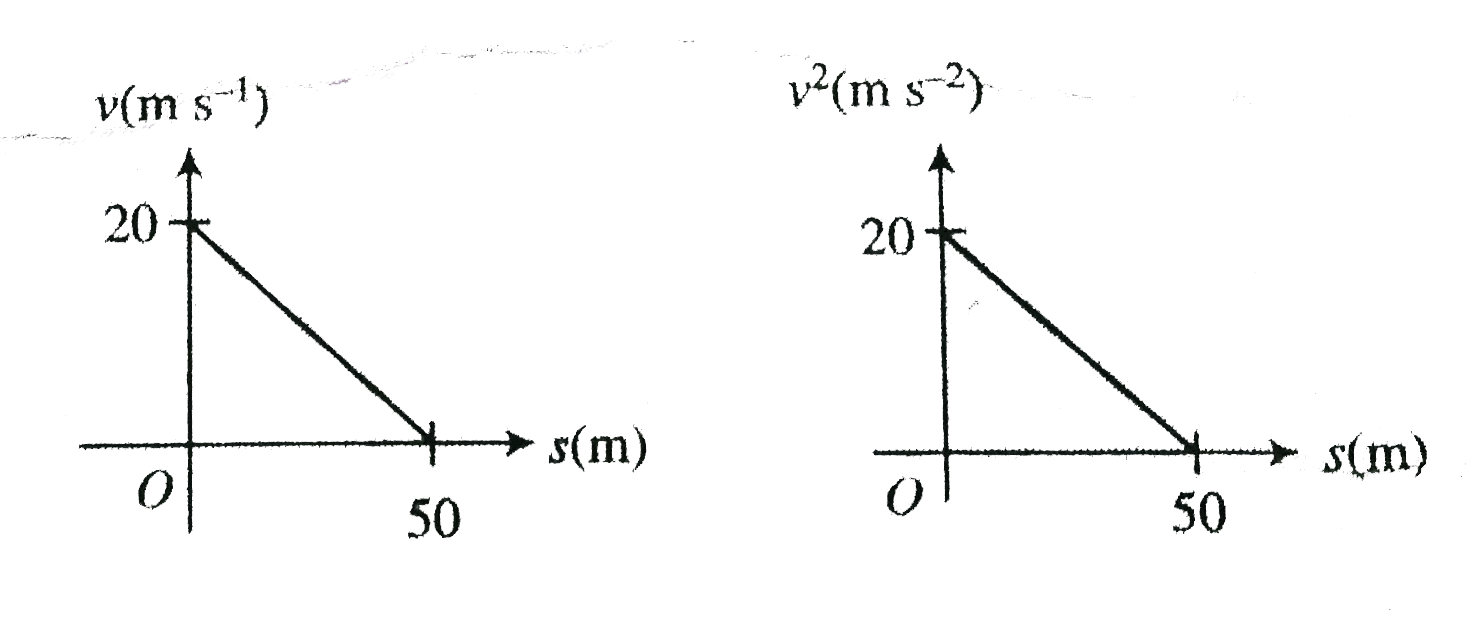 .A-8, -0.2B9, 0.2C8, 0.3D9, 0.3
.A-8, -0.2B9, 0.2C8, 0.3D9, 0.3
A particle is moving on a circular path with speed given by v= αt, where α is a constant. Find the acceleration of the particle at th instant when it has covered the nth fraction of the . Circle.
View SolutionA particle moves uniformly with speed v along a parabolic path y=kx2, where k is a positive constant. Find the acceleration of the particle at the point x = 0.
View SolutionThekinetic energyofaparticle movingalong a circle of radius R depends on the distance covered S as K =αS2, where α is a constant. Find theaorce acting on the particle as a function of S.
View SolutionA particle of unit mass is moving along x-axis. The velocity of particle varies with position x as v(x). =αx−β (where α and β are positive constants and x>0 ). The acceleration of the particle as a function of x is given as
View SolutionA particle is moving in a straight line and its velocity varies with its displacement as v=√4+4s m/s. Assume s=0 at t=0. find the displacement of the particle in metres at t=1s.
View SolutionA particle moving along the circular path with a speed v and its speed increases by g in one second.If the radius of the circular path be r ,then the net acceleration of the particle is?
View Solution
