A particle moves in a circular path such that its speed v varies with distance s as v=α√s, where α is a positive constant. Find the acceleration of the particle after traversing a distance s.
α√14+s2R2
α2√14+sR
α√14+sR
α2√14+s2R2
The correct Answer is:D
To find the acceleration of a particle moving in a circular path with a speed that varies with distance, we can follow these steps:
1. Understand the relationship between speed and distance: We are given that the speed v of the particle varies with distance s as:
v=α√s
where α is a positive constant.
2. Differentiate the speed with respect to time: To find the acceleration, we need to differentiate v with respect to time t. Using the chain rule, we have:
dvdt=dvds⋅dsdt
3. Calculate dvds: First, we differentiate v with respect to s:
dvds=dds(α√s)=α⋅12√s=α2√s
4. Substitute dsdt: We know that dsdt=v=α√s. Now substituting this into the equation for dvdt:
dvdt=α2√s⋅(α√s)=α22
5. Calculate the radial acceleration Ar: The radial acceleration is given by:
Ar=v2R
Substituting v=α√s:
Ar=(α√s)2R=α2sR
6. Calculate the total acceleration A: The total acceleration A is the vector sum of the tangential acceleration At and the radial acceleration Ar:
A=√A2t+A2r
Substituting At=α22 and Ar=α2sR:
A=√(α22)2+(α2sR)2
7. Factor out common terms: We can factor out α2:
A=α√α44+α4s2R2=α2√14+s2R2
Final Result:
Thus, the acceleration of the particle after traversing a distance s is:
A=α2√14+s2R2
v=α√s
where α is a positive constant.
dvdt=dvds⋅dsdt
dvds=dds(α√s)=α⋅12√s=α2√s
dvdt=α2√s⋅(α√s)=α22
Ar=v2R
Substituting v=α√s:
Ar=(α√s)2R=α2sR
A=√A2t+A2r
Substituting At=α22 and Ar=α2sR:
A=√(α22)2+(α2sR)2
A=α√α44+α4s2R2=α2√14+s2R2
Thus, the acceleration of the particle after traversing a distance s is:
A=α2√14+s2R2
Topper's Solved these Questions
- KINEMATICS-2BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Solved Examples
7 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise 5.1
15 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression
2 Videos
Similar Questions
A particle moves in a circular path such that its speed v varies with distance s as v=α√s where α is a positive constant. If the acceleration of the particle after traversing a distance s is [α2√x+s2R2] find x.
View SolutionA particle of mass m moves in a circle of radius R in such a way that its speed (v) varies with distance (s) as v=a√s where a is a constant. Calcualte the acceleration and force on the particle.
View Solution
Knowledge Check
A particle moves in a circular path such that its speed v varies with distance s as v=α√s, where α is a positive constant. Find the acceleration of the particle after traversing a distance s.
Aα√14+s2R2Bα2√14+sRCα√14+sRDα2√14+s2R2A particle having a velocity v=v0 at t=0 is decelerated at the rate |a|=α√v, where α is a positive constant.
AThe particle comes to rest at t=2√v0αBThe particle will come to rest at infinityCThe distance travelled by the particle before coming to rest is 2v3/20αDThe distance travelled by the particle before coming to rest is 2v3/203αThe v−s and v2−s graph are given for two particles. Find the accelerations of the particles at s=0.
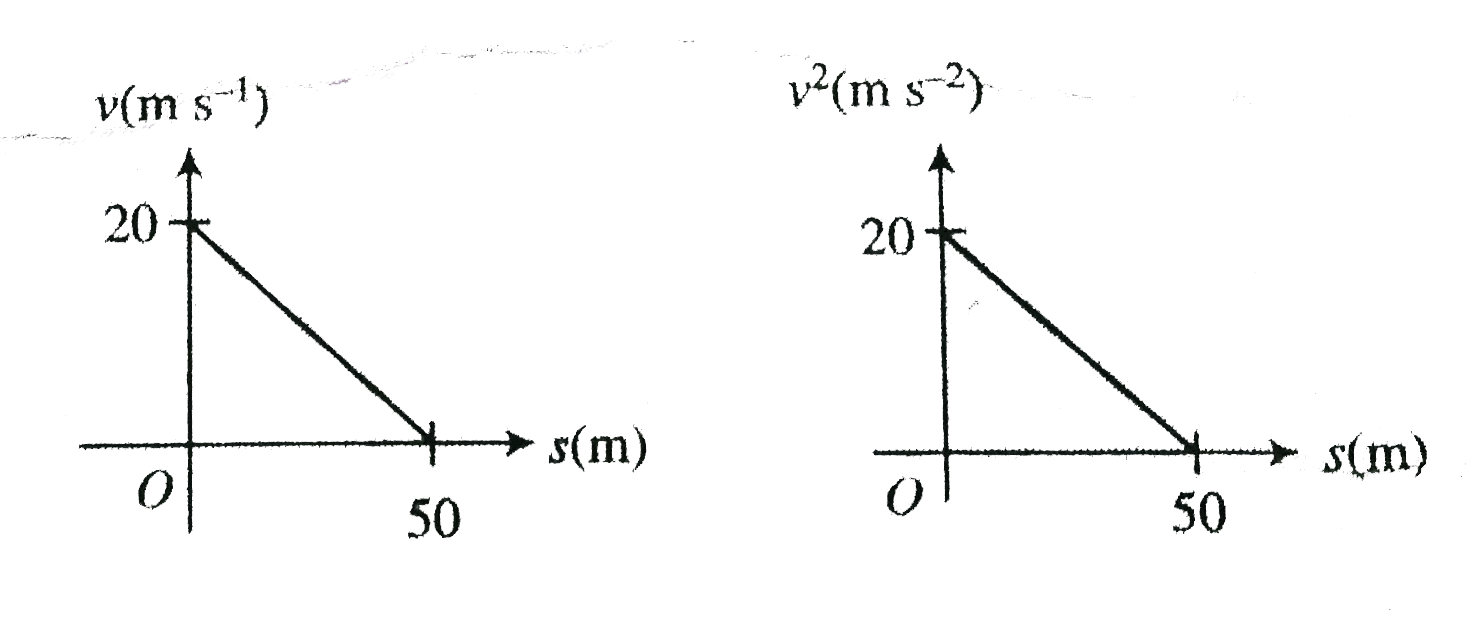 .A-8, -0.2B9, 0.2C8, 0.3D9, 0.3
.A-8, -0.2B9, 0.2C8, 0.3D9, 0.3
The speed (v) of a particle moving in a circle of radius R varies with distance s as v=ks where k is a positive constant.Calculate the total acceleration of the particle
View SolutionA particle is moving on a circular path with speed given by v= αt, where α is a constant. Find the acceleration of the particle at th instant when it has covered the nth fraction of the . Circle.
View SolutionA particle moves uniformly with speed v along a parabolic path y=kx2, where k is a positive constant. Find the acceleration of the particle at the point x = 0.
View SolutionA particle of unit mass is moving along x-axis. The velocity of particle varies with position x as v(x). =αx−β (where α and β are positive constants and x>0 ). The acceleration of the particle as a function of x is given as
View SolutionThekinetic energyofaparticle movingalong a circle of radius R depends on the distance covered S as K =αS2, where α is a constant. Find theaorce acting on the particle as a function of S.
View Solution
