A particle moves in a circular path such that its speed v varies with distance s as v=α√s where α is a positive constant. If the acceleration of the particle after traversing a distance s is [α2√x+s2R2] find x.
The correct Answer is:32
To solve the problem, we need to analyze the motion of a particle moving in a circular path with a varying speed. The speed v of the particle is given by the equation:
v=α√s
where α is a positive constant and s is the distance traveled along the circular path. We are also given that the acceleration of the particle after traversing a distance s is:
a=α2√x+s2R2
where R is the radius of the circular path. We need to find the value of x.
Step 1: Identify the components of acceleration
The total acceleration a of the particle can be broken down into two components:
1. Radial acceleration aR (centripetal acceleration) which is directed towards the center of the circular path.
2. Tangential acceleration aT which is due to the change in speed along the path.
The radial acceleration is given by:
aR=v2R
And the tangential acceleration can be found using the chain rule:
aT=dvdt=dvds⋅dsdt=dvds⋅v
Step 2: Calculate dvds
From the expression for v:
v=α√s
Taking the derivative with respect to s:
dvds=α2√s
Step 3: Calculate tangential acceleration aT
Now substituting dvds and v into the expression for aT:
aT=α2√s⋅(α√s)=α22
Step 4: Substitute into total acceleration
Now we can express the total acceleration a as the resultant of aR and aT:
a=√a2R+a2T
Substituting aR and aT:
aR=(α√s)2R=α2sR
Thus,
a=√(α2sR)2+(α22)2
Step 5: Simplify the expression
Now we simplify the expression:
a=√α4s2R2+α44=α2√s2R2+14
Step 6: Compare with given acceleration
We know from the problem statement that:
a=α2√x+s2R2
Equating the two expressions for acceleration:
α2√s2R2+14=α2√x+s2R2
Step 7: Square both sides
Squaring both sides gives:
s2R2+14=x+s2R2
Step 8: Solve for x
Subtracting s2R2 from both sides:
14=x
Thus, we find:
x=14
Final Answer:
The value of x is:
14
The total acceleration a of the particle can be broken down into two components:
1. Radial acceleration aR (centripetal acceleration) which is directed towards the center of the circular path.
2. Tangential acceleration aT which is due to the change in speed along the path.
From the expression for v:
Now substituting dvds and v into the expression for aT:
Now we can express the total acceleration a as the resultant of aR and aT:
Now we simplify the expression:
We know from the problem statement that:
Squaring both sides gives:
Subtracting s2R2 from both sides:
The value of x is:
Similar Questions
The speed (v) of a particle moving in a circle of radius R varies with distance s as v=ks where k is a positive constant.Calculate the total acceleration of the particle
View SolutionA particle of mass m moves in a circle of radius R in such a way that its speed (v) varies with distance (s) as v=a√s where a is a constant. Calcualte the acceleration and force on the particle.
View Solution
Knowledge Check
A particle moves in a circular path such that its speed v varies with distance s as v=α√s, where α is a positive constant. Find the acceleration of the particle after traversing a distance s.
Aα√14+s2R2Bα2√14+sRCα√14+sRDα2√14+s2R2The v−s and v2−s graph are given for two particles. Find the accelerations of the particles at s=0.
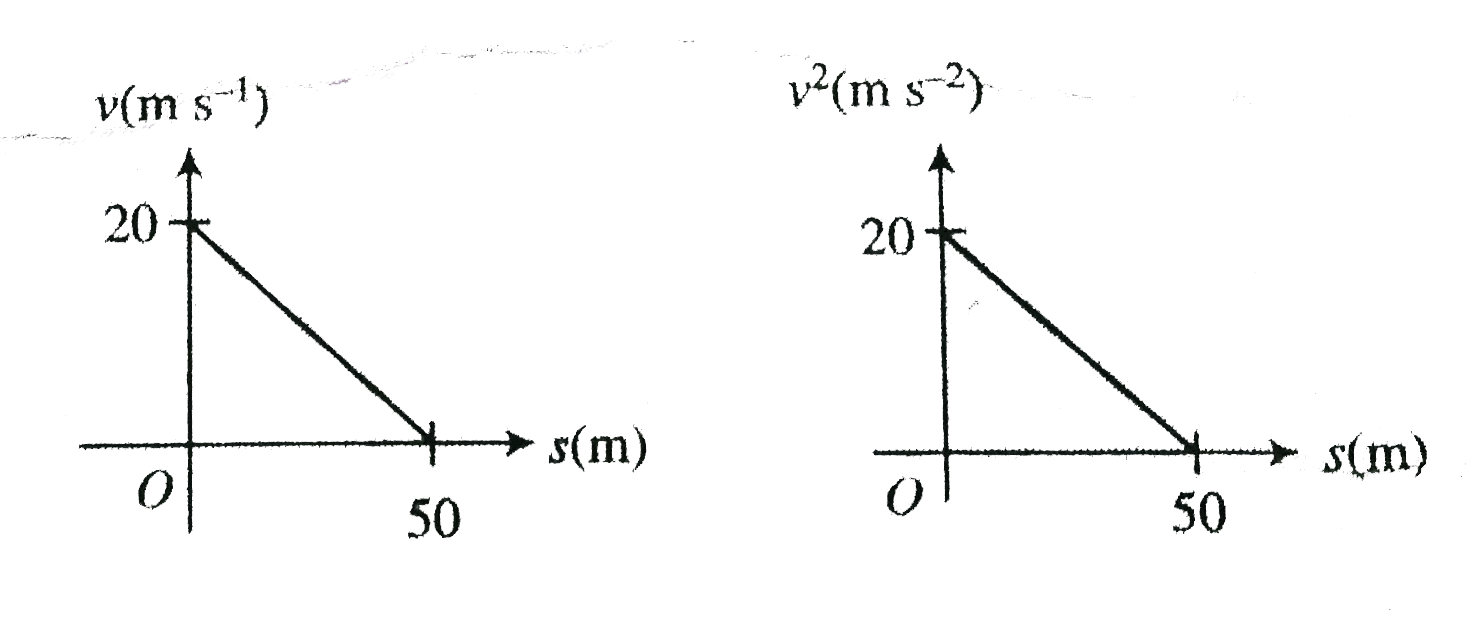 .A-8, -0.2B9, 0.2C8, 0.3D9, 0.3
.A-8, -0.2B9, 0.2C8, 0.3D9, 0.3
A particle is moving on a circular path with speed given by v= αt, where α is a constant. Find the acceleration of the particle at th instant when it has covered the nth fraction of the . Circle.
View SolutionA particle moves uniformly with speed v along a parabolic path y=kx2, where k is a positive constant. Find the acceleration of the particle at the point x = 0.
View SolutionThekinetic energyofaparticle movingalong a circle of radius R depends on the distance covered S as K =αS2, where α is a constant. Find theaorce acting on the particle as a function of S.
View SolutionA particle of unit mass is moving along x-axis. The velocity of particle varies with position x as v(x). =αx−β (where α and β are positive constants and x>0 ). The acceleration of the particle as a function of x is given as
View SolutionA particle is moving in a straight line and its velocity varies with its displacement as v=√4+4s m/s. Assume s=0 at t=0. find the displacement of the particle in metres at t=1s.
View SolutionA particle moving along the circular path with a speed v and its speed increases by g in one second.If the radius of the circular path be r ,then the net acceleration of the particle is?
View Solution

