A force is applied on a door at point P making an angle θ with position vector ¯r , by partical observation , give the value of theta for which on has to exert minimum force to rotate the door ?
00
π
π2
π3
The correct Answer is:C
To find the angle θ at which the minimum force is required to rotate the door, we can analyze the torque produced by the applied force. Here’s the step-by-step solution:
Step 1: Understand the Torque Concept
Torque (τ) is the measure of the rotational force applied to an object. It is given by the formula:
τ=r⋅F⋅sin(θ)
where:
- r is the distance from the pivot point (hinge) to the point of force application,
- F is the magnitude of the applied force,
- θ is the angle between the position vector →r and the force vector →F.
Step 2: Identify Components of Force
When a force F is applied at an angle θ to the position vector →r, it can be resolved into two components:
- The component along the direction of →r: Fcos(θ)
- The component perpendicular to →r: Fsin(θ)
Step 3: Determine the Effective Torque
The effective torque that causes the door to rotate is produced by the perpendicular component of the force:
τ=r⋅(Fsin(θ))
To open the door easily, we need to maximize this torque.
Step 4: Maximize the Torque
To maximize the torque, we need to maximize the sin(θ) term. The sine function reaches its maximum value of 1 when:
θ=90∘(or π2 radians)
Step 5: Conclusion
Thus, the angle θ for which one has to exert minimum force to rotate the door is:
θ=90∘
Torque (τ) is the measure of the rotational force applied to an object. It is given by the formula:
τ=r⋅F⋅sin(θ)
where:
- r is the distance from the pivot point (hinge) to the point of force application,
- F is the magnitude of the applied force,
- θ is the angle between the position vector →r and the force vector →F.
When a force F is applied at an angle θ to the position vector →r, it can be resolved into two components:
- The component along the direction of →r: Fcos(θ)
- The component perpendicular to →r: Fsin(θ)
The effective torque that causes the door to rotate is produced by the perpendicular component of the force:
τ=r⋅(Fsin(θ))
To open the door easily, we need to maximize this torque.
To maximize the torque, we need to maximize the sin(θ) term. The sine function reaches its maximum value of 1 when:
θ=90∘(or π2 radians)
Thus, the angle θ for which one has to exert minimum force to rotate the door is:
θ=90∘
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EXERCISE - II (C.W)
60 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EXERCISE - II (H.W)
60 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EXERCISE - I (C.W)
63 Videos
- SYSTEM OF PARTICLESBOOK - NARAYNACHAPTER - SYSTEM OF PARTICLESEXERCISE - Level-VI
78 Videos
- THERMAL PROPERTIES OF MATTERBOOK - NARAYNACHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - LEVEL - II (H.W.)
19 Videos
Similar Questions
If O be the origin and OP=r and OP makes an angle θ with the positive direction of x-axis and lies in the XY plane find the coordinates of P.
View SolutionA bar of cross section A is subjected to equal and opposite tensile forces F at its ends. Consider a plane through the bar making an angle θ with a plane at right angles to the bar as shown in figure.
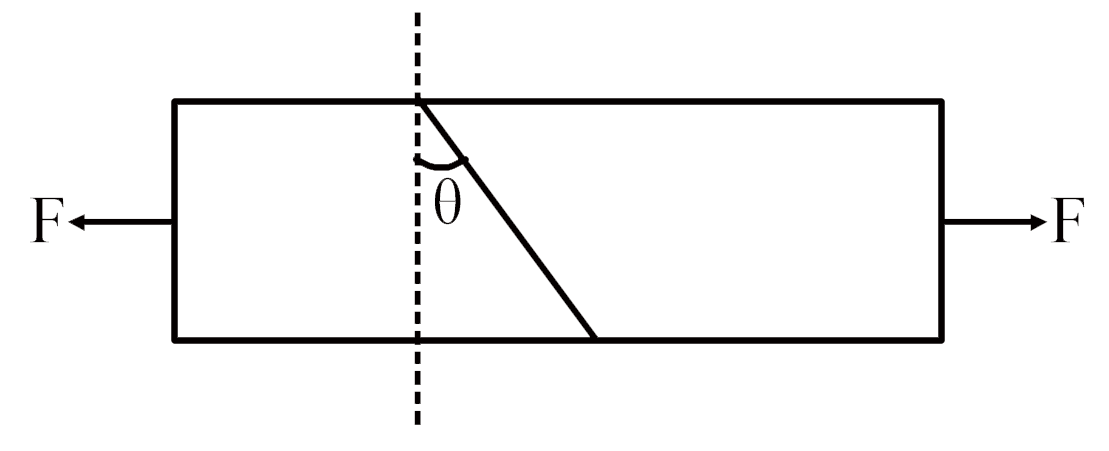
In the above problem, for what value of θ is the tensile stress maximum ?
View Solution
Knowledge Check
A pulling force making an angle θ with the horizontal is applied on a block of weight W placed on a horizontal table. If the angle of friction is ϕ, the magnitude of the force required to move the body is equal to
AWCosϕCos(θ−ϕ)BWsinϕCos(θ−ϕ)CWtanϕSin(θ−ϕ)DWSinϕTan(θ−ϕ)Pushing force making an angle θ to the horizontal is applied on a block of weight W placed on a horizontal table . If the angle of friction is ϕ , magnitude of force required to move the body is equal to :
AWcosϕ/cos(θ−ϕ)BWsinϕ/cos(θ+ϕ)CWtanϕ/sin(θ−ϕ)DWsinθ/g tan(θ−ϕ)A block of mass M is pulled on a smooth horizontal table by a string making an angle θ with the horizontal as shown in figure. If the acceleration of the block is a find the force applied by the string and by the table N on the block.
 AT=Matanθ , N=(Mg−Matanθ)BT=Mgcosθ , N=(Mg−Matanθ)CT=Macosθ , N=(Mg−Macosθ)DT=Macosθ , N=(Mg−Matanθ)
AT=Matanθ , N=(Mg−Matanθ)BT=Mgcosθ , N=(Mg−Matanθ)CT=Macosθ , N=(Mg−Macosθ)DT=Macosθ , N=(Mg−Matanθ)
The resultant of two forces 3P and 2P is R. If the first force is doubled then resultant is also doubled.The angle between the two forces is
View SolutionA tangential force F is applied on a disc of radius R, due to which it deflects through an angle θ from its initial position. The work done by this force would be
View SolutionAn inclined plane is inclined at an angle θ with the horizontal. A body of mass m rests on it, if the coefficient of friction is μ, then the minimum force that has to be applied to the inclined plane to make the body just move up the inclined plane is
View SolutionPulling force making an angle θ to the horizontal is applied on a block of weight W placed on a horizontal table. If the angle of friction is α, then the magnitude of force is α , then the magnitude of force required to move the body is equal to
View SolutionTwo forces of equal magnitude act at a point making an angle θ with each other. If the direction of one of the forces is reversed, the direction of the resultant will turn through
View Solution
