A heavy disc is thrown on a horizontal surface in such a way that it slides with a speed V0 intially without rollind . It will start rolling without slipping when its speed is reduced
13V0
23V0
35V0
57V0
The correct Answer is:B
To solve the problem, we need to analyze the motion of a heavy disc that is initially sliding on a horizontal surface without rolling. The disc will eventually start rolling without slipping due to the frictional force acting on it. We will derive the final speed at which the disc starts rolling without slipping.
1. Initial Conditions:
- The disc is thrown with an initial speed V0 and has no initial angular velocity (i.e., it is not rolling).
- The disc has mass m and radius r.
2. Frictional Force:
- Since the surface is rough, a frictional force will act on the disc. This force will oppose the motion of the disc, reducing its linear speed and increasing its angular speed until it starts rolling without slipping.
3. Angular Momentum Conservation:
- We will analyze the angular momentum of the disc about its center of mass.
- Initially, the angular momentum Li of the disc can be calculated as:
Li=m⋅V0⋅r
- This is because the entire mass can be considered to be concentrated at a distance r from the axis of rotation.
4. Final Angular Momentum:
- When the disc starts rolling without slipping, it has both translational and rotational motion.
- The final angular momentum Lf can be expressed as:
Lf=m⋅V⋅r+I⋅ω
- Here, I is the moment of inertia of the disc, which is given by I=12mr2, and ω (angular velocity) can be related to the linear velocity V by:
ω=Vr
- Substituting these into the equation for Lf:
Lf=m⋅V⋅r+(12mr2)⋅(Vr)=m⋅V⋅r+12mVr
- Simplifying this gives:
Lf=m⋅V⋅r+12mVr=32mVr
5. Setting Initial and Final Angular Momentum Equal:
- By the conservation of angular momentum, we set Li=Lf:
m⋅V0⋅r=32mV⋅r
- Dividing both sides by m⋅r (assuming m and r are not zero):
V0=32V
6. Solving for Final Velocity V:
- Rearranging the equation gives:
V=23V0
Final Answer:
The disc will start rolling without slipping when its speed is reduced to:
V=23V0
- The disc is thrown with an initial speed V0 and has no initial angular velocity (i.e., it is not rolling).
- The disc has mass m and radius r.
- Since the surface is rough, a frictional force will act on the disc. This force will oppose the motion of the disc, reducing its linear speed and increasing its angular speed until it starts rolling without slipping.
- We will analyze the angular momentum of the disc about its center of mass.
- Initially, the angular momentum Li of the disc can be calculated as:
Li=m⋅V0⋅r
- This is because the entire mass can be considered to be concentrated at a distance r from the axis of rotation.
- When the disc starts rolling without slipping, it has both translational and rotational motion.
- The final angular momentum Lf can be expressed as:
Lf=m⋅V⋅r+I⋅ω
- Here, I is the moment of inertia of the disc, which is given by I=12mr2, and ω (angular velocity) can be related to the linear velocity V by:
ω=Vr
- Substituting these into the equation for Lf:
Lf=m⋅V⋅r+(12mr2)⋅(Vr)=m⋅V⋅r+12mVr
- Simplifying this gives:
Lf=m⋅V⋅r+12mVr=32mVr
- By the conservation of angular momentum, we set Li=Lf:
m⋅V0⋅r=32mV⋅r
- Dividing both sides by m⋅r (assuming m and r are not zero):
V0=32V
- Rearranging the equation gives:
V=23V0
The disc will start rolling without slipping when its speed is reduced to:
V=23V0
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - C.U.Q
71 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EXERCISE - I (C.W)
63 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EVALUATE YOURSELF - 3
4 Videos
- SYSTEM OF PARTICLESBOOK - NARAYNACHAPTER - SYSTEM OF PARTICLESEXERCISE - Level-VI
78 Videos
- THERMAL PROPERTIES OF MATTERBOOK - NARAYNACHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - LEVEL - II (H.W.)
19 Videos
Similar Questions
A ball (solid sphere) is thrown down the valley in such a way that it slides with a speed v0 initially without rolling. Prove that it will roll without any sliding when its speed falls to (57)v0. The transition from pure sliding to pure rolling is gradual, so that both sliding and rolling take place during this interval.
View SolutionA ball is thrown down a lawn in such a way that it initially slides with a speed v0 without rolling. It gradually picks up rotational motion. Prove that it will be without sliding, that is, its motion will be pure rolling when its speed falls to 57v0.
View Solution
Knowledge Check
A uniform disc of mass m and radius R is thrown on horizontal law in such a way that it initially slides with speed V0 without rolling. The distance travelled by the disc till it starts pure rolling is (Coefficient friction between the contact is 0.5)
Av209gB5v209gCv203gD3v208gA heavy solid sphere is thrown on a horizontal rough surface with initial velocity u without rolling. What will be its speed, when it starts pure rolling motion ?
A3u5B2u5C5u7D2u7A ball is thrown down a lawn in such a way that it initially slides with a speed v0 without rolling. It gradually picks up rotational motion. Find the speed of the ball at which there will be rolling without slipping :
A27v0B25v0C57v0D35v0
A sphereicla ball of mass M and radius R is projected along a rough horizontal surface so that initially (t=0) it slides with a linear speed v0 but does not rotate. As it slides, it begins to spin and eventually rolls without slipping. How long does it take to begin rolling without slipping?
View SolutionA disc rolls without slipping on a horizontal surface such that its velocity of center of the mass is v. Find the velocity of points A,B,C and D.
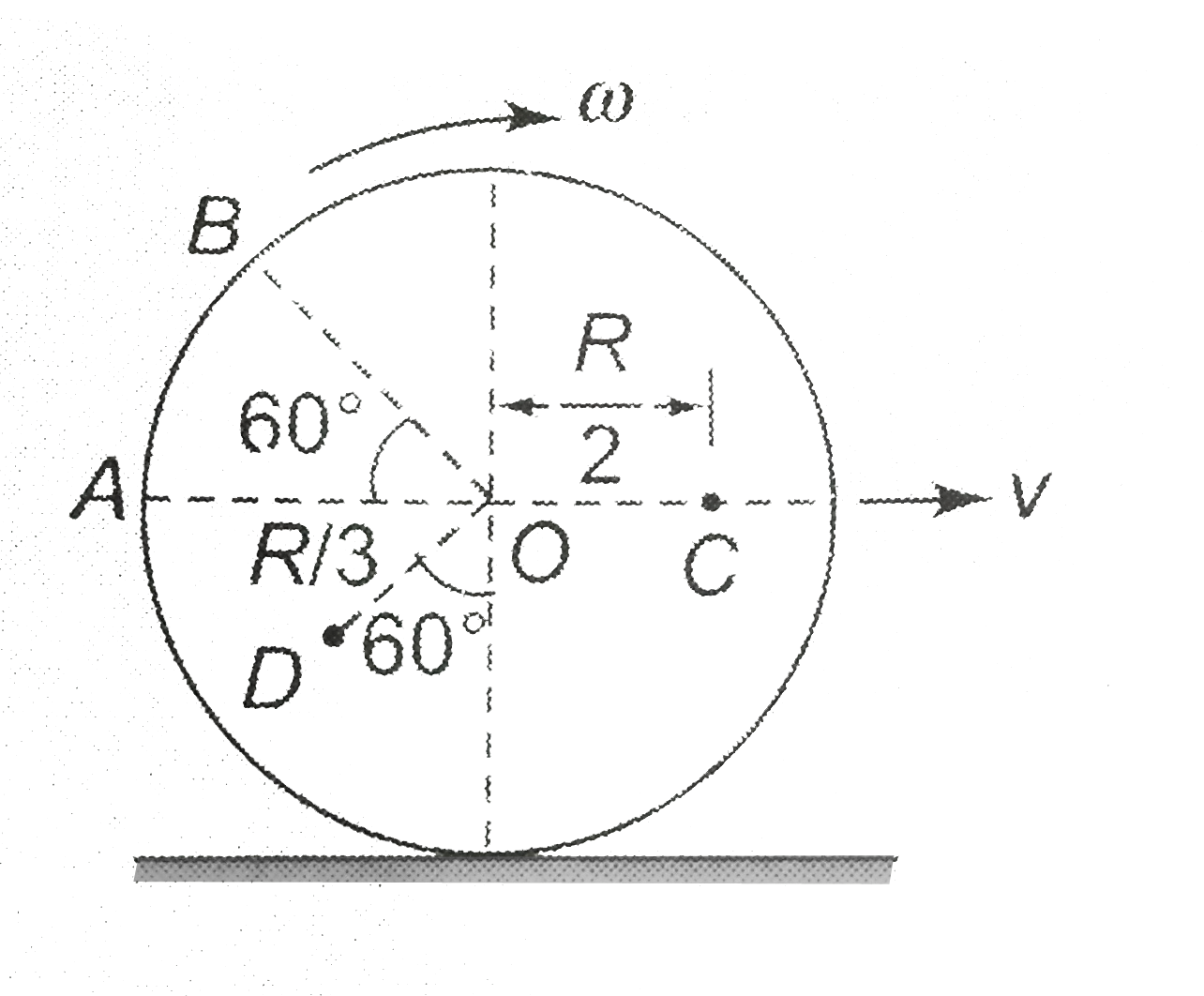
View SolutionA solid sphere is thrown on a horizontal rough surface with initial velocity of centre of mass V0 without rolling. Velocity of its centre of mass when its starts pure rolling is
View SolutionA thin spherical shell lying on a rough horizontal surface is hit by as cue in such a way that the line of action passes through the centre of the shell. As a result the shell starts moving with a linear speed v without any initial angualr velocity. Find the linear speed of the shell after it starts pure rolling on the surface.
View SolutionA solid uniform sphere resting on a rough horizontal plane is given a horizontal impulse directed through its centre so that it starts sliding with an initial velocity v0. When it finally starts rolling without slipping the speed of its centre is
View Solution
