In Fig. 10.36, A,B and C are three points on a circle with centre O such that and . If D is a point on the circle other than the arc ABC, find
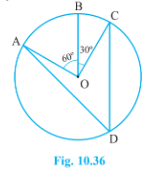
The angle subtended by an arc at the center is double the angle subtended by it at any point on the remaining part of the circle.
(By Theorem 10.8)
Topper's Solved these Questions
- CIRCLESBOOK - NCERTCHAPTER - CIRCLESEXERCISE - EXERCISE 10.2
2 Videos
- CIRCLESBOOK - NCERTCHAPTER - CIRCLESEXERCISE - Exercise 10.4
6 Videos
- AREAS OF PARALLELOGRAMS AND TRIANGLESBOOK - NCERTCHAPTER - AREAS OF PARALLELOGRAMS AND TRIANGLESEXERCISE - EXERCISE 9.1
1 Videos
- CONSTRUCTIONSBOOK - NCERTCHAPTER - CONSTRUCTIONSEXERCISE - EXERCISE 11.2
5 Videos
Similar Questions
Knowledge Check
A, B and C are three points on a circle with centre O. The tangent at C meets BA produced to T. If ∠ATC=30∘ and ∠ACT=48∘, then what is the value of ∠AOB?
A78∘B96∘C102∘D108∘
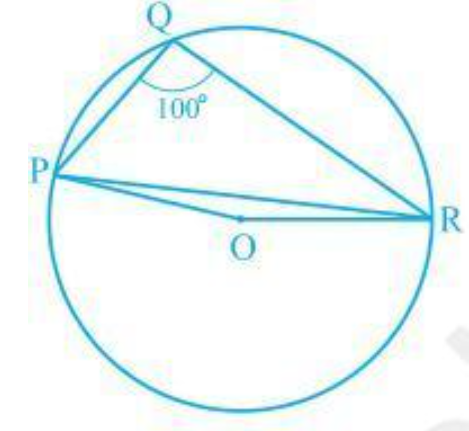
In Fig. 10.37, ∠PQR = 100∘, where P, Q and R are points on a circle with centre O. Find ∠OPR.
View SolutionIn Fig. PQ is tangent at a point R of the circle with centre O . If ∠TRQ=30o , find ∠PRS . (FIGURE)
View SolutionIn Fig. 10.96, if TP and TQ are tangents drawn from an external points T to a circle with centre O such that ∠TQP=60o , then ∠OPQ= (FIGURE) 25o (b) 30o (c) 40o (d) 60o
View SolutionIf AB, BC and CD are equal chords of a circle with O as centre and AD diameter, then ∠AOB= 600 (b) 900 (c) 1200 (d) none of these
View SolutionIn Fig. 10.101, PQ and PR are two tangents to a circle with centre O . If ∠QPR=46o , then ∠QOR equals (FIGURE) 67o (b) 134o (c) 44o (d) 46o
View SolutionIf A,B,C are three points on a circle with centre O such that ∠AOB=90∘ and ∠BOC=120∘, then ∠ABC= 60∘ (b) 75∘ (c) 90∘ (d) 135∘
View SolutionThe diagonals of a rectangle ABCD meet at O . If ∠BOC=44∘, find ∠OAD
View Solution