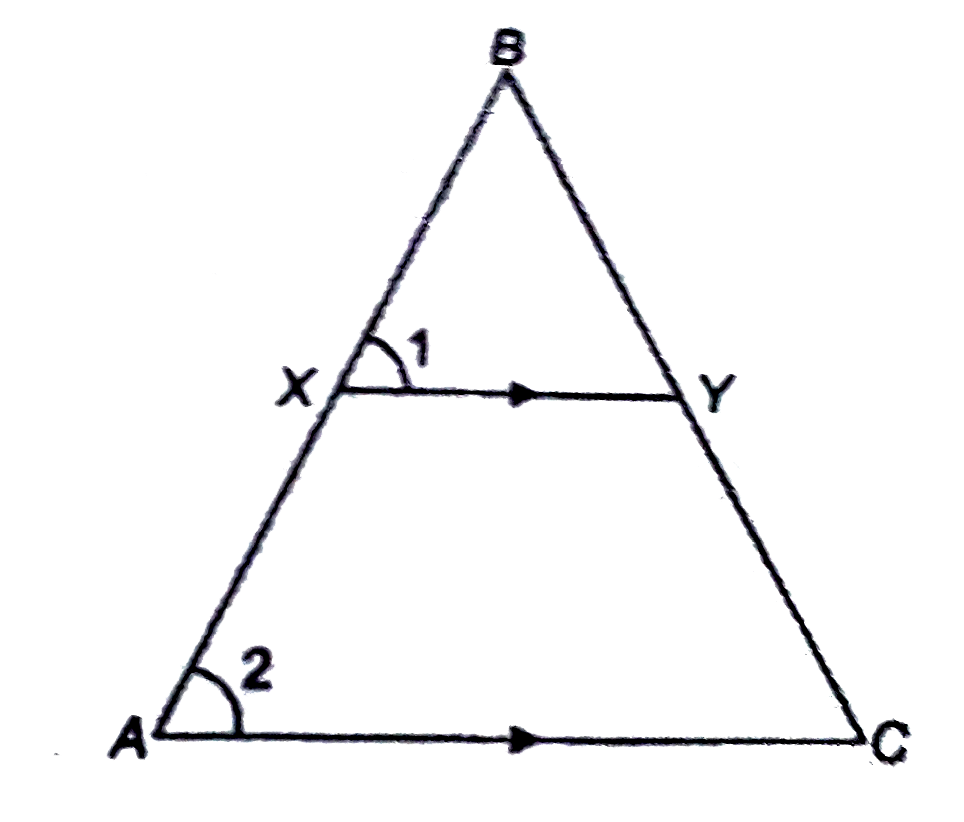
X and Y are points on the sides AB and BC respectively of $△ A B C$ such that XY||AC and XY divides $△ A B C$ into two parts in area , find $\frac{A X}{A B}$

Video Solution

Text Solution

Verified by Experts

Since XY||AC
In $△ B X Y and △ B A C$ .
$∠ 1 = ∠ 2$ (corresponding angles)
$∠ B = ∠ B$ (common)
$△ B X Y ~ △ B A C$ (AA corollary)
$\frac{a r (△ B X Y)}{a r (△ B A C)} = \frac{B X^{2}}{B A^{2}}$ (area ratio theorem) ....(1)
But $a r (△ B X Y) = \frac{1}{2} a r (△ A B C)$ (given)
$\Rightarrow \frac{a r (△ B X Y)}{a r (△ A B C)} = \frac{1}{2}$
from (1) and (2) we get
$\frac{B X^{2}}{A B^{2}} = \frac{1}{2} \Rightarrow \frac{B X}{A B} \frac{1}{\sqrt{2}}$
$\Rightarrow \frac{A B - A X}{A B} = \frac{1}{\sqrt{2}} \Rightarrow \frac{A B}{A B} - \frac{A X}{A B} = \frac{1}{\sqrt{2}}$
$\Rightarrow 1 - \frac{A X}{A B} = \frac{1}{\sqrt{2}} \Rightarrow \frac{A X}{A B} = 1 - \frac{1}{\sqrt{2}} = \frac{\sqrt{2} - 1}{\sqrt{2}}$
Hence , $\frac{A X}{A B} = \frac{2 - \sqrt{2}}{2}$

Updated on:21/07/2023

In $△ A B C$ , F and E are the points on sides AB and AC respectively, such that FE || BC and FE divides the triangle in two parts of equal area. If $A D ⊥ B C$ and AD intersect FE at G, then GD: AG = ?
$△ A B C$ में F और E क्रमशः भुजा AB और AC पर स्थित ऐसे बिंदु हैं कि FE || BC है तथा FE त्रिभुज को बराबर क्षेत्रफल वाले दो भागों में विभाजित करती है | यदि $A D ⊥ B C$ है और AD, FE को G पर काटती है, तो GD: AG = ?
A $\sqrt{2} : 1$
B $(\sqrt{2} - 1) : 1$
C $2 \sqrt{2} : 1$
D $(\sqrt{2} + 1) : 1$
In $Δ A B C$ , D and E are points on AB and AC respectively such that DE||BC and DE divides the $Δ A B C$ into two parts of equal areas. Then ratio of AD and BD is
A $1 : 1$
B $1 : \sqrt{2} - 1$
C $1 : \sqrt{2}$
D $1 : \sqrt{2} + 1$
X and Y are points on sides AB and AC respectively of $Δ A B C$ . For which of the following cases will XY be parallel to BC?
AAX = 1.3 cm, XB = 3.9 cm,
AY = 2.8 cm, YC = 5.6 cm,
BAX = 1.3 cm, XB = 3.9 cm,
AY = 2.8 cm, YC = 8.4 cm
CAX = 1.3 cm, XB = 2.6 cm,
AY = 2.8 cm, YC = 8.4 cm
DAX = 1.3 cm, XB = 2.6 cm,
AY = 2.8 cm, YC = 11.2 cm