If two sides and a median bisecting the third side of a triangle ar respectively proportional to the corresponding sides and median of the other triangle; then the two triangles are similar.
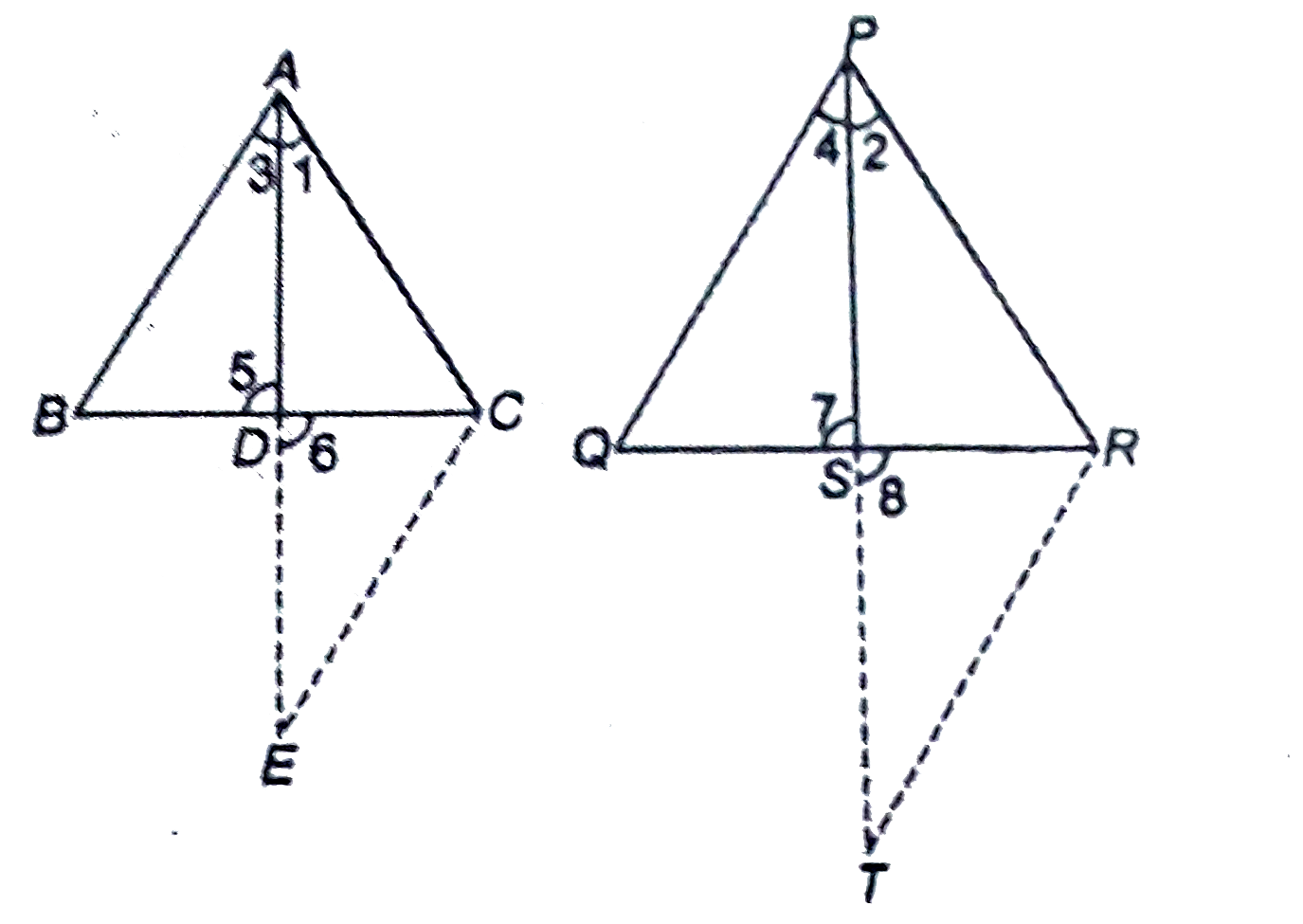
Given: △ABCand△PQR in which AD and PS are the medians such that.
ABPQ=ACPR=ADPS
To prove , △ABC~△PQR
construciton : produce AD to E such that AD =DE. Join EC. Also produce PS to T such that PS =ST. Join TR.
Proof: In △ABDand△ECD we have
⎧⎪⎨⎪⎩BD=CD(∵D is the mid point of BC as AD is the median)∠5=∠6(vertically opposite angles)AD=DE(construction)
△ABD≅△ECD (SAS criterion of congruency)
AB=EC (corresponding parts of congruent trinagles) ...(1)
similarly, we can prove
△PQS≅△TRS
PQ = TR .....(2)
Now, since ABPQ=ACPR=ADPS (given)
⇒ECTR=ACPR=2AD2PS [ from (1) and (2)]
⇒ECTR=ACPR=AEPT
△AEC~△PTR ( SSS criterion for similarity)
∠1=∠2 (corresponding angles of similar triangles are equal) ....(3)
Similarly , we can prove
∠3=∠4
Adding (3) and (4) , we get
∠1+∠3=∠2+∠4
⇒∠A=∠P
Now, in △ABCand△PQR
ABPQ=ACPR
and ∠A=∠P
△ABC~△PQR (SAS criterion for similarity) Hence proved.
Topper's Solved these Questions
- TRIANGLES Book:NAGEEN PRAKASHANChapter:TRIANGLES Exercise:Problems From NCERT/ Exemplar
Explore 14 Videos
- TRIANGLES Book:NAGEEN PRAKASHANChapter:TRIANGLES Exercise:Exercise 6a
Explore 23 Videos
- STATISTICS Book:NAGEEN PRAKASHANChapter:STATISTICS Exercise:Revision Exercise Long Answer Questions
Explore 5 Videos
- VOLUME AND SURFACE AREA OF SOLIDSBook:NAGEEN PRAKASHANChapter:VOLUME AND SURFACE AREA OF SOLIDSExercise:Revisions Exercise Long Answer Questions
Explore 5 Videos
Similar Questions
If two sides and a median bisecting one of these sides of a triangle are respectively proportional to the two sides and corresponding median of another triangle; then triangle are similar.
View SolutionTwo sides and the perimeter of one triangle are respectively three times the corresponding sides and the perimeter of the other triangle. Are the two triangles similar? Why?
View SolutionIs it true to say that, if in two triangles, an angle of one triangle is equal to an angle of another triangle and two sides of one triangle are proportional to the two sides of the other triangle, then the triangles are similar? Give reason for your answer.
View SolutionSides AB and AC and median AD of a triangle ABC are respectively proportional to sides PQ and PR and median PM of another triangle PQR. Prove that ΔABC~DetlaPQR
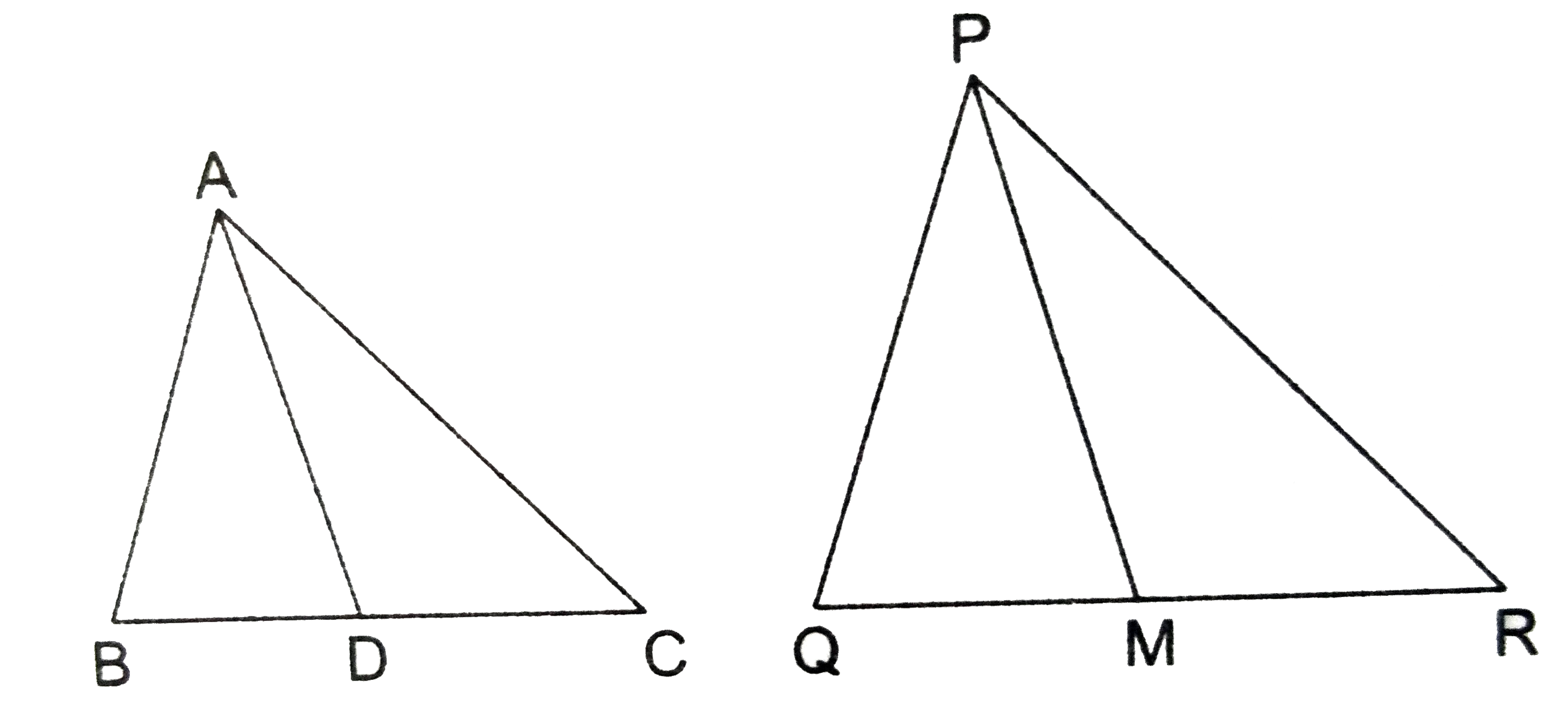
View SolutionSides AB and AC and median AD of a triangle ABC are respectively proportional to sides PQ and PR and median PM of another triangle PQR. Show that ΔABC~ΔPQR.
View SolutionIn two right triangles one side an acute angle of one are equal to the corresponding side and angle of the other. Prove that the triangles are congruent.
Let ABC and DEF be two right triangles such that
∠A=∠D,BC=EFand∠B=∠E=900
Thus, in △s ABC and DEF<br>/_A=/_D<br>/_B=/_E=90^0<br>BC=EFSo,by∀S≅ruencecriterion,weobta∈<br>/_\ABC~=/_\DEF
View SolutionTwo triangles are similar if their corresponding sides are ………….
View SolutionConsider the following statements : Two triangles are said to be congruent, if
1. Three angles of one triangle are equal to the corresponding three angles of the other triangle.
2. Three sides of one triangle are equal to the corresponding three sides of the other triangle,
3. Two sides and the included angle of one triangle are equal to the corresponding two sides and the included angle of the other triangle.
4. Two angles and the included side of one triangle are equal to the corresponding two angles and the included side of the other triangle.
Which of the above statements are correct ?
View SolutionWhich of the following statements are true (T) and which are false (F): Side opposite to equal angles of a triangle may be unequal. Angle opposite to equal sides of a triangle are equal. The measure of each angle of an equilateral triangle is 600. If the altitude from one vertex of a triangle bisects the opposite side, then the triangle may be isosceles. The bisectors of two equal angles of a triangle are equal. If the bisector of the vertical angle of a triangle bisects the base, then the triangle may be isosceles. The two altitudes corresponding to two equal sides of a triangle need not be equal. If any two sides of a right triangle are respectively equal to two sides of other right triangle, then the two triangles are congruent. Two right triangles are congruent if hypotenuse and a side of one triangle are respectively equal to the hypotenuse and a side of the other triangle.
View SolutionThe ratio of the area of two similar triangle is 4:5, the ratio of their corresponding sides are :
View Solution