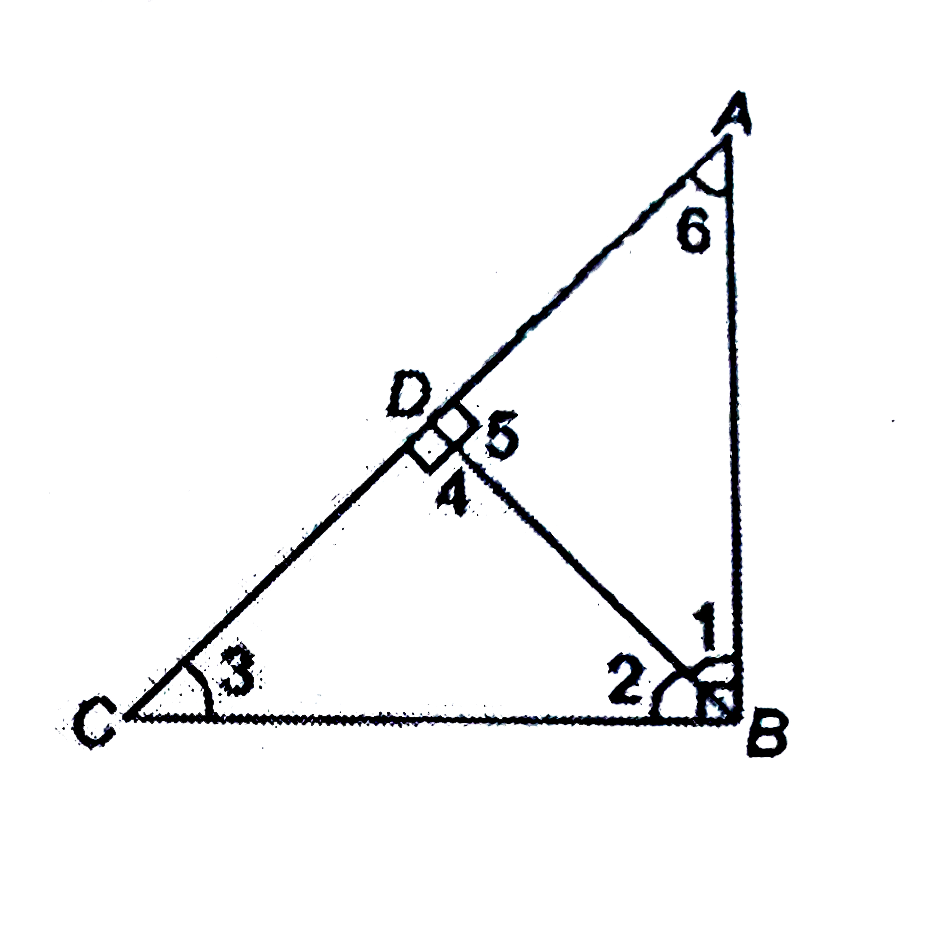
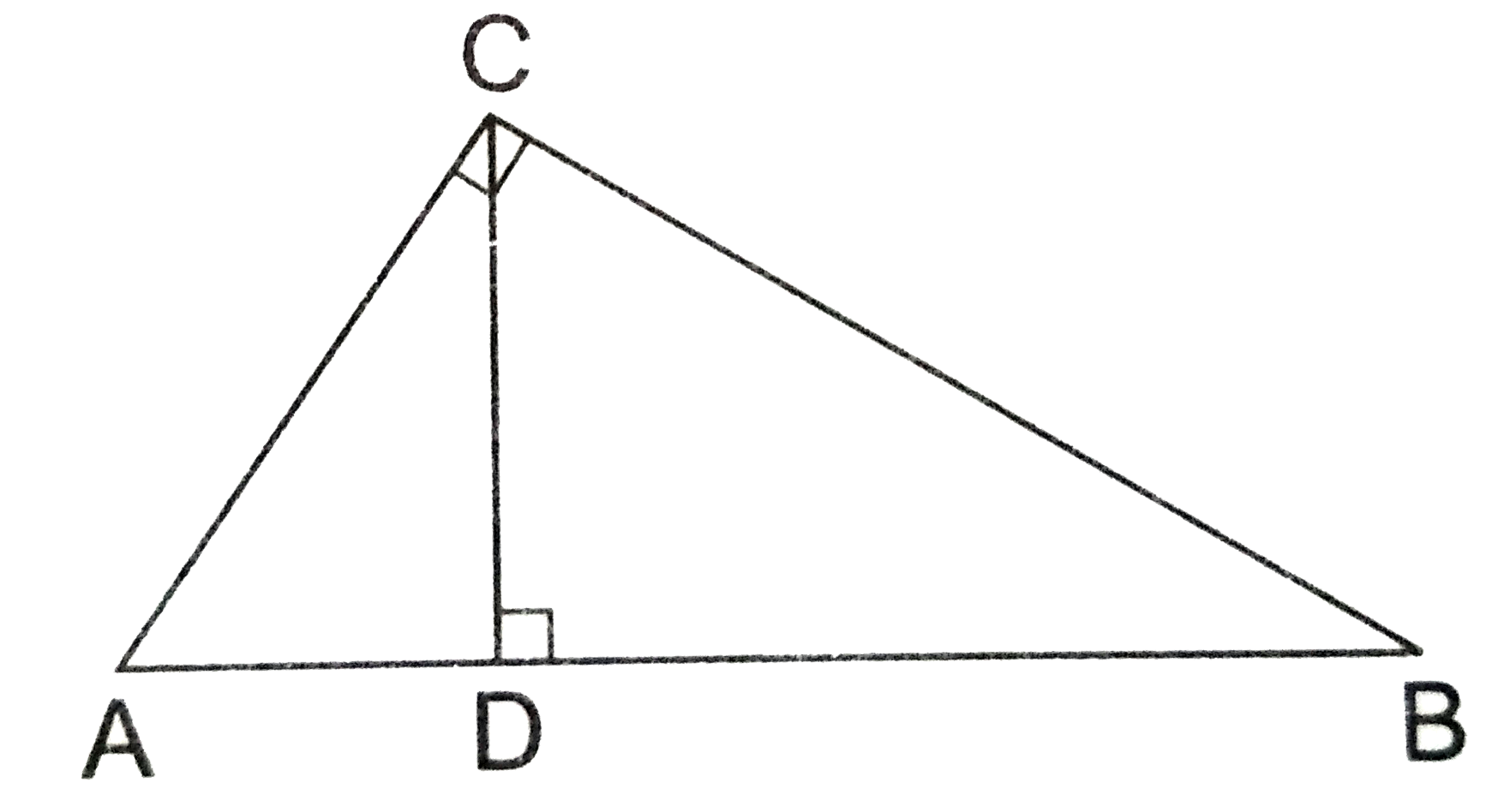
In the given figure if $∠ B = 90^{\circ}$ and BD is perpendicular to AC then
prove that:
$(i) △ A D B ~ △ B D C (i i) △ A D B ~ △ A B C$
(iii) $△ B D C ~ △ A B C (i v) B D^{2} = A D \times D C$
(v) $A B^{2} = A D \times A C (v i) B C^{2} = C D \times A C$
(vii) $A B^{2} + B C^{2} = A C^{2}$

Video Solution

Text Solution

Verified by Experts

(i) In $△ A D B and △ B D C$
$\begin{matrix} ∠ 5 = ∠ 4 (each 90^{\circ}) & Searching for second Angles \\ We know that ∠ 1 + ∠ 2 = 90^{\circ} ... . . (1) \\ Also, Since ∠ 4 = 90^{\circ} \\ ∴ ∠ 2 + ∠ 3 = 90^{\circ} \\ (angle sum property)...(2) \\ ∴ From (1) and (2) , We get \\ \Rightarrow ∠ 1 + ∠ 2 = ∠ 2 + ∠ 3 (e a c h 90^{\circ}) \\ \Rightarrow ∠ 1 = ∠ 3 \end{matrix}$
$∠ 1 = ∠ 3$ ( just proved)
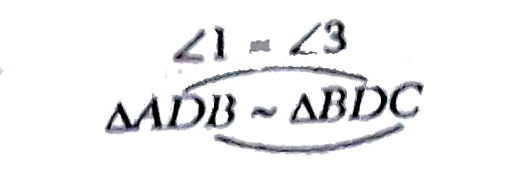(AA corollary) Hence proved.
(ii) $△ B D C and △ A B C$
$∠ 4 = ∠ 1 + ∠ 2 (each 90^{\circ})$
$∠ 3 = ∠ 3$ ( common)
(AA corollary) Hence poved.
(iii) In $△ B D C and t r \in ∠ A B C$
$∠ 4 = ∠ 1 + ∠ 2 (each 90^{\circ})$
$∠ 3 = ∠ 3$ (common)
[from part (i)
(corresponding sides of similar triangles are proportional)
(iv) For $B D^{2} = A D \times D C$ . we need to prove two $△ s$ similar which contain BD, AD and DC as sides.
Obviosly these are $△ A B D and △ B D C$ , we have proved these $△ s$ as similar in part (i). so ,
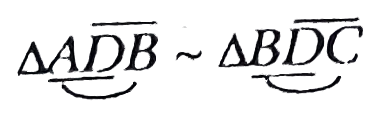[from part (ii)]
( corresponding sides of similar triangle are proportional)
$B D^{2} = A D \times D C$ Hence proved.
(v) For $A B^{2} = A D \times A C$ we need to prove two triangles similar which contain AB, AD and AC as sides. Obviosusly these are $△ A B D and △ A B C$ . we have already proved these $△ s$ as similar in part (ii)
$△ A D B ~ △ A B C$ [ from part (ii) ]
$\Rightarrow A B^{2} = A D \times A C$ .... (1) Hence proved
(vi) For $B C^{2} = C D \times A C$ we need to prove two triangles similar which contains BC, CD and AC as sides. Obviosly these are $△ B D C and △ A B C$ , we have proved these triangles as similar in part (iii)
so,
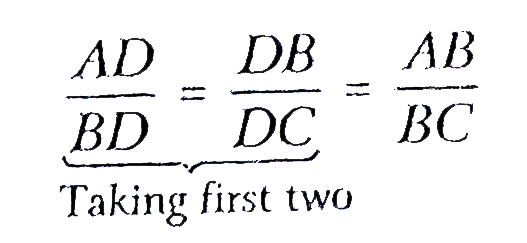( cossesponding sides of similar triangle are proportional)
$B C^{2} = C D \times A C$ ....(2) Hence proved.
(vii) Addings results (1) and (2), we get
$A B^{2} + B C^{2} = A D \times A C + C D \times A C$
$A C (A D + C D) < b r > = A C \times A C = A C^{2}$ Hence proved.

Updated on:21/07/2023

|

Class 10 MATHS TRIANGLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

In the given figure $A D = B C, A C = B D$ . Then $Δ P A B$ is

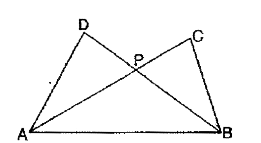Aequilateral
Bright angled
Cscalene
Disosceles
In $Δ A B C, ∠ C = 90^{\circ}$ and CD is perpendicular to AB at D. If $\frac{A D}{B D} = \sqrt{k}$ , then $\frac{A C}{B C} = ?$
Aa)k
Bb) $\sqrt{k}$
Cc) $\frac{1}{\sqrt{k}}$
Dd) $\sqrt[4]{k}$

NAGEEN PRAKASHAN-TRIANGLES -Revision Exercise Long Questions

Home
Ask Doubt
Profile