Prove that the least positive value of $x,$ satisfying $tan x = x + 1,$ lies in the interval $(\frac{π}{4}, \frac{π}{2})$

Video Solution

Text Solution

Verified by Experts

Let f(x) = tan x and g(x) = x + 1, which could be shown as follows.

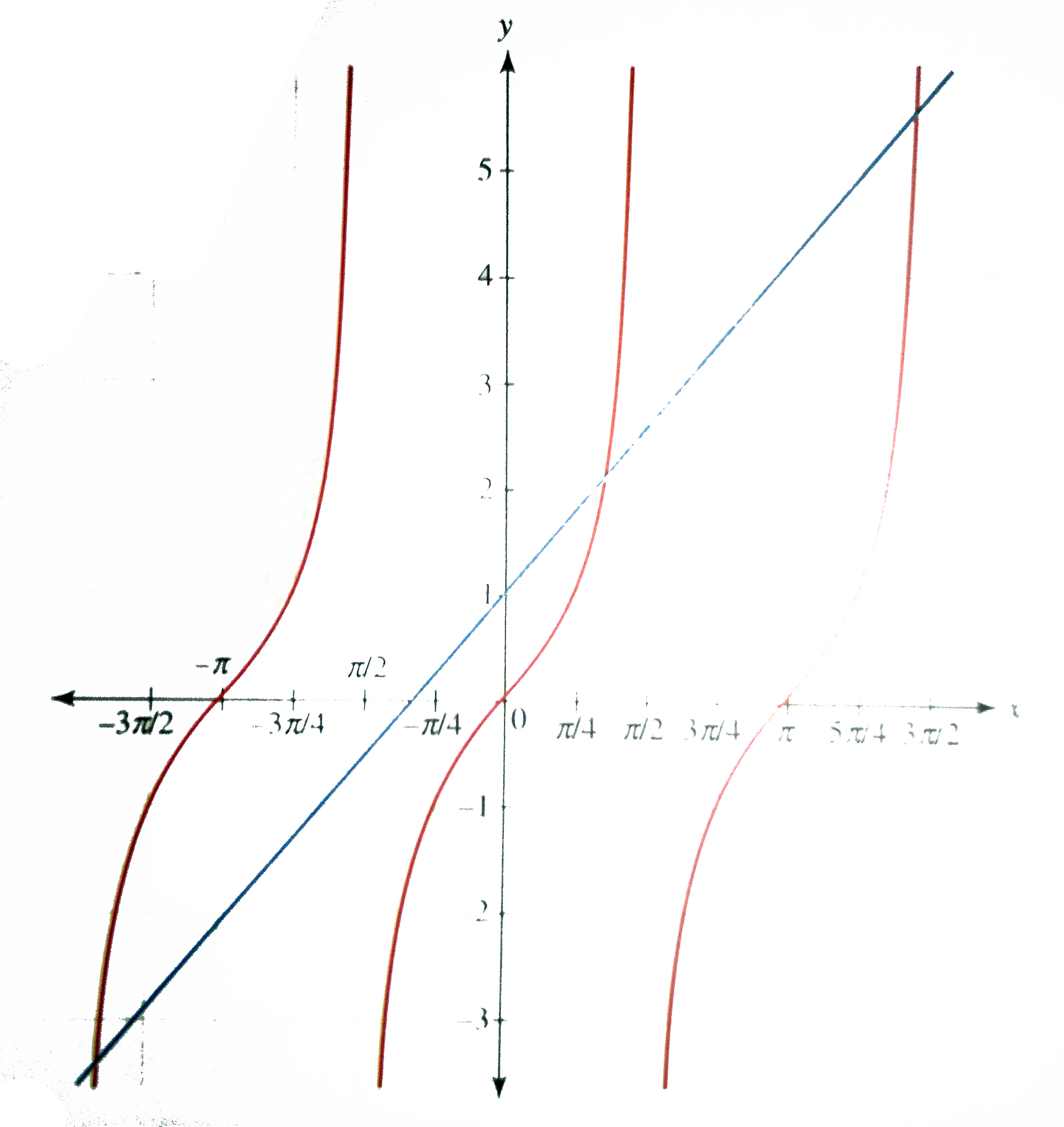
From the above the figure, tan x = x + 1 has infinitely many solutions, but the least positive value of $x \in (\frac{π}{4}, \frac{π}{2}) .$

|

Updated on:21/07/2023

Class 12 MATHS GRAPHS OF TRIGONOMETRIC FUNCTIONS

Topper's Solved these Questions

BOOK - CENGAGECHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise
22videos
GRAPHS OF TRIGONOMETRIC FUNCTIONS
BOOK - CENGAGECHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - Exercise
34videos
GRAPHS OF ELEMENTARY FUNCTIONS
BOOK - CENGAGECHAPTER - HIGHT AND DISTANCE EXERCISE - JEE Previous Year
3videos
HIGHT AND DISTANCE

Similar Questions

Knowledge Check

Question 1 - Select One
The least positive root of the function $sin x - π / 2 + 1 = 0$ lies in the interval
A $[0, π / 2]$
B $π / 2, π]$
C $[π / 2, 3 π / 2]$
Dnone of these
Question 2 - Select One
The greatest possible value of the expression $tan x + cot x + cos x$ on the interval $[π / 6, π / 4]$ is
A $\frac{12}{5} \sqrt{2}$
B $\frac{11}{6} \sqrt{2}$
C $\frac{12}{5} \sqrt{3}$
D $\frac{11}{6} \sqrt{3}$
Question 3 - Select One
The number of values of x satisfying the condition $sin x + sin 5 x = sin 3 x$ in the interval $[0, π]$ is
A6
B2
C10
D0

CENGAGE-GRAPHS OF TRIGONOMETRIC FUNCTIONS-Exercise

Home
Ask Doubt
Profile