A bead slides along a frictionless wire lying on a horizontal plane. It makes an anlge of 45∘ with x-axis as show in figure. In addition to any normal forces exerted by the wire, the bead is subject to an external force that depends on position according to formula.
→F=F0(xx0)2ˆi+F0(yy0)2ˆj
If the bead has speed v0 at the origin O, find its speed (in m/s) as it leaves the end P of wire Given data : F0=1N,x0=15m,y0=15m, mass of bead=2kg Initially kinetic energy of bead = 26 J
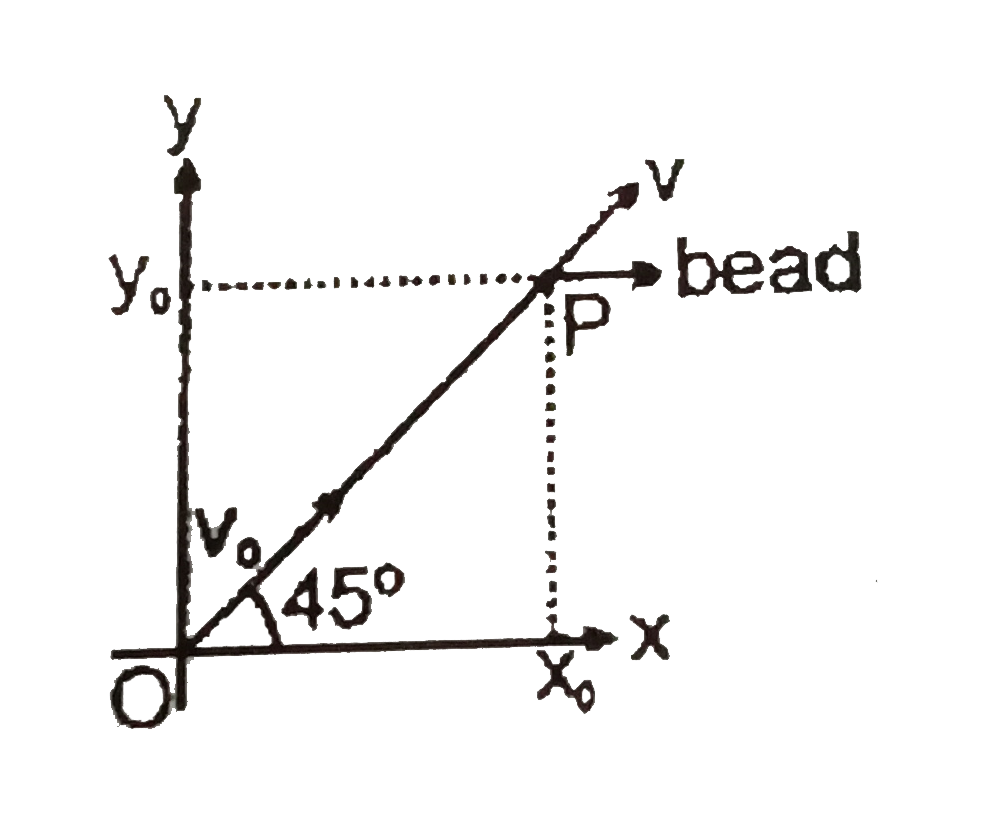
The correct Answer is:6
WDF=∫→F.d→r
=F0x20x0∫0x2dx+F0y20y0∫0y2dy
=F03(x0+y0)=10J
WD=kl−kL
10 =kl−26 implies kl=36
implies 12×2×v2=36
∴ v=6m/s
Topper's Solved these Questions
Similar Questions
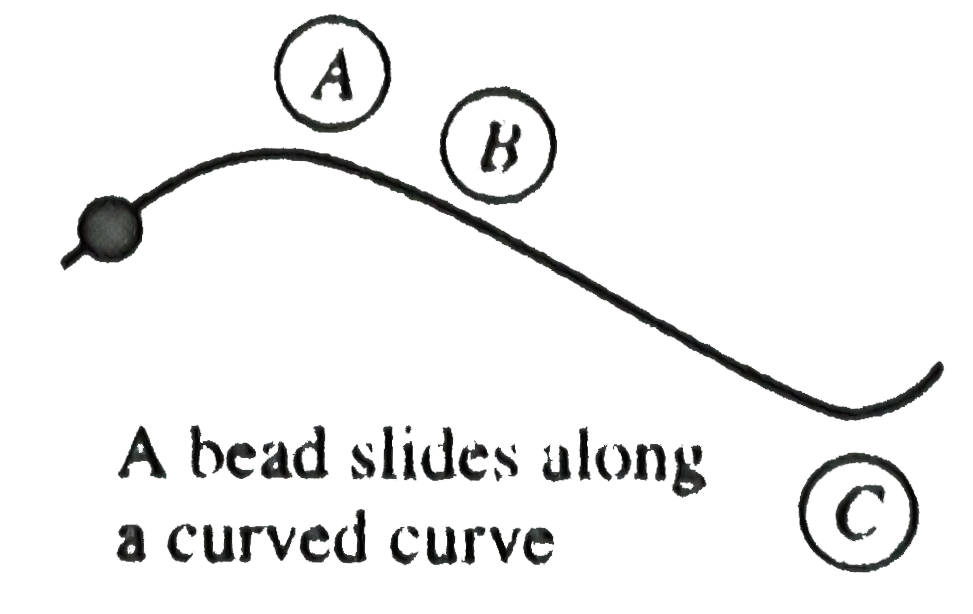
A bead slide freely along a curved wire lying on a horizontal surface at constant speed as shown in Fig
a. Draw the vectors repesenting the force exerted by the wire on the bead at point A ,B and C
b. Suppose the bead in figure speeds up with constant tangential acceleration as it moves toward the right Draw the vector representing the force on the bead at point A B and C
View SolutionA wire forming one cycle of sine curve is moved in x-y plane with velocity →V=Vxˆi+Vyˆj. There exist a magnetic field →B=−B0ˆk.
Find the motional emf decelop across the ends PQ of wire.
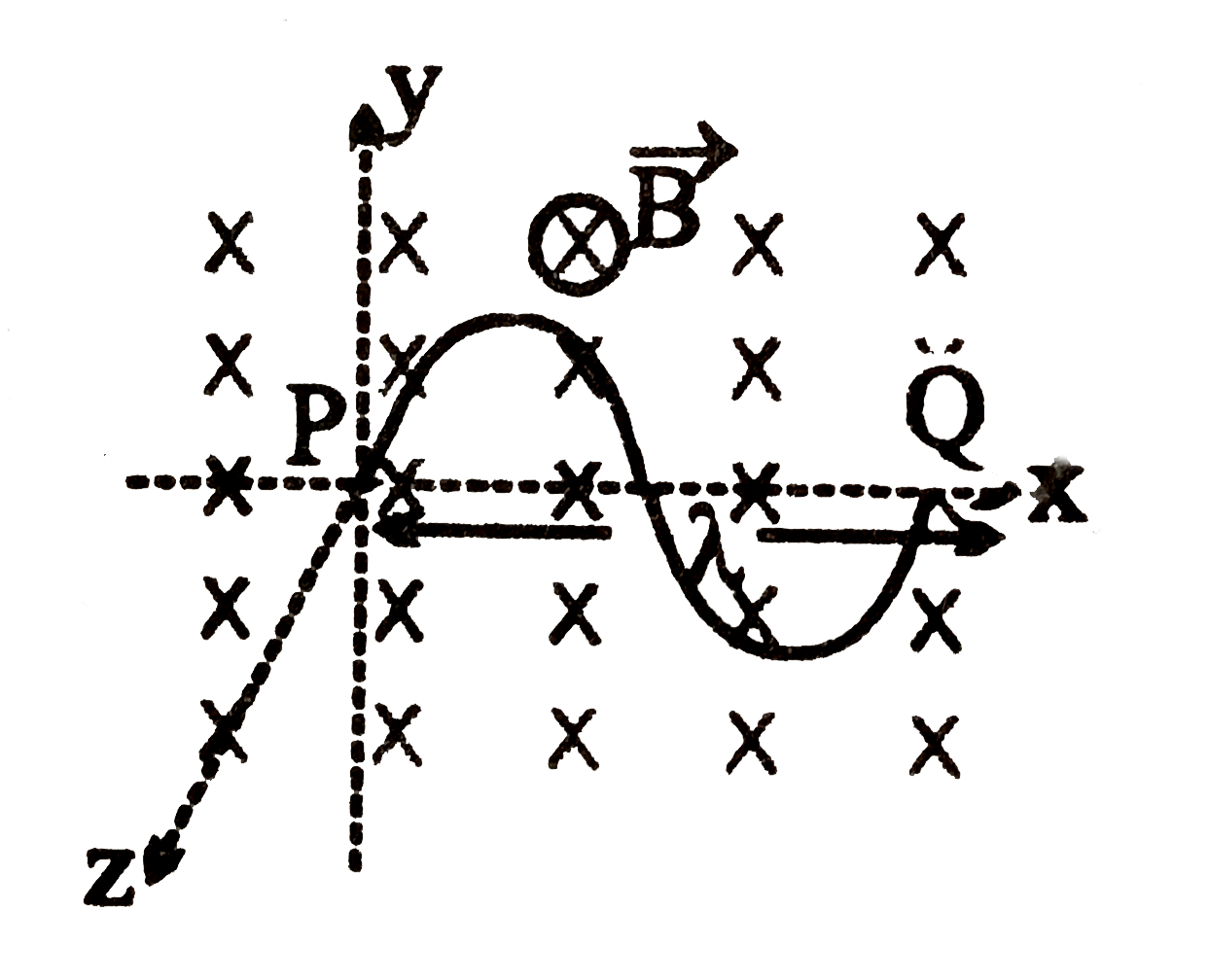
View Solution
Knowledge Check
A bead slides on a fixed frictionless wire bent into a horizontal semicircle of radius R0 as shown in figure. In addition to any normal forces exerted by the wire, the bead is subjected to an external force that points directly away from origin and depends on distance r from the origin according to the formula F=F0(rR0)2ˆr
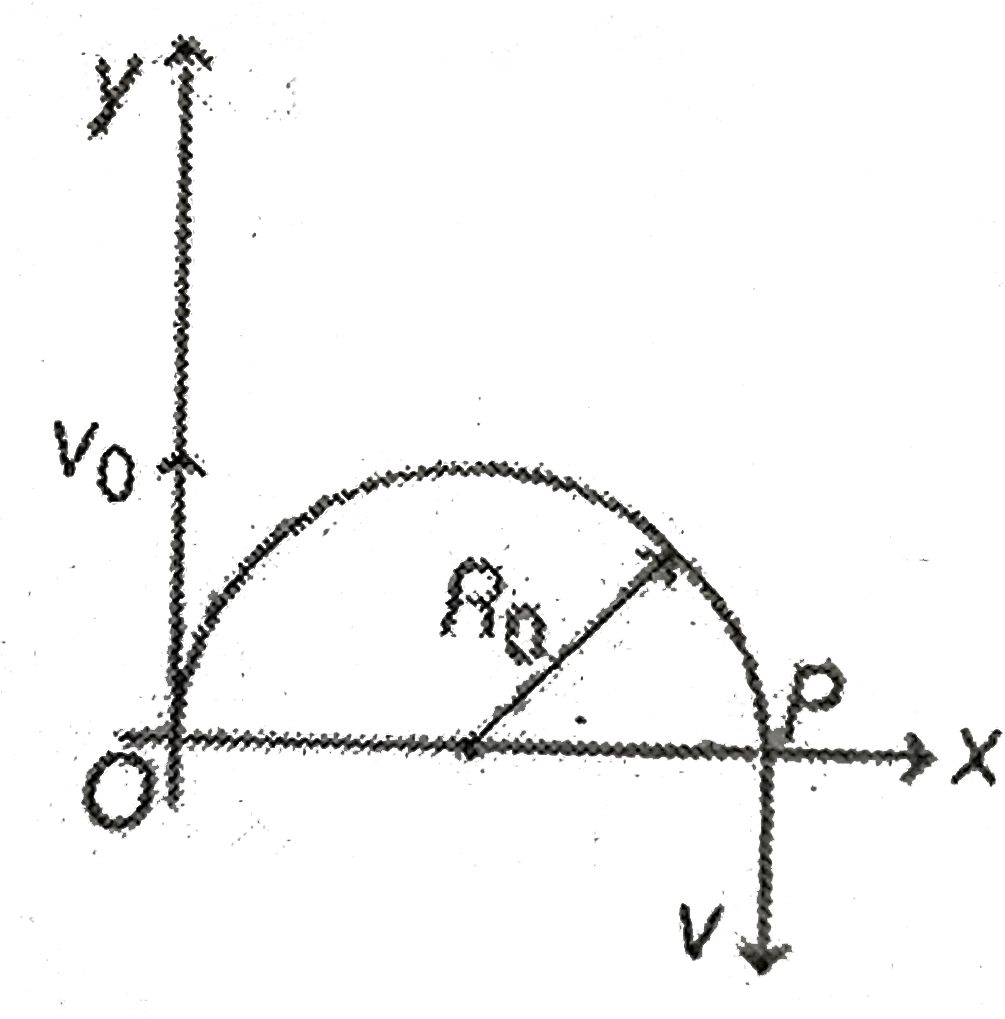 AGiven force is a central forceBGiven force is a conservation forceCWork done by external force as bead leaves the track (starting from origin) is 8F0R03DSpeed v of bead as it leaves the wire at P is √v20+18F0R03m
AGiven force is a central forceBGiven force is a conservation forceCWork done by external force as bead leaves the track (starting from origin) is 8F0R03DSpeed v of bead as it leaves the wire at P is √v20+18F0R03mA bead is moving on a frictionless fixed circular wire of radius 0.8 m in horizontal plane with constant speed & there is no interaction force between bead & wire & it is also connected with string & spring .
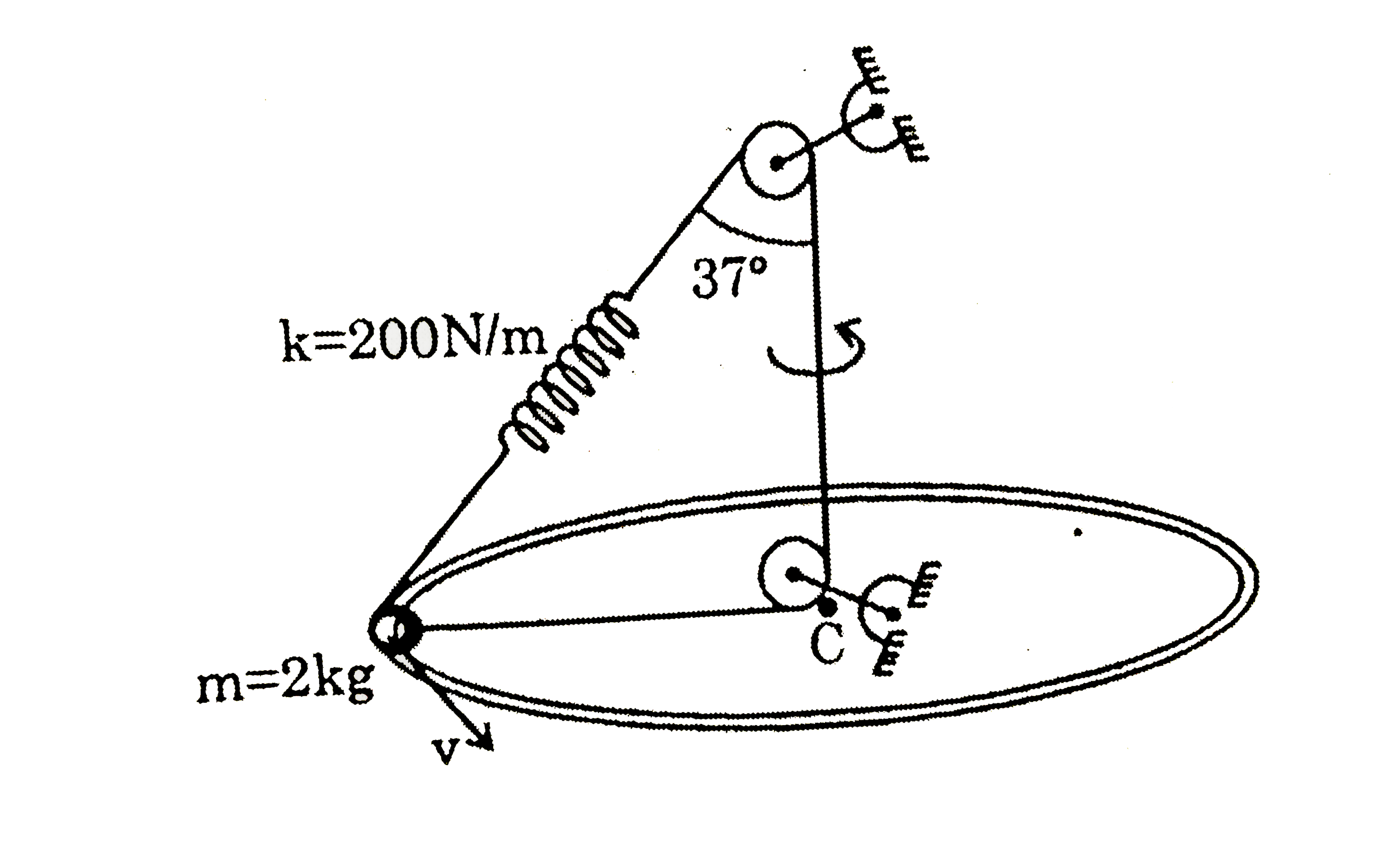 ASpeed of bead is 4m/secBElongation in spring is 10 cmCIf we cut the string , normal force due to wire on bead is 20√5N.DIf we cut the spring , normal force due to wire is 20√5N.
ASpeed of bead is 4m/secBElongation in spring is 10 cmCIf we cut the string , normal force due to wire on bead is 20√5N.DIf we cut the spring , normal force due to wire is 20√5N.In the shown figure two beads slide along a smooth horizontal rod as shown in figure. The relation between v and v0 in the shown position will be
 Av=v0cotθBv=v0sinθCv=v0tanθDv=v0cosθ
Av=v0cotθBv=v0sinθCv=v0tanθDv=v0cosθ
A bead moves along a straight horizontal wire of length L, starting from the left end with velocity v0. Its retardation is proportional to the distance that remains to the right end of the wire. Find the initial retardation (at left end of the wire) if the bead reaches the right end of the wire with a velocity v02.
View SolutionA particle of mass m is subject to a force (F) .Find the time dependent position x(t) of the particle for the following two cases : (a) F(x)=kx, with k>0, the initial position is x0, and the initial speed is zero. (b) F(v)=−bv2 and the initial position is zero,and the initial speed is v0.
View SolutionA particle is moved inx−y plane form (a,0) to (0,a) along circular arc having centre at origin. Work done by a force −ky(x2+y2)32ˆi+kx(x2+y2)32ˆj on the particle during this motion will be
View SolutionAn object of mass m has a speed v0 as it passes throgh the origin. Origin. It subjected to a retaeding force given by F(x)=−Ax. Here, A is a positive constant. Find its x-coordinate when it stops.
View SolutionTwo forces are acting on a same body −→F1=(2ˆi+3ˆj−ˆk) and −→F2=(ˆi+2ˆj+4ˆk), then find component of −→F1 along −→F2.
View Solution