A ring of radius r is moving without slipping on a circular track of radius R speed of the centre of the ring is v=ωr. Ignoring the fact that the ring axis is inclined
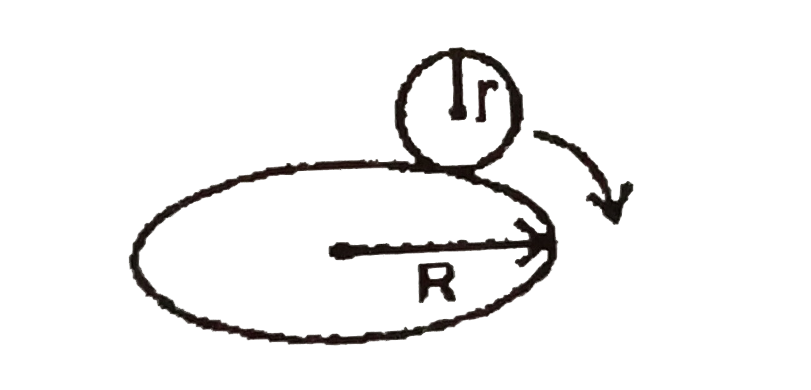
The radial acceleration of centre of the ring is ω2r2R
The radial acceleration of centre of the ring is (R−r)2Rω2
The angular velocity of centre of the ring about vertical axis passing through the centre of track is rω/R.
The angular velocity of centre of the ring about vertical axis passing through the centre of track is (R−r)Rω
The correct Answer is:A, C
velocity of centre of ring is = rω
Topper's Solved these Questions
Similar Questions
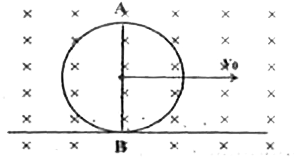
A conducting rim of radius R rolls without slipping on a horizontal surface . The speed of the ring 's centre of mass is v0 . A uniform magnetic field B perpendicular to the ring 's plane is swithched on. The emf induced across point of contact and the topmost point of the rim is 2Bv0R.
View SolutionA ring of radius R is with a uniformly distributed charge Q on it .A charge q is now placed in the centre of the ring .Find the increment in tension in the ring.
View Solution
Knowledge Check
A ring of radius R is rolling purely on the outer surface of a pipe of radius 4R At some instant the center of the ring has constant speed =v then the acceleration of the point on the ring which is in contact with the surface of the pipe is
A4v2/5RB3v2/5RCv2/4RDzeroCharges q is uniformly distributed over a thin half ring of radius R. The electric field at the centre of the ring is
Aq2π2ε0R2Bq4π2ε0R2Cq4πε0R2Dq2πε0R2A half ring of radius r has a linear charge density λ.The potential at the centre of the half ring is
Aλ4εoln0Bλ4π2ε0rCλ4πε0rDλ4πε0r2
A particle of mass m is kept on the axis of a fixed circular ring of mass M and radius R at a distance x from the centre of the ring. Find the maximum gravitational force between the ring and the particle.
View SolutionA ring of mass m and radius r rolls without slipping on a fixed hemispherical surface of radius R as shown. The time period of small oscillations of ring is 2π√n(R−r)3g then find the value of n.

View SolutionA quarter ring of radius R is having uniform charge density λ. Find the electric field and potential at the centre of the ring.
View SolutionA positive charge Q is uniformly distrubuted along a circular ring of radius R.a small test chage q is placed at the centre of the ring .The

View SolutionFor a uniform ring of mass M and radius R at its centre
View Solution
