A wire forming one cycle of sine curve is moved in x-y plane with velocity $\to V = V_{x} ˆ i + V_{y} ˆ j$ . There exist a magnetic field $\to B = - B_{0} ˆ k$ .
Find the motional emf decelop across the ends PQ of wire.

Video Solution

Text Solution

Verified by Experts

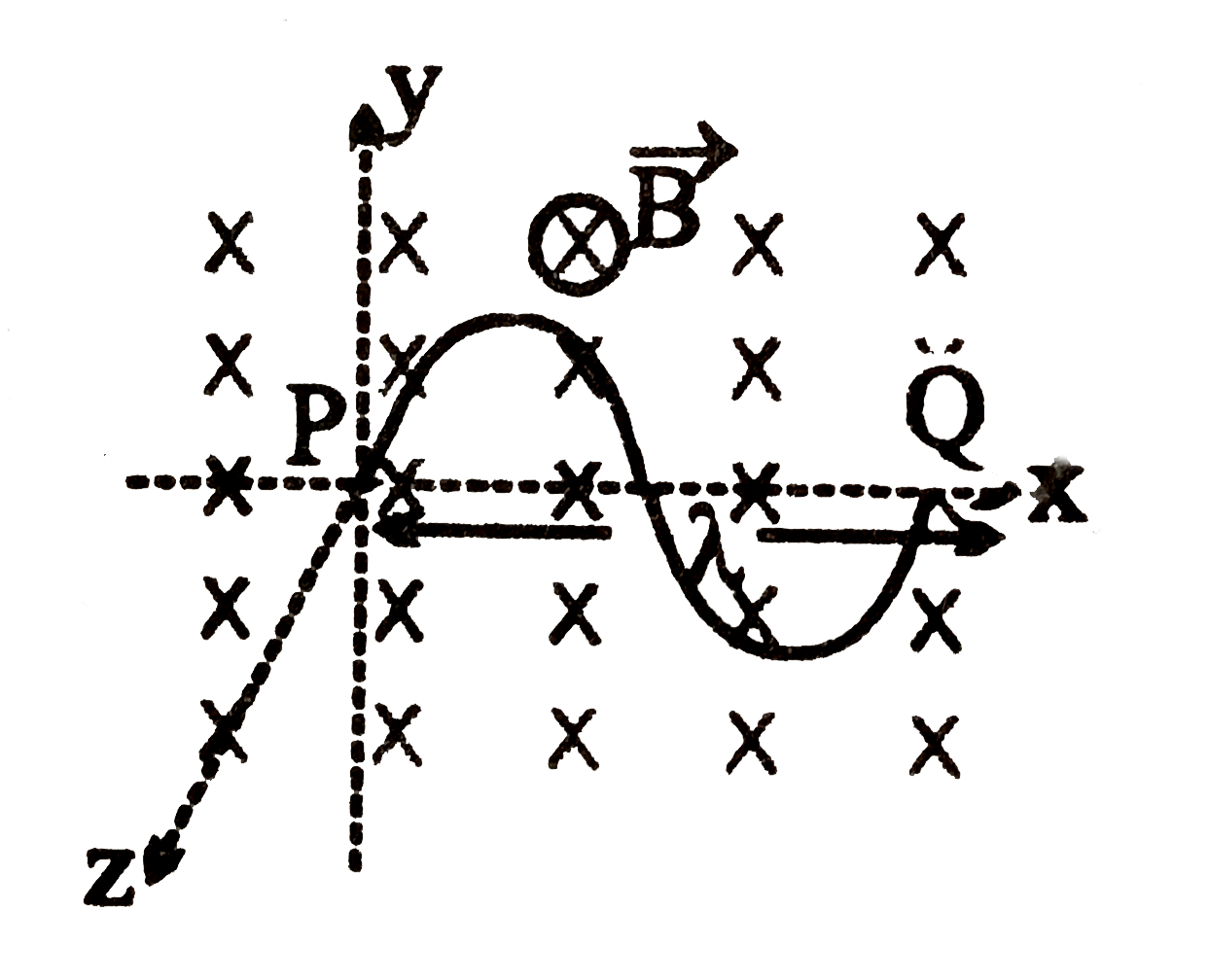
The correct Answer is: $λ V_{y} B_{0}$

$e = - (\to B \times \to V) . \to l = - [B_{0} ˆ k \times (V_{x} ˆ i + V_{y} ˆ j] . λ ˆ i = B_{0} V_{y} λ$

|

Updated on:21/07/2023

Class 12 PHYSICS ELECTROMAGNETIC INDUCTION

Similar Questions

Knowledge Check

Question 1 - Select One
A conductor $A B$ of length $l$ moves in $x y$ plane with velocity $\to v = v_{0} (ˆ i - ˆ j)$ . A magnetic field $\to B = B_{0} (ˆ i + ˆ j)$ exists in the region. The induced emf is
Azero
B $2 B_{0} l v_{0}$
C $B_{0} l v_{0}$
D $\sqrt{2} B_{0} l v_{0}$
Question 2 - Select One or More
A portion is fired from origin with velocity $\to v = v_{0} ˆ j + v_{0} ˆ k$ in a uniform magnetic field $\to B = B_{0} ˆ j$ . In the subsequent motion of the proton
Aits z co-ordinate can never be negative
Bits x co-ordinate can never be positive
Cits x and z co-oridinate cannot be zero at the same time
Dits y co-ordinate will be proportional to its time of flight
Question 3 - Select One
An electron is projected wit velocity $\to v = v_{0} ˆ x$ in an electric field $\to E = E_{0} ˆ y$ . Trace the path followed by the electron :-
AParabola
BCircle
CStraight line in +y direction
DStraight line in -y direction

Recommended Questions

Home
Ask Doubt
Profile