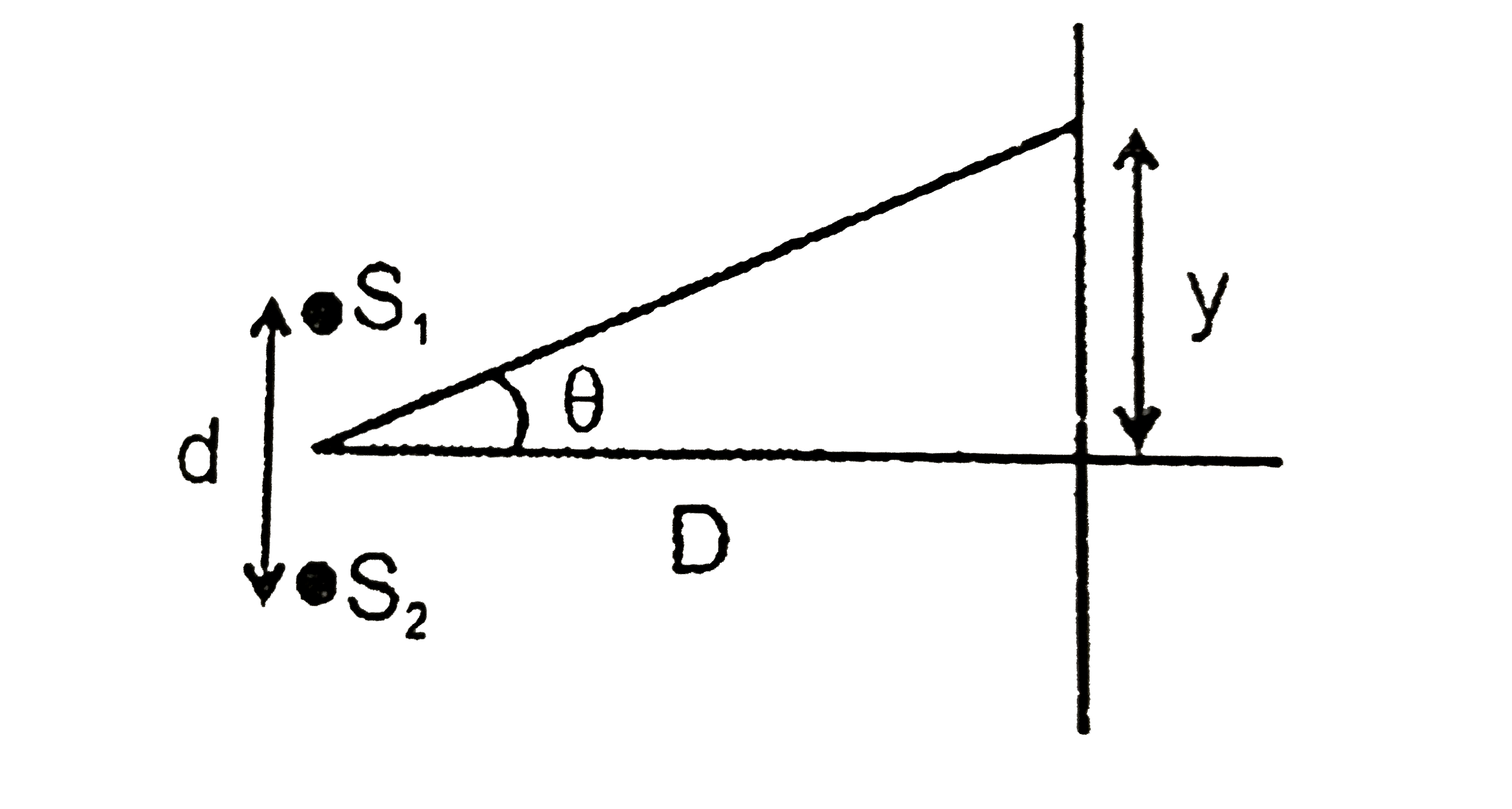
In a YDSE, $D = 1 m, d = 1 m m, and λ = 1 / 2 m m .$
(a) Find the distance between the first and central maxima on the screen.
(b) Find the number of maxima and minima obtained on the screen.

Video Solution

Text Solution

Verified by Experts

(i) D gt gt d $< b r > H e n c e Δ P = d sin θ$
$\frac{d}{λ} = 2,$
clearly, $n < < \frac{d}{λ} = 2$ is not possible for any value of $n .$
Hence $Δ p = \frac{d y}{D}$ cannot be used
for lst maxima,
$Δ p = d sin θ = λ$
$\Rightarrow sin θ = \frac{λ}{d} = \frac{1}{2}$
$\Rightarrow θ = 30^{\circ}$ Hence, $y = D tan θ = \frac{1}{\sqrt{3}}$ meter

(ii) Maximum path difference
$Δ P_{max} = d = 1 m m$
$\Rightarrow$ Highest order maxima, $n_{max} = [\frac{d}{λ}] = 2$ and highest order minima $n_{min} = [\frac{d}{λ} + \frac{1}{2}] = 2$
Total no, of maxima $= 2 n_{max} + 1^{\cdot} = 5$ .^(*)("central maxima") $. < b r > T o t a l n ⊙ o f min i m a$ =2n_(min)=4

Updated on:21/07/2023

In YDSE, $D = 1 m, d = 1 m m$ , and $λ = 5000 n m$ . The distance of the 100th maxima from the central maxima is
A $\frac{1}{2} m$
B $\frac{\sqrt{3}}{2}$ m
C $\frac{1}{\sqrt{3}}$ m
Ddoes not exist
In YDSE $a = 2 m m$ , D = 2m, $λ = 500$ mm. Find distance of point on screen from central maxima where intensity becomes $50 %$ of central maxima
A $1000 μ m$
B $500 μ m$
C $250 μ$ mu
D $125 μ$ m
In a YDSE experiment, d = 1mm, $λ$ = 6000Å and D= 1m. The minimum distance between two points on screen having 75% intensity of the maximum intensity will be
A0.50 mm
B0.40 mm
C0.30 mm
D0.20 mm