For a damped oscillator the mass 'm' of the block is 200 g, k = 90 N m−1 and the damping constant b is 40 g s−1. Calculate the period of oscillation.
0.2
0.3
0.5
0.1
The correct Answer is:B
To solve the problem of calculating the period of a damped oscillator, we will follow these steps:
Step 1: Convert the mass to SI units
The mass m is given as 200 g. To convert this to kilograms (the SI unit for mass), we use the conversion factor:
m=200g=200×10−3kg=0.2kg
Step 2: Identify the spring constant
The spring constant k is given as:
k=90N/m
Step 3: Convert the damping constant to SI units
The damping constant b is given as 40 g/s. To convert this to kg/s, we use the conversion factor:
b=40g/s=40×10−3kg/s=0.04kg/s
Step 4: Calculate the damped angular frequency ωd
The formula for the damped angular frequency ωd is given by:
ωd=√km−(b2m)2
First, we calculate km:
km=90N/m0.2kg=450s−2
Next, we calculate b2m:
b2m=0.04kg/s2×0.2kg=0.040.4=0.1s−1
Now, we square this value:
(b2m)2=(0.1)2=0.01s−2
Now we can find ωd:
ωd=√450−0.01=√449.99≈21.21rad/s
Step 5: Calculate the period of oscillation T
The period T of the damped oscillator is given by:
T=2πωd
Substituting the value of ωd:
T=2π21.21≈6.283221.21≈0.296s
Step 6: Round the answer
Rounding 0.296s gives us approximately:
T≈0.3s
Final Answer
The period of oscillation for the damped oscillator is approximately 0.3s.
---
The mass m is given as 200 g. To convert this to kilograms (the SI unit for mass), we use the conversion factor:
m=200g=200×10−3kg=0.2kg
The spring constant k is given as:
k=90N/m
The damping constant b is given as 40 g/s. To convert this to kg/s, we use the conversion factor:
b=40g/s=40×10−3kg/s=0.04kg/s
The formula for the damped angular frequency ωd is given by:
ωd=√km−(b2m)2
First, we calculate km:
km=90N/m0.2kg=450s−2
b2m=0.04kg/s2×0.2kg=0.040.4=0.1s−1
(b2m)2=(0.1)2=0.01s−2
ωd=√450−0.01=√449.99≈21.21rad/s
The period T of the damped oscillator is given by:
T=2πωd
Substituting the value of ωd:
T=2π21.21≈6.283221.21≈0.296s
Rounding 0.296s gives us approximately:
T≈0.3s
The period of oscillation for the damped oscillator is approximately 0.3s.
Topper's Solved these Questions
- OSCILLATIONSBook:NARAYNAChapter:OSCILLATIONSExercise:C. U . Q
Explore 75 Videos
- OSCILLATIONSBook:NARAYNAChapter:OSCILLATIONSExercise:EXERCISE - I (C.W)
Explore 63 Videos
- OSCILLATIONSBook:NARAYNAChapter:OSCILLATIONSExercise:EVALUATE YOURSELF - 3
Explore 5 Videos
- NEWTONS LAWS OF MOTIONBook:NARAYNAChapter:NEWTONS LAWS OF MOTIONExercise:PASSAGE TYPE QUESTION
Explore 6 Videos
- PHYSICAL WORLDBook:NARAYNAChapter:PHYSICAL WORLDExercise:C.U.Q
Explore 10 Videos
Similar Questions
For the damped oscillator shown in Fig, the mass of the block is 200g,k=80Nm−1 and the damping constant b is 40gs−1 Calculate
(a) The period of oscillation
(b) Time taken for its amplitude of vibrations to drop to half of its initial values
(c) The time for the mechanical energy to drop to half initial values

View SolutionFor the damped oscillator shown in previous Figure, the mass m of the block is 400 g, k=45Nm−1 and the damping constant b is 80gs−1. Calculate .
(a) The period of osciallation ,
(b) Time taken for its amplitude of vibrations to drop to half of its initial value and
(c ) The time taken for its mechanical energy to drop to half its initial value.
View Solution
Knowledge Check
For the small damping oscillator, the mass of the block is 500 g and value of spring constant is k = 50 N/m and damping constant is 10 g s^(-1) The time period of oscillation is (approx.)
A2πsB2π13sCπ5sDπ√5s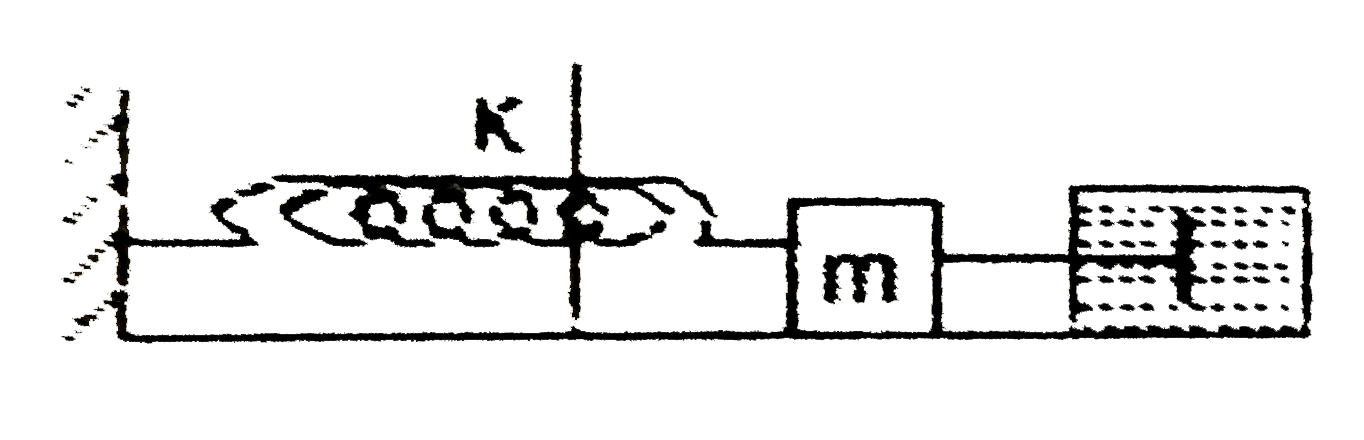
For a damped oscillator which follow the equation →F=−k→x−b→V
the mass of the block is 100 gm K=100N/M and the damping constant is 20 gm/sec. thhen find the time taken for its mechanical energy to drop to one-fourth of its initial value.A1.73B3.46C6.93D13.84There is a LCR circuit , If it is compared with a damped oscillation of mass m oscillating with force constant k and damping coefficient 'b'. Compare the terms of damped oscillation with the devices in LCR circuit.
AL→m,C→1k,R→bBL→m,c→R→bCL→K,C→b,R→mDL→1m,C→1k,R→1b
For the damped oscillator shown in previous figure, k=180Nm−1 and the damping constant b is 40gs−1 .Period of oscillation is given as 0.3 s, find the mass of the block . (Assume b is much less than √km).
Hint :T=2π√mk
View SolutionFor the damped oscillator shown in previous figure, k=180Nm−1 and the damping constant b is 40gs−1 .Period of oscillation is given as 0.3 s, find the mass of the block . (Assume b is much less than √km).
View SolutionIn damped oscillatory motion a block of mass 20kg is suspended to a spring of force constant 90N/m in a medium and damping constant is 40g/s. Find (a) time period of oscillation (b) time taken for amplitude of oscillation to drop to half of its intial value (c) time taken for its mechanical energy to drop to half of its initial value.
View SolutionThe time period of small oscillations of mass m :-
_S01_040_Q01.png)
View SolutionFind the time period of oscillation of block of mass m. Spring, and pulley are ideal. Spring constant is k.

View Solution