Instantaneous angle (in radian) between string of a simple pendulum and verical is given by θ=π180sin2πt. Find the length of the pendulum if g=π2m/s2.
The correct Answer is:0.25m
Step by step video, text & image solution for Instantaneous angle (in radian) between string of a simple pendulum and verical is given by theta = (pi)/(180) sin2pit. Find the length of the pendulum if g = pi^(2) m//s^(2). by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 1, PART - II
37 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 2, PART - I
26 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Board Level Exercise
24 Videos
- SEMICONDUCTORSBOOK - RESONANCECHAPTER - SEMICONDUCTORSEXERCISE - Exercise 3
88 Videos
- TEST PAPERSBOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - FST-3
30 Videos
Similar Questions
Knowledge Check
The period of a simple pendulum is double, when its length is increased by 1.2 m. The original length of the simple pendulum is
A0.2 mB0.3 mC0.4 mD0.5 mA simple pendulum is oscillating at 34th of a second, where g = 980 cm sec2, the length of the pendulum is
A55.85 cmB48.5 cmC13.96 cmD42.2 cmThe instantaneous displacement of a simple pendulum oscillator is given by x=Acos(ωt+π4)Its speed will be maximum at time
Aπ4ωBπ2ωCπωD2πω
Find the length of seconds pendulum at a place where g=π2m/s2.
View SolutionIf sin(πcosθ)=cos(πsinθ) . Find sin(2θ)
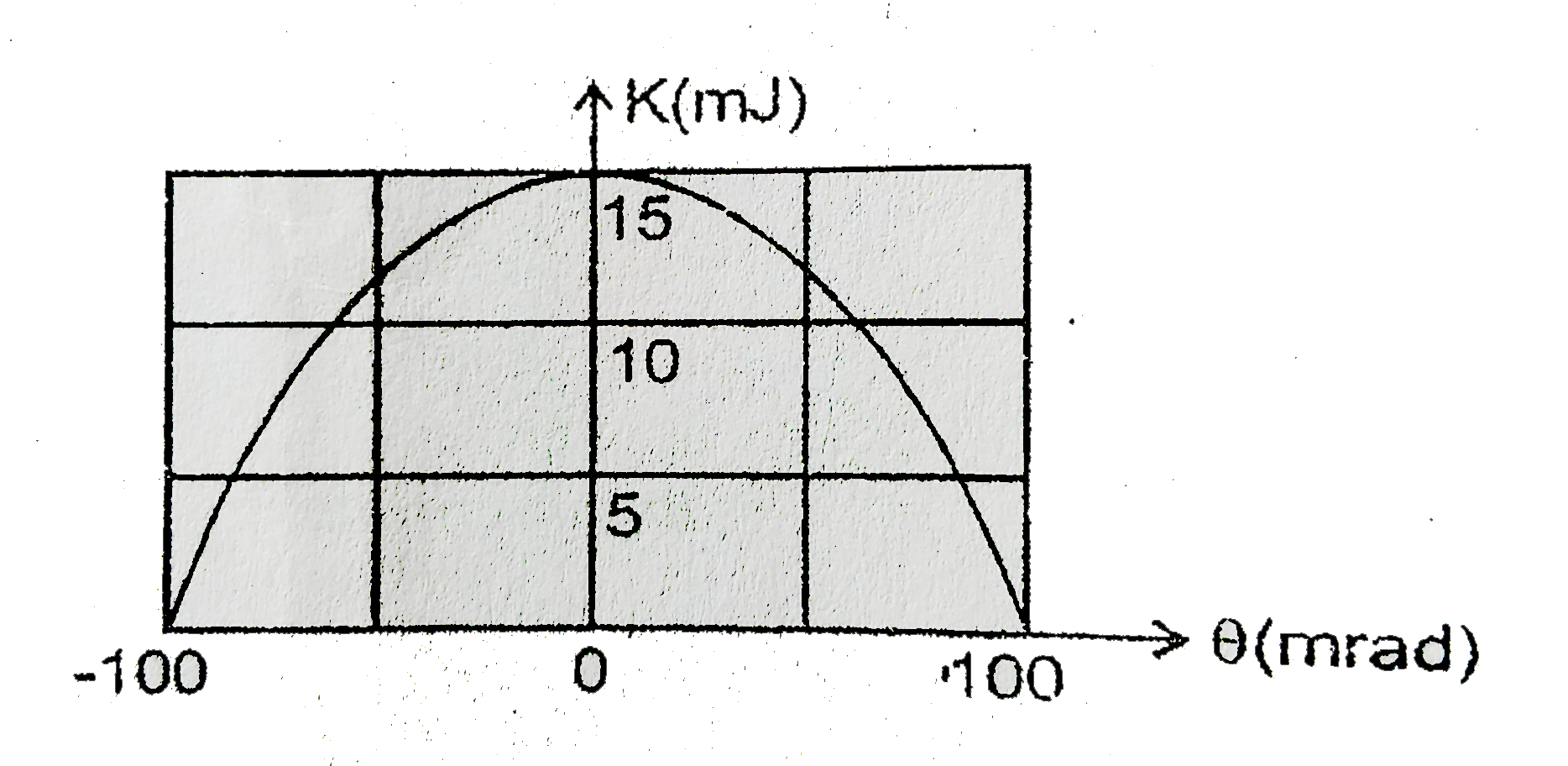
View SolutionFigure shows the kinetic energy K of a simple pendulum versus its angle θ from the verticle. The pendulum bob has mass 0.2kg. If the length of the pendulum is equal to ng meter. Then find n(g=10m/s2)
View SolutionThe instantaneous displacement of a simple pendulum oscillator is given by x=Acos(ωt+π4)Its speed will be maximum at time
View SolutionA graph is drawn between the displacement and time taken for the oscillations of a simple pendulum. The length of the penddulum is _______ m ( if g = 10 s−2andπ2=10)
View Solution
