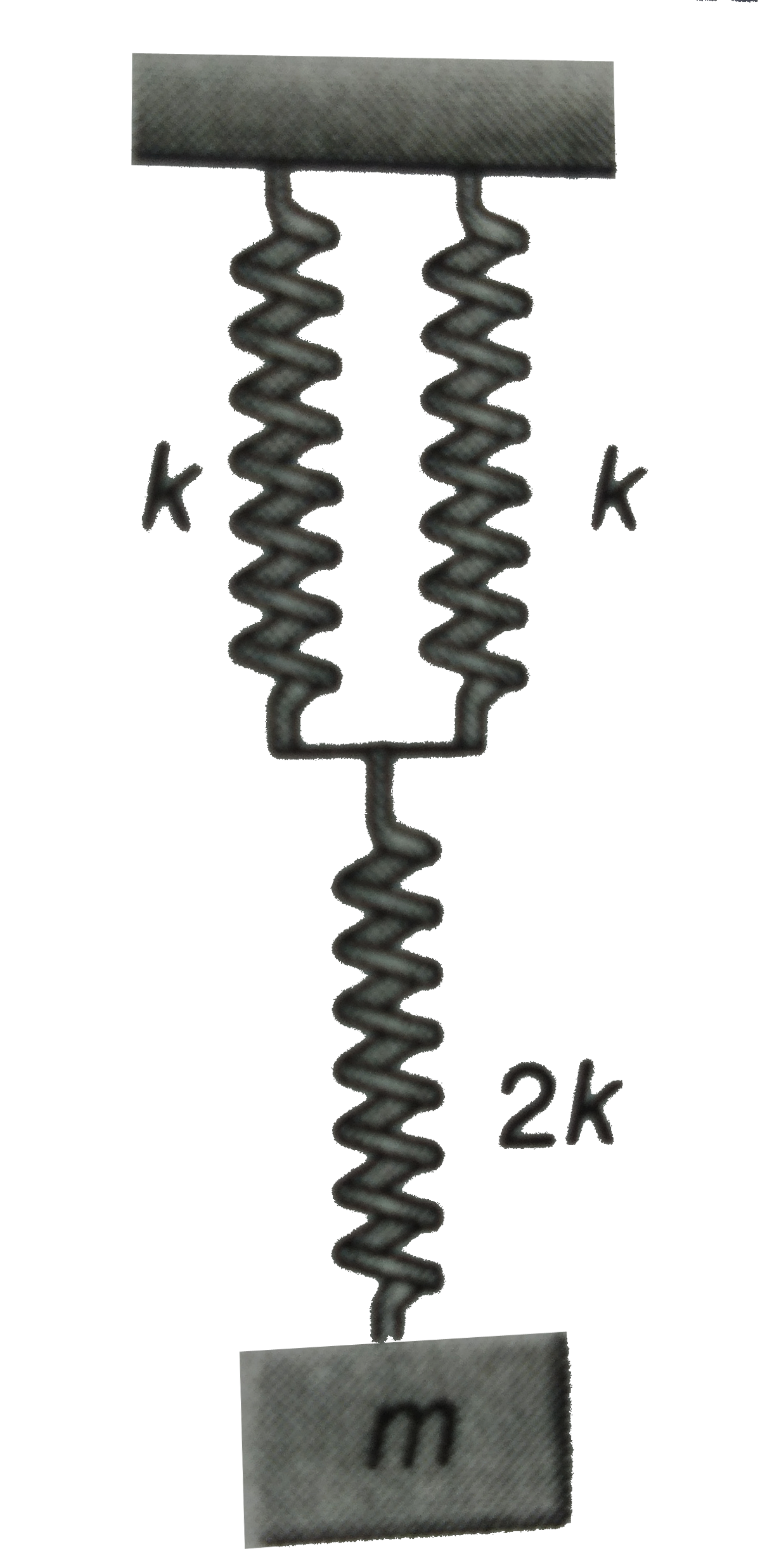
Three spring mass systems are shown in figure. Assuming gravity free spece, find the time period of oscillations in each case. What should be the answer if space is not gravity free ?
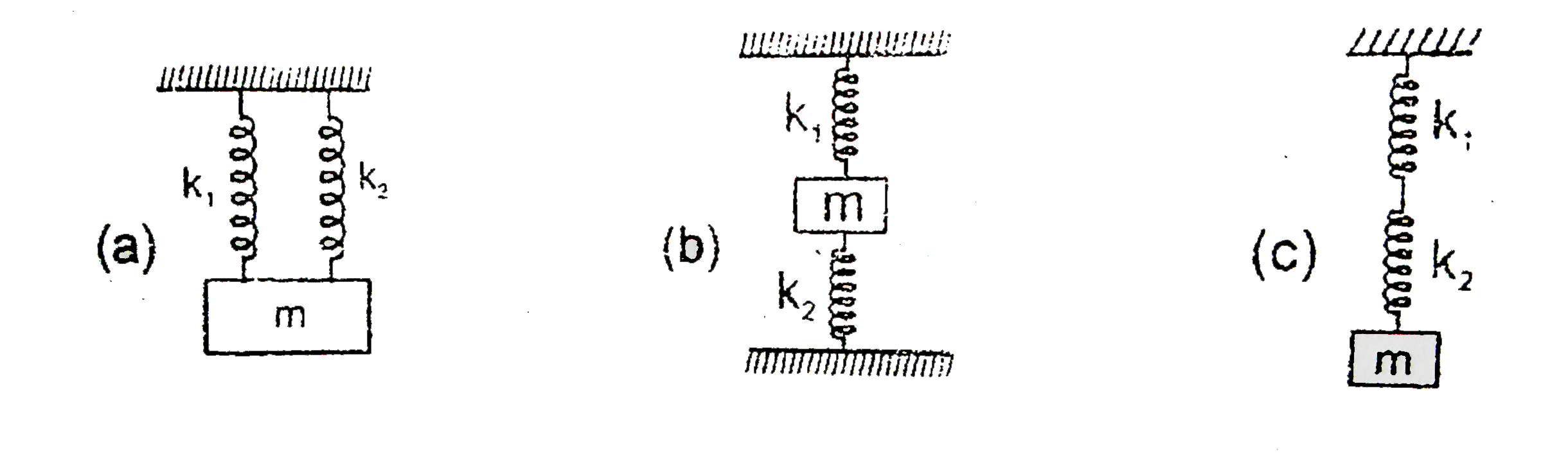
The correct Answer is:(a) 2π√mk1+k2,keq.=k1+k2;
2π√mk1+k2
keq.=k1+k2; (c) 2π√m(k1+k2)k1k2
keq.=k1k2k1+k2
(a) When spring is stretched by x then restoring force.
F=K1x+K2x
F=Keqx
Keqx=K1+K2x
Keq=K1+K2
T=2π√mKeq=2π√mK1+K2
(b) When bock is displaced by x from mean position then restoring force.
F=K1x+K2x
Keqx=K1x+K2x
Keq=K1+K2
T=2π√mKeq=2π√mK1+K2
(c) When block is displaced yx and extension is upper spring is x1, extension in lower spring is x2 then F=K1x1⇒x1=FK4
F=K2x2⇒x2=FK2
F=Keqx⇒x=FKeq
x=x1+x2⇒FKeq=FK1+FK2
Keq=K1K2K1+K2
T=2π√mKeq=2π√m(K1+K2)K1K2
When space in not gravity free than answer do not changes as time period of spring mass system is independent of gravity.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 1, PART - II
37 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 2, PART - I
26 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Board Level Exercise
24 Videos
- SEMICONDUCTORSBOOK - RESONANCECHAPTER - SEMICONDUCTORSEXERCISE - Exercise 3
88 Videos
- TEST PAPERSBOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - FST-3
30 Videos
Similar Questions
Knowledge Check
A system is shown in the figure. The force The time period for small oscilations of the two blocks will be
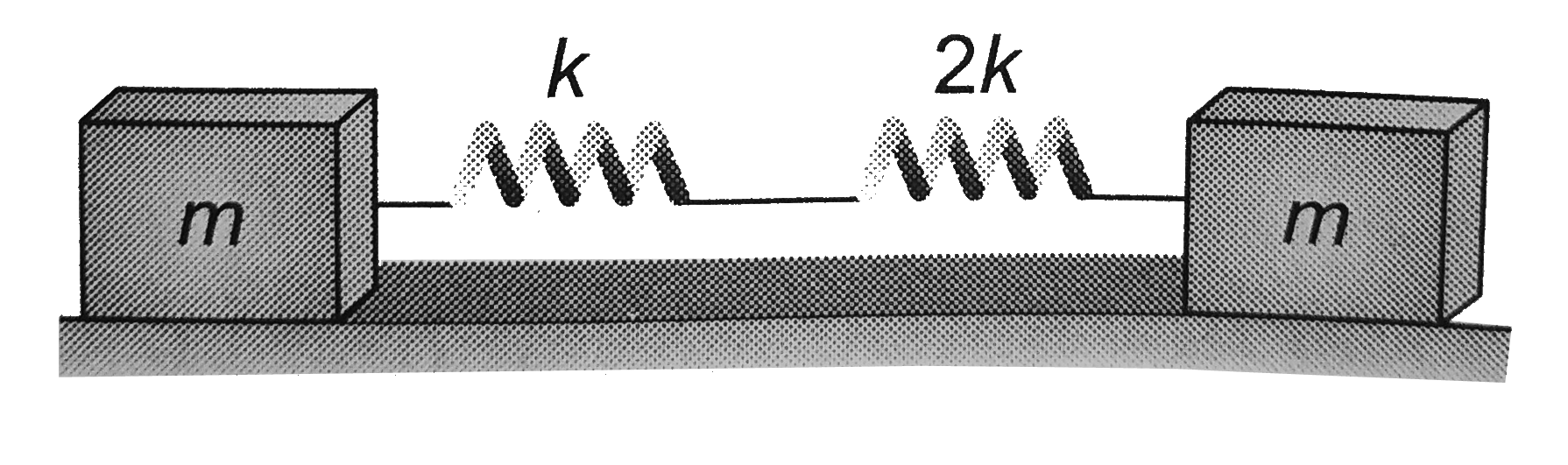 A2π√3mKB2π√3m2KC2π√3m4KD2π√3m8K
A2π√3mKB2π√3m2KC2π√3m4KD2π√3m8KThe two spring-mass system, shown in the figure, oscillates with a period T. If only one spring is used, the time period will be
 AT√2BT2C√2TD2T
AT√2BT2C√2TD2TThe two spring-mass system, shown in the figure, oscillates with a period T. If only one spring is used, the time period will be
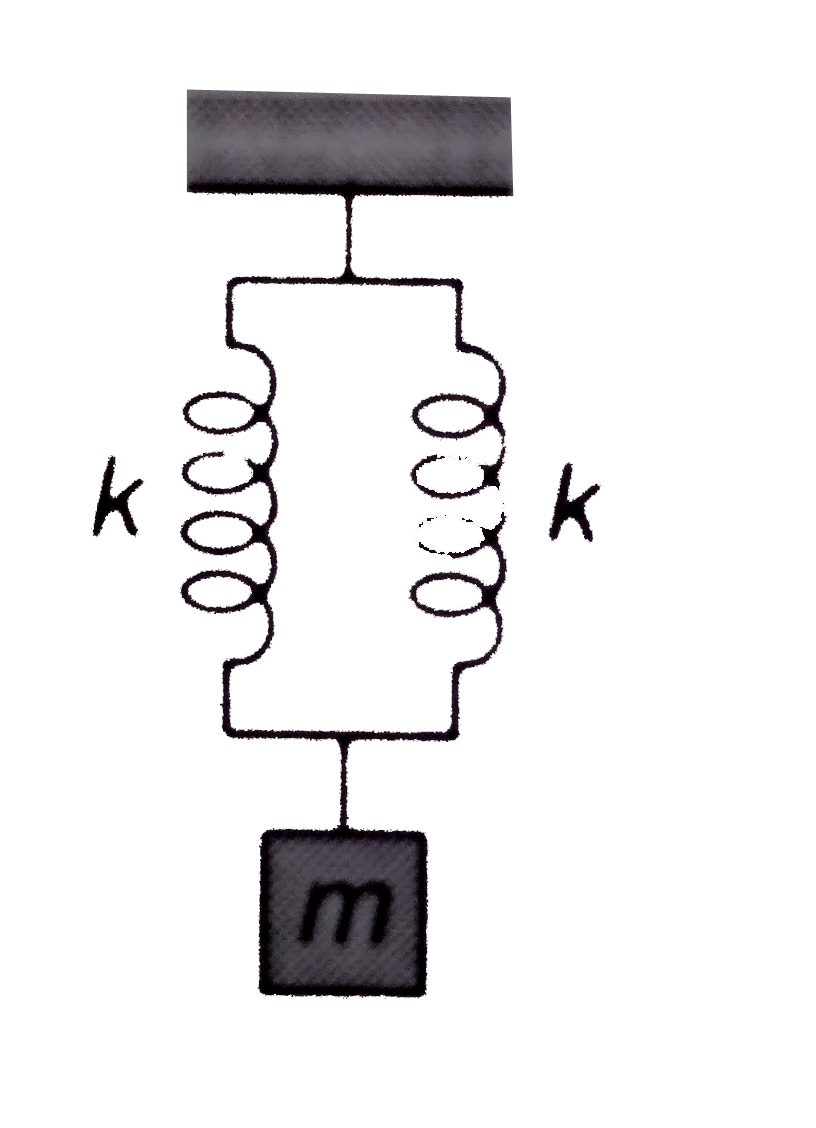 AT√2BT2C√2TD2T
AT√2BT2C√2TD2T
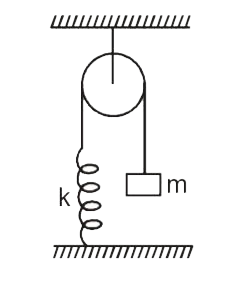
The string ,the spring and the pulley shown in figure are light. Find the time period of the mass m.
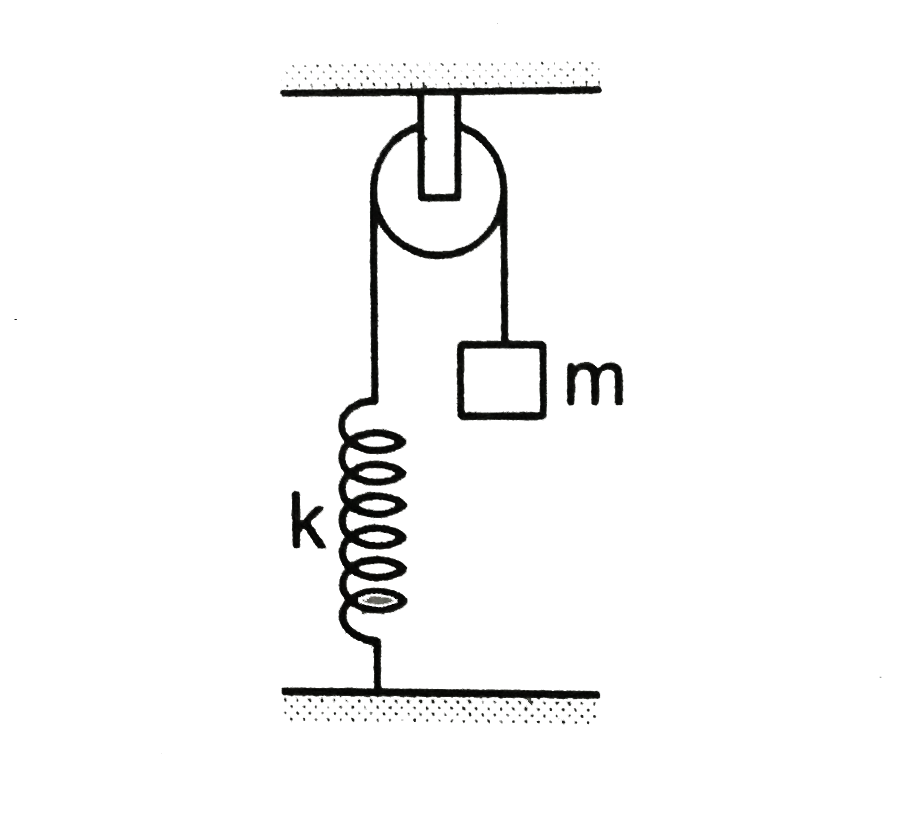
View SolutionThe string, spring and the pulley shown in figure are light. Find the time period of the mass m.
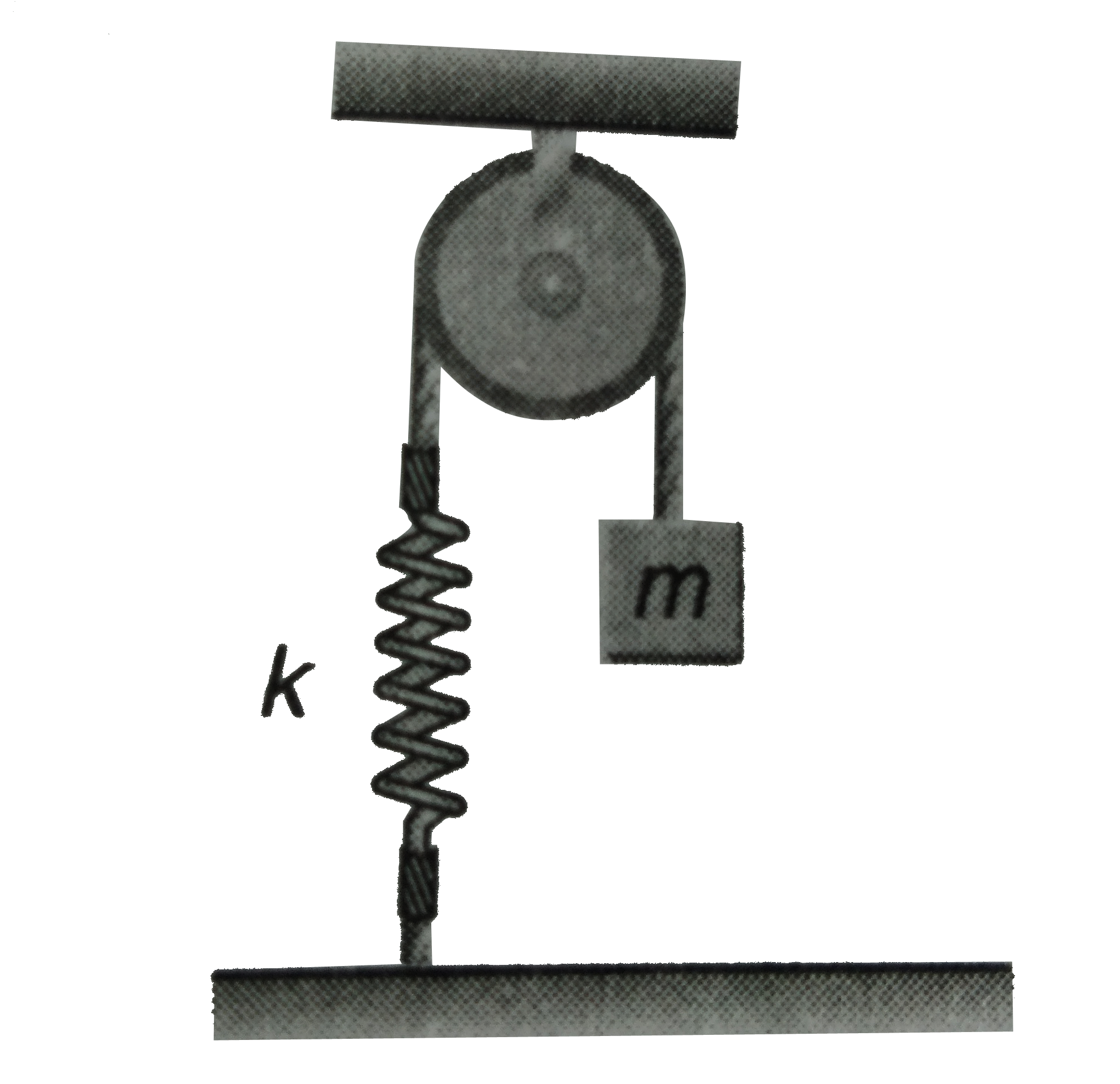
View SolutionA mass of 5 kg is connected to a spring. The potential energy curve of the simple harmonic motion executed by the system is shown in the figure. A simple pendulum of length 4 m has the same period of oscillation as the spring system. What is the value of acceleration due to gravity on the planet where these experiments are performed ?
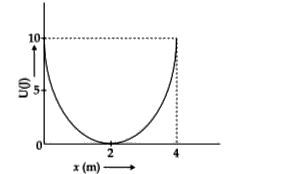
View SolutionA mass of 5 kg is connected to a spring. The potential energy curve of the simple harmonic motion executed by the system is shown in the figure. A simple pendulum of length 4 m has the same period of oscillation as the spring system. What is the value of acceleration due to gravity on the planet where these experiments are performed ?
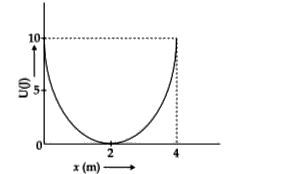
View SolutionA body of mass m is suspended from three springs as shown in figure. If mass m is displaced slightly then time period of oscillation is

View Solution