Distance between the centres of two rings is a. The masses of these rings are M and 4M. A body of mass m is fired straight from the centre of the heavier ring. What should be its minimum initial speed to cross of lighter ring ? The radius of the both ring is R. (Assume a>>R).

The correct Answer is:√8GMR
Step by step video, text & image solution for Distance between the centres of two rings is a. The masses of these rings are M and 4M. A body of mass m is fired straight from the centre of the heavier ring. What should be its minimum initial speed to cross of lighter ring ? The radius of the both ring is R. (Assume a gt gt R). by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- GRAVITATIONBOOK - FIITJEECHAPTER - GRAVITATIONEXERCISE - Assigment problems (Objective) (level-I)
50 Videos
- GRAVITATIONBOOK - FIITJEECHAPTER - GRAVITATIONEXERCISE - Assigment problems (Objective) (level-I) Assertion & Reasoning type
2 Videos
- GRAVITATIONBOOK - FIITJEECHAPTER - GRAVITATIONEXERCISE - Assigment problems (Subjective) (level I)
14 Videos
- GMP ASSESMENTBOOK - FIITJEECHAPTER - GMP ASSESMENTEXERCISE - Numerical Based
61 Videos
- HEAT AND TEMPERATUREBOOK - FIITJEECHAPTER - HEAT AND TEMPERATUREEXERCISE - NUMERICAL BASES QUESTIONS
1 Videos
Similar Questions
Consider a nebula in the form of a ring of radius R and mass M. A star of mass m(mR from the centre of the ring on its axis, initially at rest.The speed with which it crosses the centre of the ring is y=√(p−√q)GMR . Find (p+q)
View SolutionA particle of mass m is kept on the axis of a fixed circular ring of mass M and radius R at a distance x from the centre of the ring. Find the maximum gravitational force between the ring and the particle.
View Solution
Knowledge Check
The escape velocity form the centre of a unifrom ring of mass M and radius R is :
A√2GMRB√GMRC√GM2RD√GMRThe total energy of rolling ring of mass m and radius R is
A3/2mv2B1/2mv2Cmv2D5/2mv2A particle of mass m is placed at a distance x from the centre of ring along the line through the centre of the ring and perpendicular to its plane.
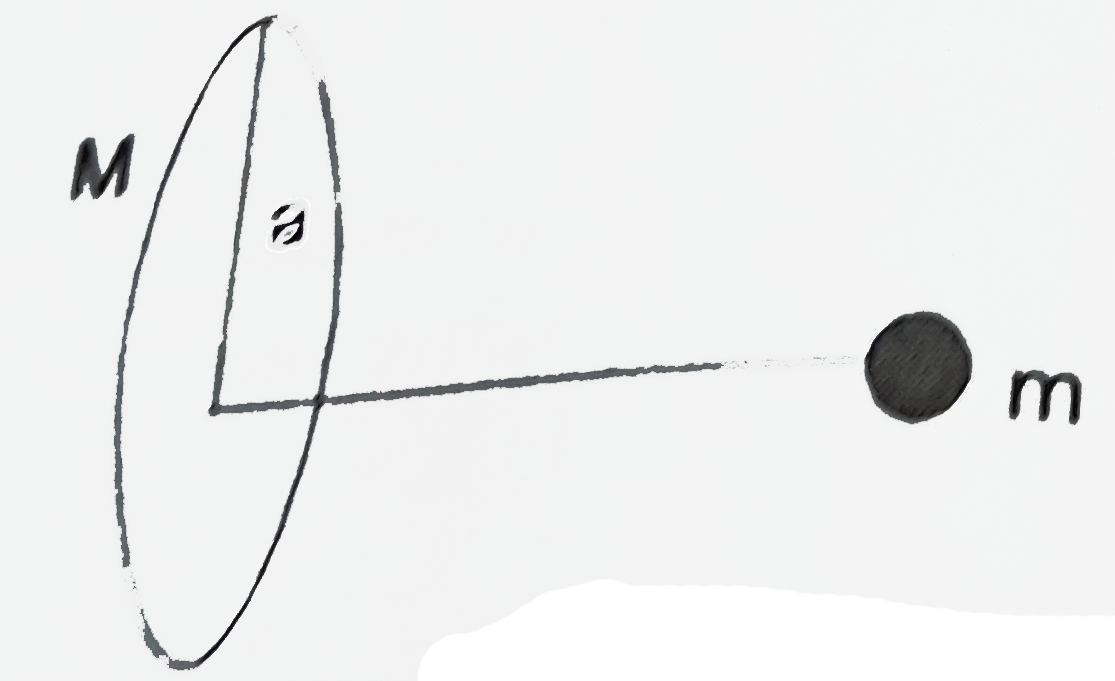
The force, when x=0 :AGMmx2BGMmx√a2+x2CGMmx(a2+x2)3/2DZero
Find the centre of mass of a uniform semicircular ring of radius R and mass M.
View SolutionFrom a complete ring of mass M and radius R, a 30∘sector is removed. The moment of inertia of the incomplete ring about an axis passing through the centre of the ring and perpendicular to the plane of the ring is
 ,
,
View SolutionWhat is the distance of centre of mass of a half fring from centre if the ring has radius =0.5 m [XI]
View SolutionA ring has a total mass M but non-uniformly distributed over its circumference. The radius of the ring is R. A point mass m is placed at the centre of the ring. Workdone in taking away this point mass from ecntre to infinity is
View SolutionThe centres of a ring of mass m and a sphere of mass M of equal radius R, are at a distance √8R apart as shown. The force of attraction between the ring and the sphere is
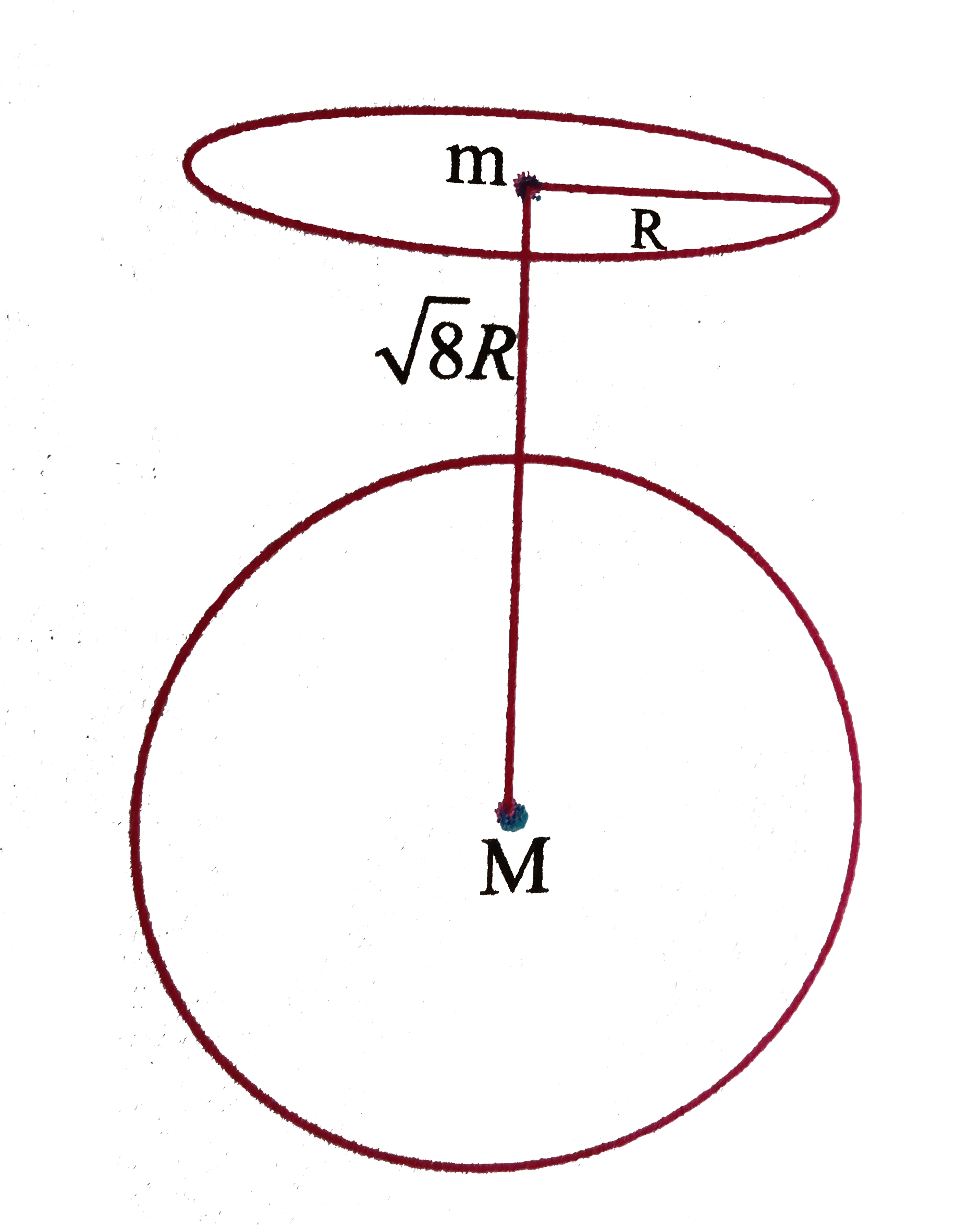
View Solution
