If a,b,c are the sides of a right triangle , where c is the hypotenuse. Prove that the radius r of the circle which touches the sides of the triangle is given by: $r = \frac{a + b - c}{2}$

Video Solution

Text Solution

Verified by Experts

Since $O E ⊥ A C$ and $O D ⊥ B C$
(radius through point of contact is $⊥$ to the tangent)
$∴ □ O D C E$ is a square of side r.
$∴ B D = a - r$ and $A E = b - r$
Now, BC, AC and AB are the tangents of a circle.
$∴ B C = B D$
(length of tangents from an external point are equal)
$= a - r$
and $A C = A E$ (length of rangents from an external point are equal)
$= b - r$
Now $C = A B = B C + A C$
$\Rightarrow C = a - r + b - r \Rightarrow 2 r = a + b - c \Rightarrow r = \frac{a + b - c}{2}$
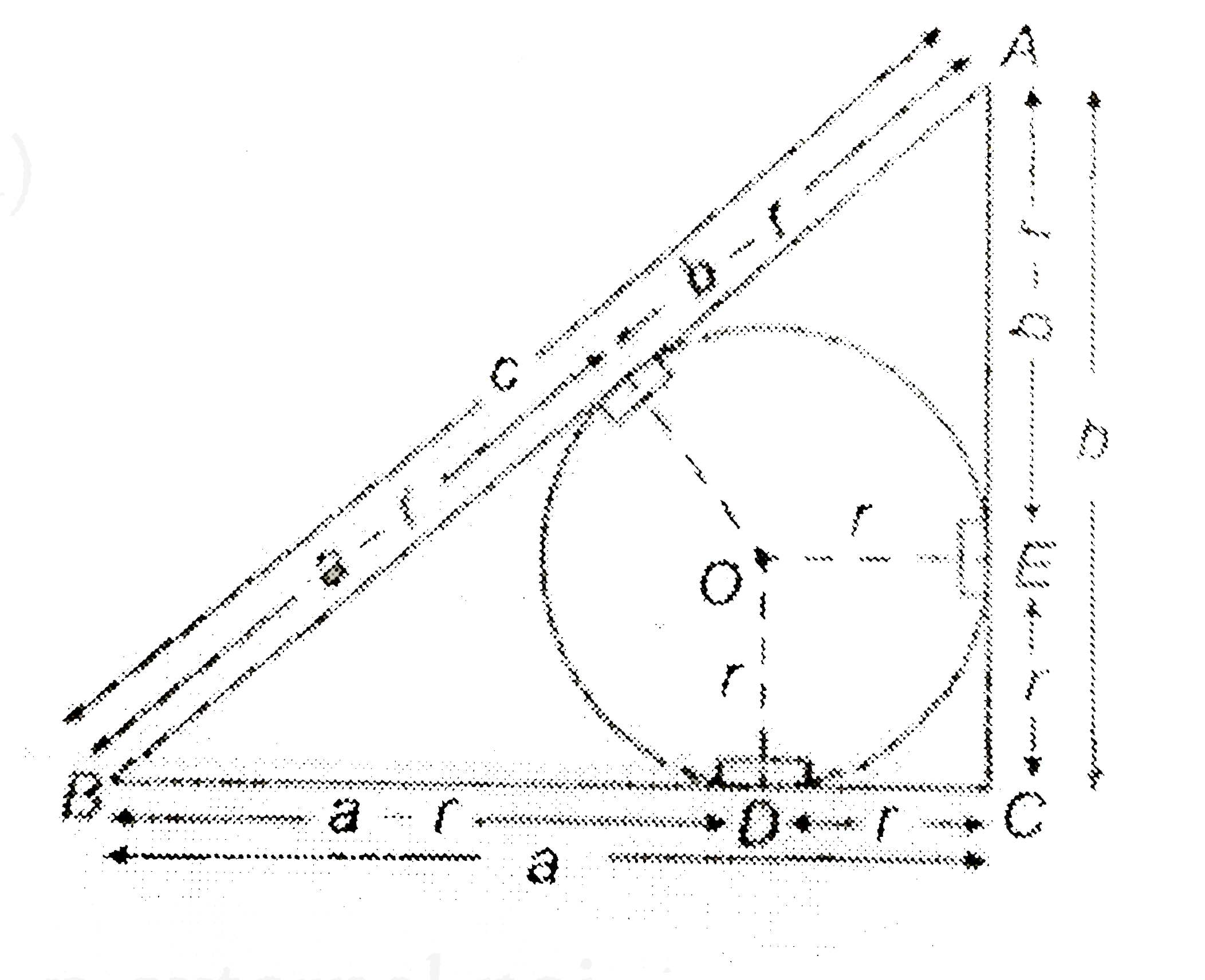Hence Proved.

Updated on:21/07/2023

The sides of a right triangle ABC are a, b and c, where c is the hypotenuse. What will be the radius of the in circle of this triangle?
A $\frac{(a + b + c)}{2}$
B $\frac{(a + b - c)}{2}$
C $\frac{(b + c - a)}{2}$
D $\frac{(a + c - b)}{2}$
If a, b,c are the lengths of the sides of triangle , then
A $a - b > c$
B $a + b < c$
C $c = a + b$
D $c < a + b$
If a,b, c are sides of a triangle and $a^{2} + b^{2} = c^{2},$ name the type of triangle.
AObtuse angled triangle
BAcute angled triangle
CRight angled triangle
DEquilateral triangle