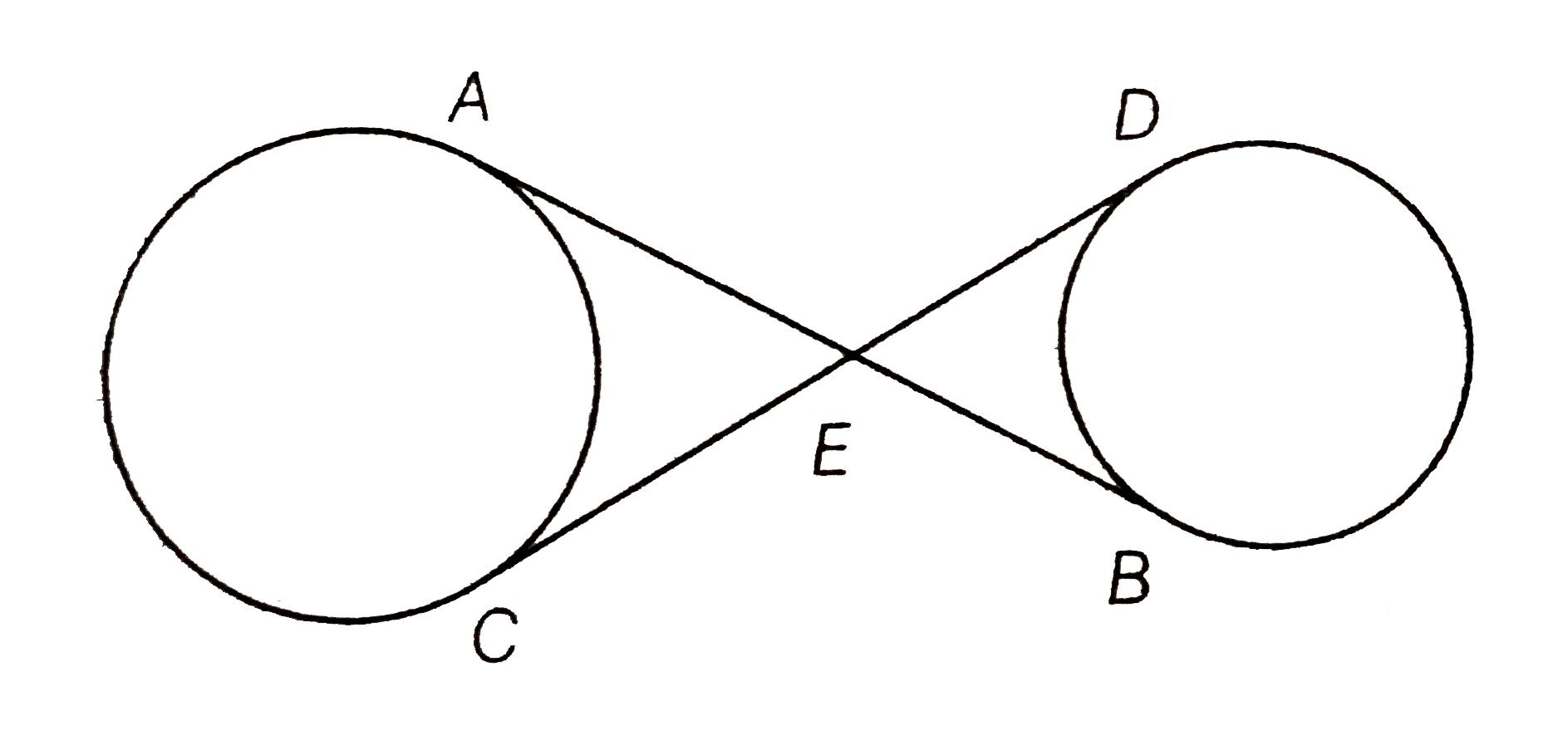
In the adjoining figure, common tangents AB and CD to two circles intersect at P.
Prove that AB=CD.

Since PA and PC are two tangents to a circle with centre O from an external point P.
∴ PA=PC …(1)
Also, since PB and PD are two tengents to a circle with centre O' from an external point P.
Circles
∴ PB=PD
Adding (2) and (1), we get
PA+PB=PC+PD
⇒ AB=CD (∵ AB and CD are two straight lines) Hence Proved.
Topper's Solved these Questions
- CIRCLESBOOK - NAGEEN PRAKASHANCHAPTER - CIRCLESEXERCISE - Problems From NCERT/exemplar
11 Videos
- CIRCLESBOOK - NAGEEN PRAKASHANCHAPTER - CIRCLESEXERCISE - Exercise
25 Videos
- ARITHMETIC PROGRESSIONBOOK - NAGEEN PRAKASHANCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Revision Exercise Long Answer Question
5 Videos
- CO-ORDINATE GEOMETRYBOOK - NAGEEN PRAKASHANCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Revision Exercise Short Answer Questions
9 Videos
Similar Questions
Knowledge Check
In the adjoining figure, if chords AB and CD of the circle intesect each other at right angles, then find the value of x+y.
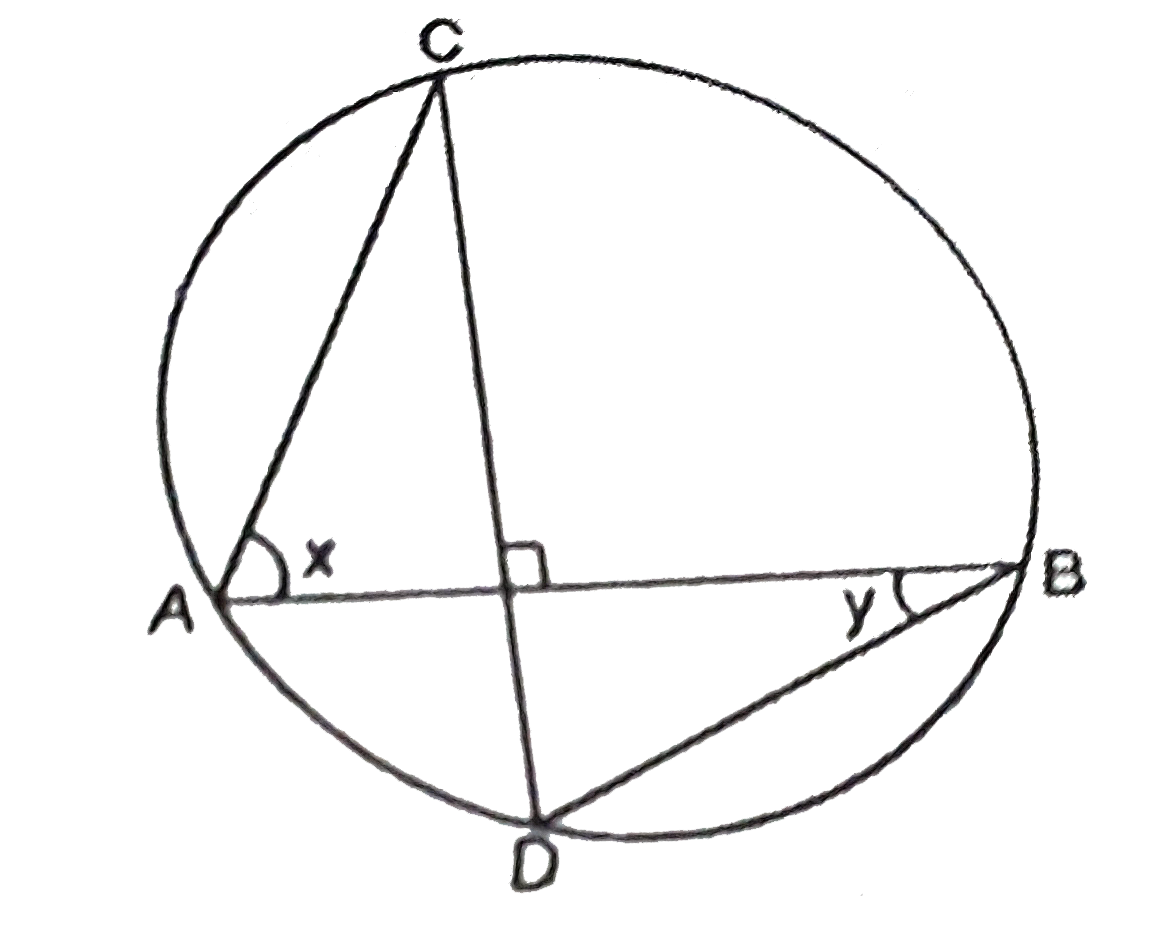 A45∘B90∘C120∘D100∘
A45∘B90∘C120∘D100∘
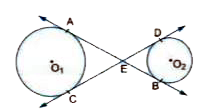
In Fig common tangents AB and CD to two circles with centres O1andO2 intersect at E. Prove that AB = CD.
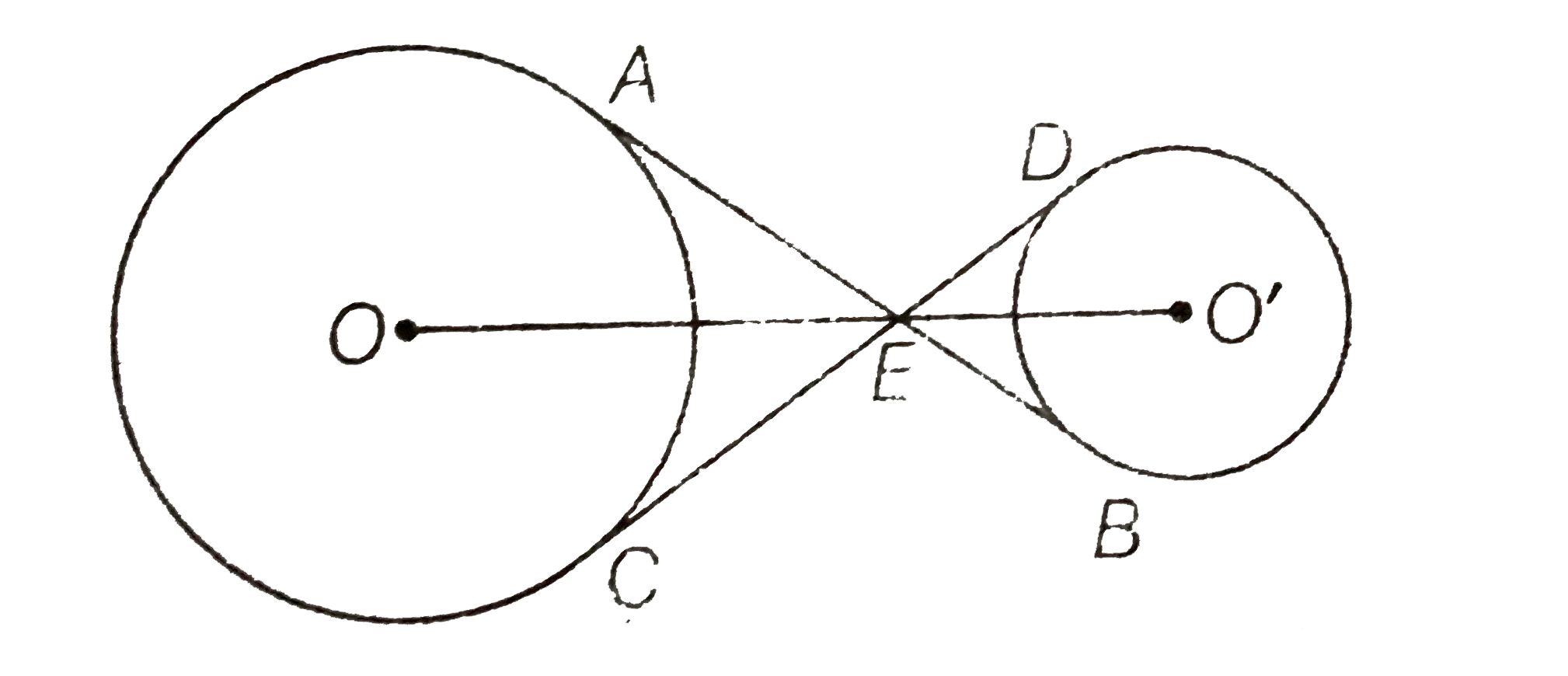
View SolutionIn a figure the common tangents, AB and CD to two circles with centers O and O' intersect at E. Prove that the points O, E and O' are collinear.
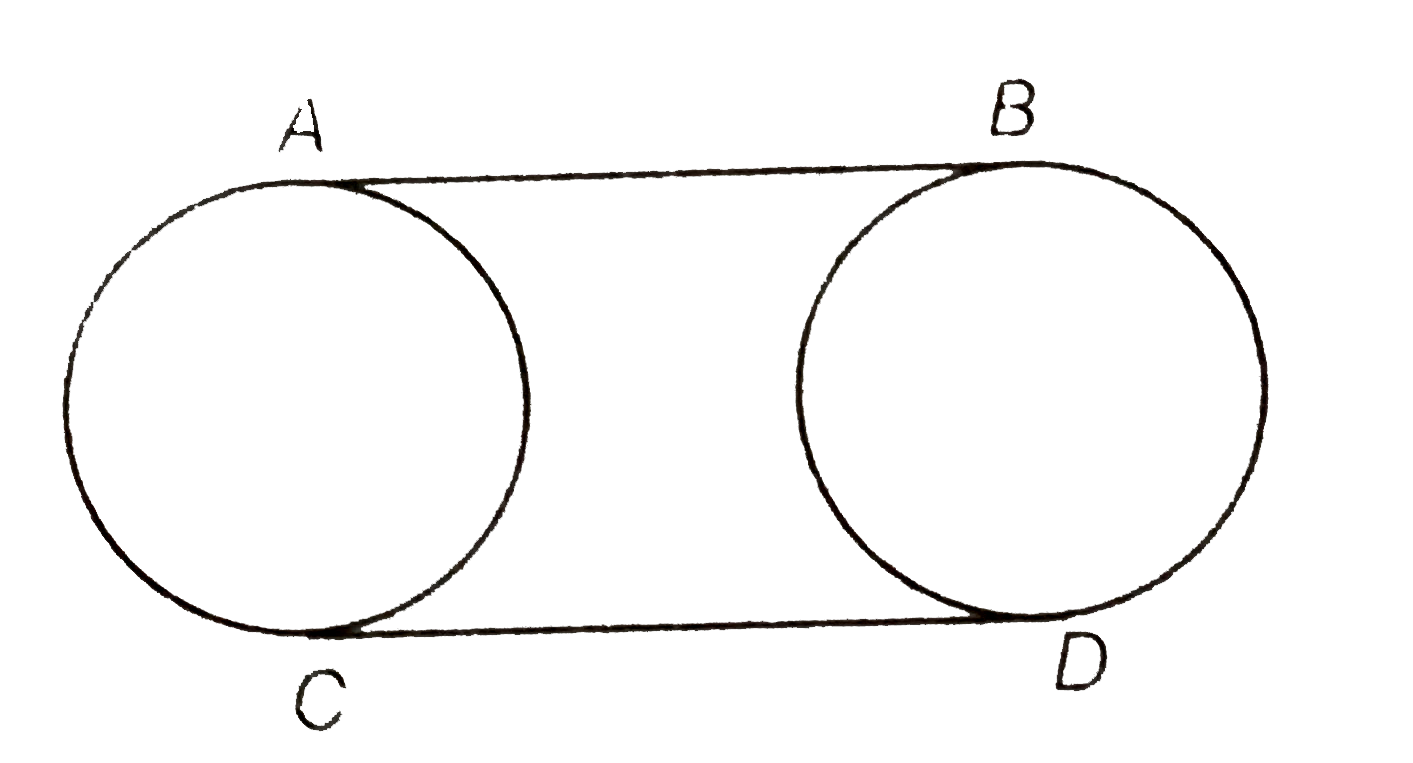
View SolutionIn figure, AB and CD are common tangents to two circles of equal radii. Prove that AB=CD.
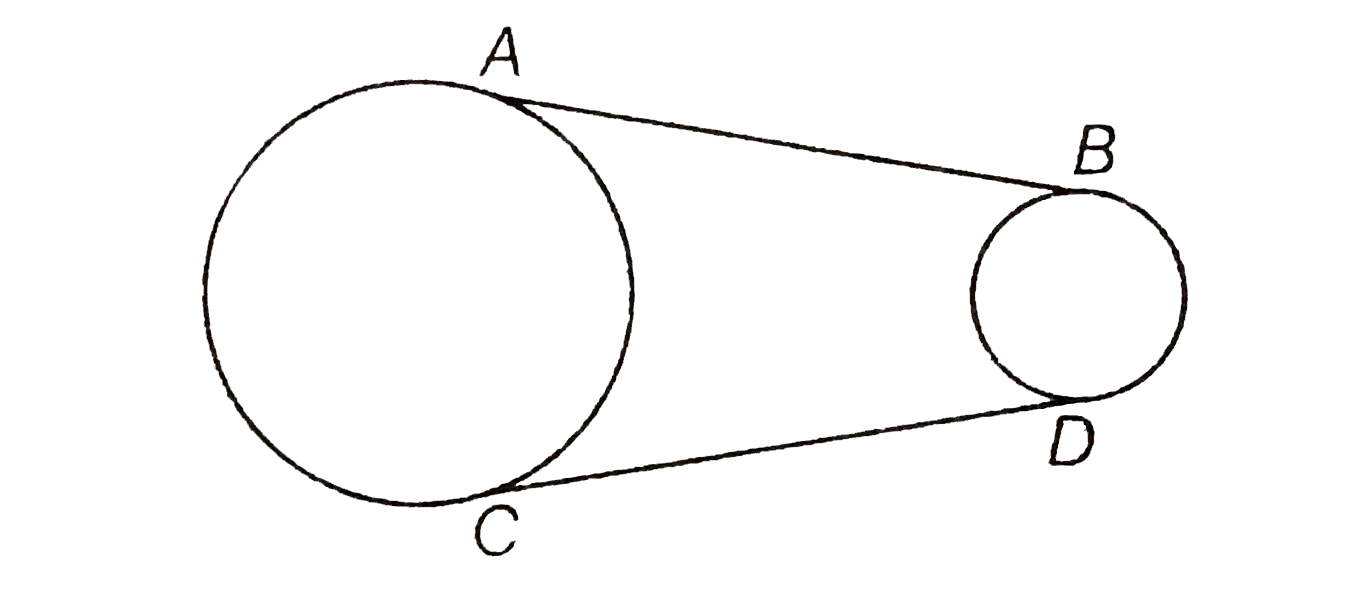
View SolutionIn figure, AB and CD are common tangents to two circles of unequal radii. Prove that AB=CD
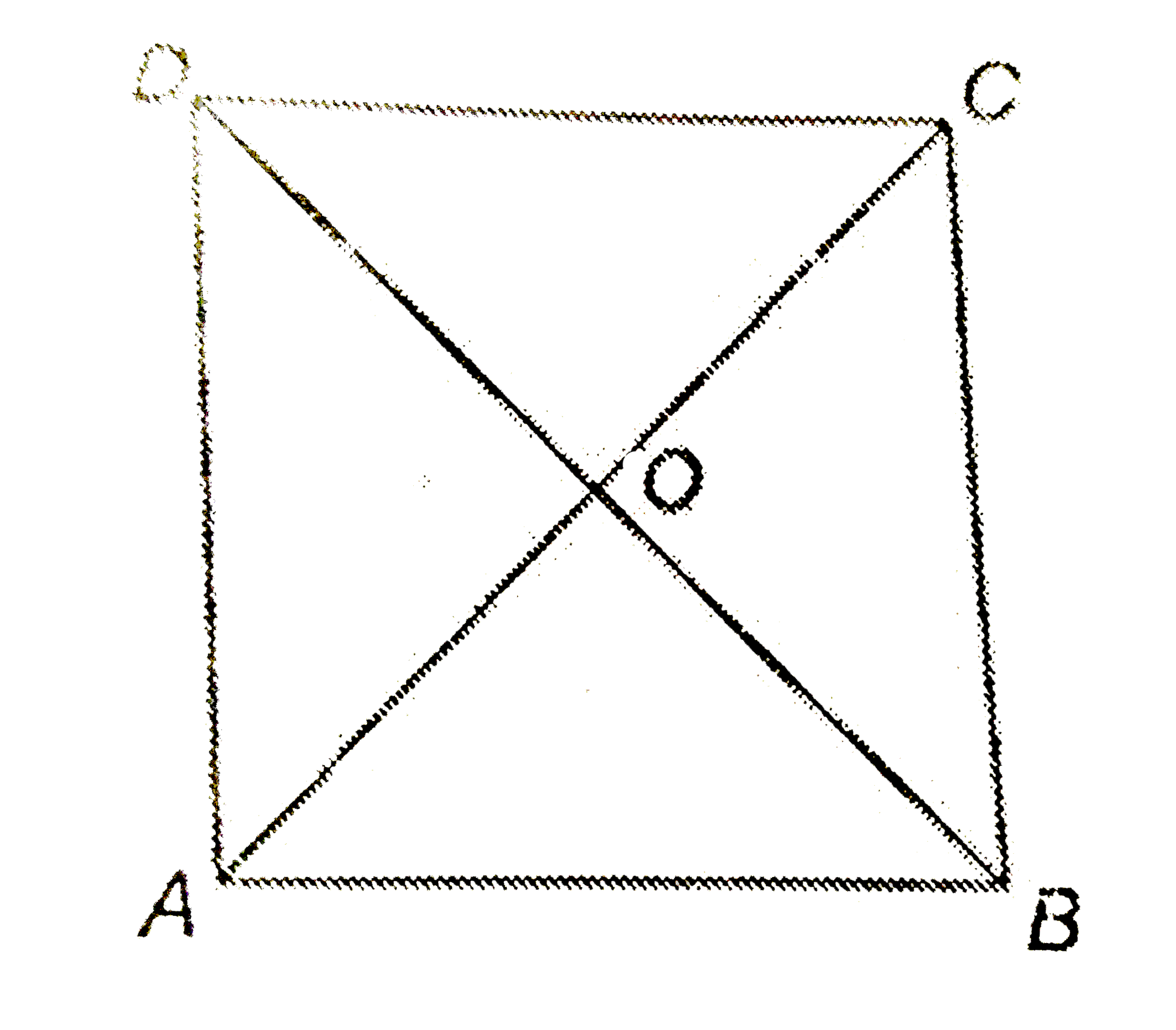
View SolutionIn the adjoining figure, the diagonals AC and BD of a quadrilateral ABCD intersect point O. Prove that :
AB+BC+CD+DA<2(AC+BD)
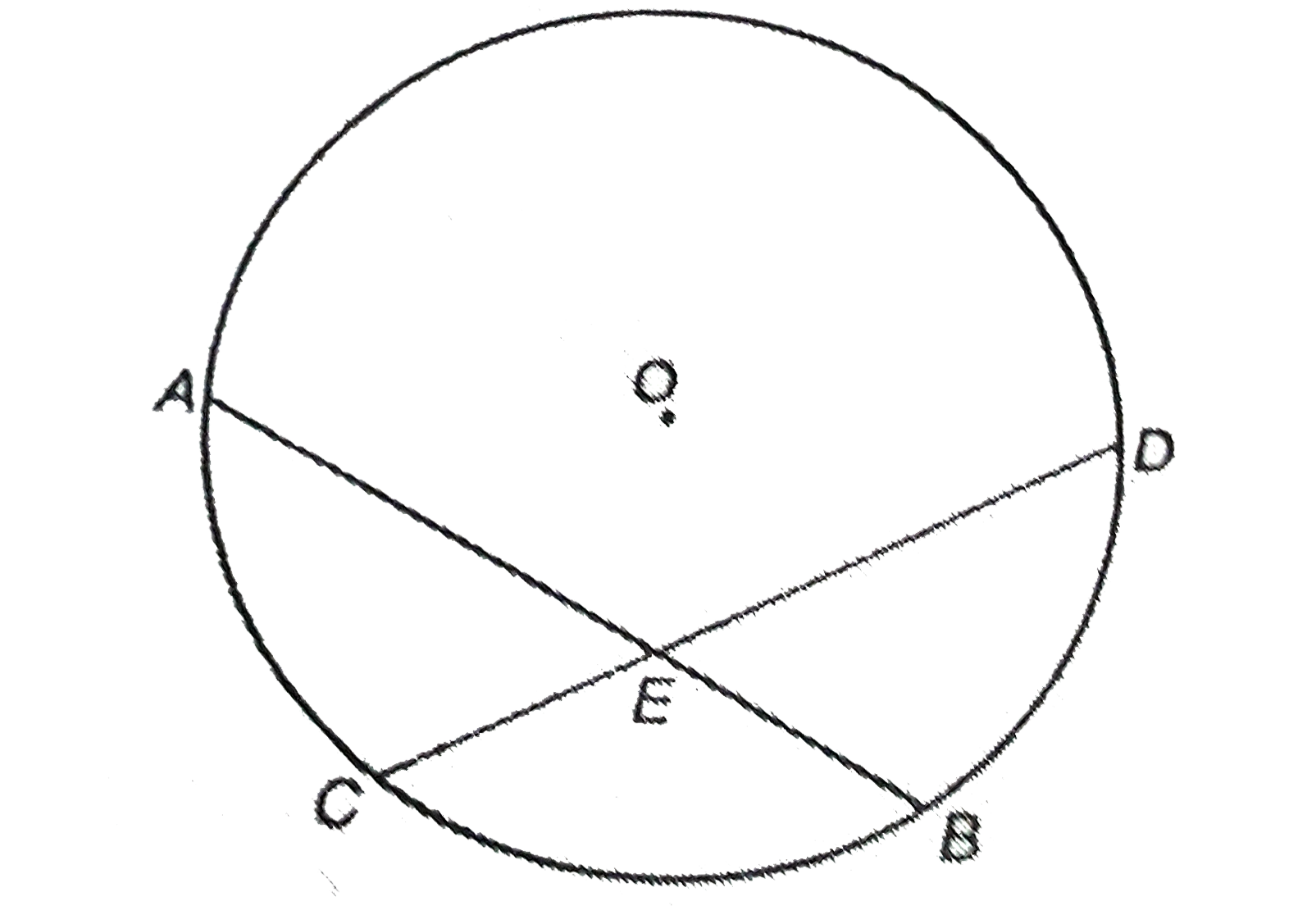
View SolutionIn the adjoining figure. If two equal chords AB and CD of a circle intersect each other at E. then prove that chords AC and DB are equal.
View SolutionIn the adjoining figure AB||CD, find x and y

View Solution