A ring of mass m and radius R is given a charge q. It is then rotated about its axis with angular velocity ω.Find
(i)Current produced due to motion of ring
To find the current produced due to the motion of a rotating charged ring, we can follow these steps:
1. Understanding the Problem:
- We have a ring with mass m, radius R, and charge q that is rotating about its axis with an angular velocity ω.
2. Determine the Time Period of Rotation:
- The time period T of one complete rotation can be calculated using the formula:
T=2πω
- Here, ω is the angular velocity.
3. Calculate the Current:
- Current I is defined as the charge passing through a point in the circuit per unit time. Therefore, we can express current as:
I=qT
- Substituting the expression for T from step 2 into the equation for current gives:
I=q2πω=q⋅ω2π
4. Final Expression for Current:
- Thus, the current produced due to the motion of the ring is:
I=qω2π
Summary:
The current produced due to the motion of the rotating charged ring is given by:
I=qω2π
- We have a ring with mass m, radius R, and charge q that is rotating about its axis with an angular velocity ω.
- The time period T of one complete rotation can be calculated using the formula:
T=2πω
- Here, ω is the angular velocity.
- Current I is defined as the charge passing through a point in the circuit per unit time. Therefore, we can express current as:
I=qT
- Substituting the expression for T from step 2 into the equation for current gives:
I=q2πω=q⋅ω2π
- Thus, the current produced due to the motion of the ring is:
I=qω2π
The current produced due to the motion of the rotating charged ring is given by:
I=qω2π
Similar Questions
A ring of mass m and radius R is given a charge q. It is then rotated about its axis with angular velocity ω.Find
(iii)Magnetic moment of ring.
View SolutionA ring of mass m and radius R is given a charge q. It is then rotated about its axis with angular velocity ω.Find
(ii) Magnetic field produced at the centre of ring.
View Solution
Knowledge Check
A metallic ring of radius r with a uniform metallic spoke of negligible mass and length r is rotated about its axis with angular velocity ω in a perpendicular uniform magnetic field B as shown in Fig. 3.163. The central end of the spoke is connected to the rim of the wheel through a resistor R as shown. The resistor does not rotate, its one end is always at the center of the ring and the other end is always in as shown is needed to maintain constant angular velocity of the wheel. F is equal to (the ring and the spoke has zero resistance)
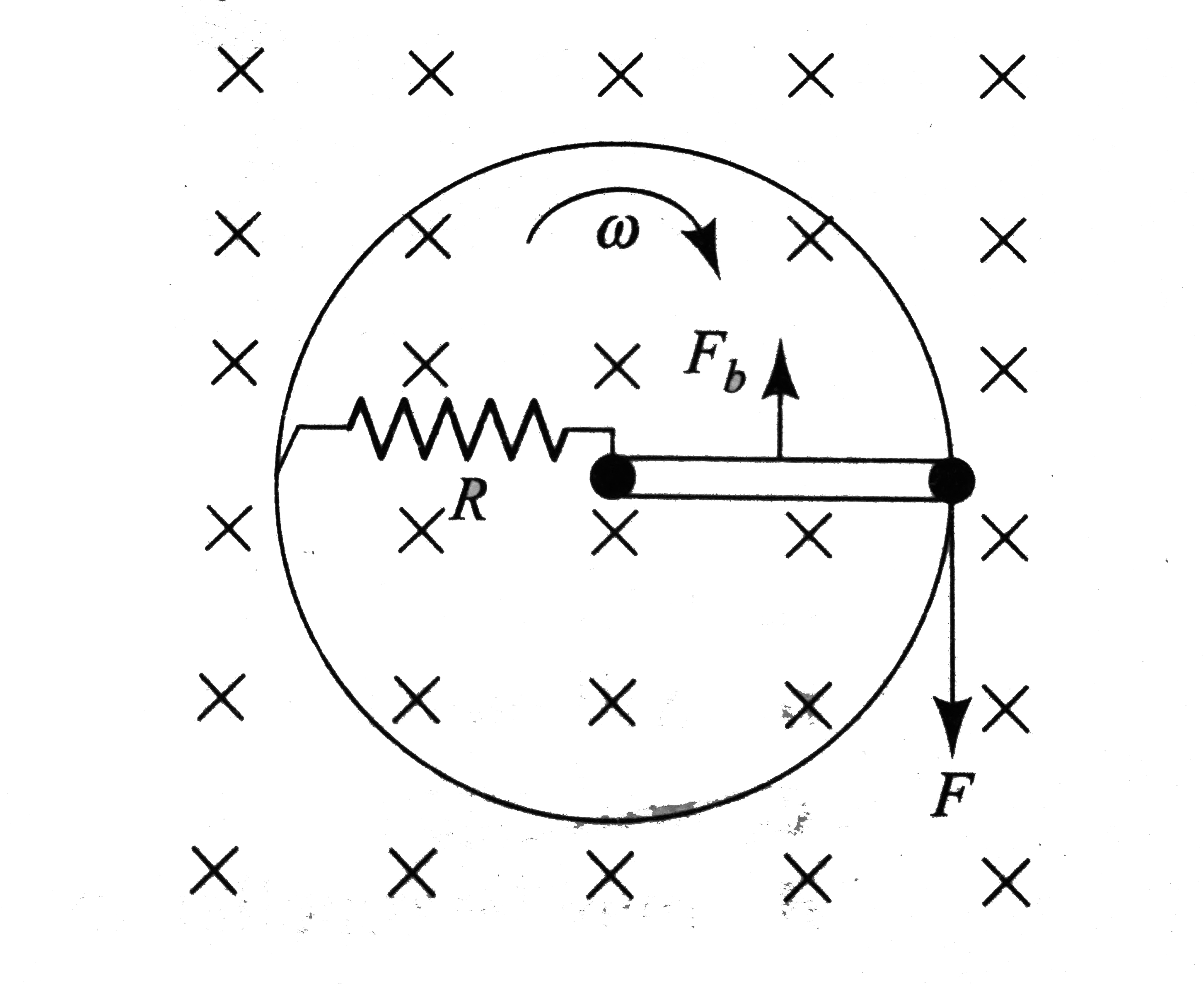 AB2ωr28RBB2ωr22RCB2ωr32RDB2ωr34R
AB2ωr28RBB2ωr22RCB2ωr32RDB2ωr34RA string has a linear mass density μ. The ends of the string are joined to form a closed loop and is given the shape of a circular ring of radius R. this ring is now rotated about its axis with an angular velocity ω
Q. The tension developed in the string isAμωR3B2μω2R2Cμω2R2Dμ2ω2R2A thin circular ring of mass M and radius R is rotating about its axis with constant angular velocity ω. The objects each of mass m are attached gently to the ring. The wheel now rotates with an angular velocity.
AωM(m+M)Bω(M−2M)(M+2m)CωM(M+2m)Dω(M+2m)M
A ring of mass m and radius R is being rotated about its axis with angular velocity ω. If a increases then tension in ring
View SolutionA ring of mass m and radius R is being rotated about its axis with constant angular velocity ω in the gravity free space. Find tension in the ring.
View SolutionA ring of mass M and radius R is rotating about its axis with angular velocity ω. Two identical bodies each of mass m are now gently attached at the two ends of a diameter of the ring. Because of this, the kinetic energy loss will be :
View SolutionA thin uniformring of radius R carrying uniform charge Q and mass M rotates about its axis with angular velocity ω. The ratio of its magnetic moment and angular momentum is:
View SolutionA thin circular ring of mass M and radius R is rotating about its axis with a constant angular velocity omega. Four objects each of mass m, are kept gently to the opposite ends of two perpendicular diameters of the ring. The angular velocity of the ring will be
View Solution