A particle move with velocity $v_{1}$ for time $t_{1} and v_{2}$ for time $t_{2}$ along a straight line. The magntidue of its average acceleration is

A

$\frac{v_{2} - v_{1}}{t_{1} - t_{2}}$

B

$\frac{v_{2} - v_{1}}{t_{1} + t_{2}}$

C

$\frac{v_{2} - t_{1}}{t_{2} - t_{1}}$

D

$\frac{v_{1} + v_{2}}{t_{1} - t_{2}}$

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:B

To find the magnitude of the average acceleration of a particle that moves with different velocities over different time intervals, we can follow these steps:
1. Understand the Concept of Average Acceleration:
Average acceleration is defined as the total change in velocity divided by the total time taken. Mathematically, it can be expressed as:
$a_{a v g} = \frac{Δ V}{Δ t}$
where $Δ V$ is the change in velocity and $Δ t$ is the total time.
2. Identify the Given Values:
- Initial velocity $v_{1}$ during time $t_{1}$ .
- Final velocity $v_{2}$ during time $t_{2}$ .
3. Calculate the Change in Velocity:
The change in velocity ( $Δ V$ ) can be calculated as:
$Δ V = v_{2} - v_{1}$
4. Calculate the Total Time:
The total time ( $Δ t$ ) during which the particle is in motion is the sum of the two time intervals:
$Δ t = t_{1} + t_{2}$
5. Substitute the Values into the Average Acceleration Formula:
Now substitute $Δ V$ and $Δ t$ into the average acceleration formula:
$a_{a v g} = \frac{v_{2} - v_{1}}{t_{1} + t_{2}}$
6. Determine the Magnitude of Average Acceleration:
Since acceleration is a vector quantity, we take the magnitude:
$| a_{a v g} | = ∣ ∣ ∣ \frac{v_{2} - v_{1}}{t_{1} + t_{2}} ∣ ∣ ∣$
Final Answer:
The magnitude of the average acceleration is:
$| a_{a v g} | = ∣ ∣ ∣ \frac{v_{2} - v_{1}}{t_{1} + t_{2}} ∣ ∣ ∣$

Updated on:18/9/2024

|

Class 12 PHYSICS MOTION IN A STRAIGHT LINE

Topper's Solved these Questions

Similar Questions

Knowledge Check

Acceleration time graph of a particle moving along a straight line is given. Then average acceleration between $t = 0$ & $t = 4$ is $: -$

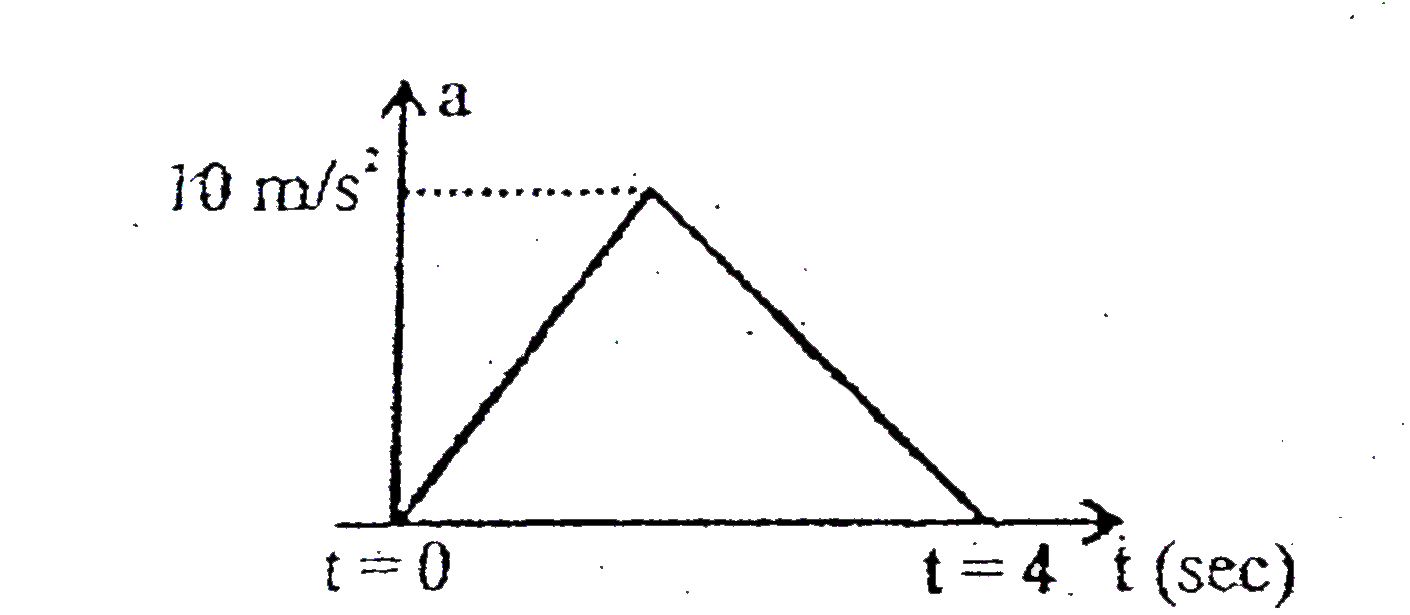A $0 m / s^{2}$
B $5 m / s^{2}$
C $10 m / s^{2}$
D $7.5 m / s^{2}$
A particle moves from A to B with a uniform velocity $v_{1}$ and from B to A with a velocity $v_{2}$ . If it moves along a straight line between A and B, then the average velocity of the particle is
A $\frac{v_{1} v_{2}}{2}$
B $\frac{v_{1} + v_{2}}{2}$
C $\frac{2 v_{1} v_{2}}{v_{1} + v_{2}}$
Dzero
If a particle is moving the velocity v(t)=cos $π$ t, along a straight line such that at t=0, s=4, its position function is given by
A $\frac{1}{π} cos π t + 2$
B $- \frac{1}{π} sin π t + 4$
C $\frac{1}{π} sin π t + 4$
Dnone of these

AAKASH INSTITUTE-MOTION IN A STRAIGHT LINE-ASSIGNMENT (SECTION - A)

Home
Ask Doubt
Profile