Two balls are projected upward simultaneously with speeds 40 m/s and 60 m/s. Relative position (x) of second ball w.r.t. first ball at time t = 5 s is [Neglect air resistance].
20 m
80 m
100 m
120 m
The correct Answer is:C
To solve the problem of finding the relative position of the second ball with respect to the first ball at time t=5 seconds, we can follow these steps:
Step 1: Understand the motion of the balls
Both balls are projected upward with initial speeds:
- Ball 1 (first ball): u1=40m/s
- Ball 2 (second ball): u2=60m/s
Both balls experience the same downward acceleration due to gravity g=9.81m/s2.
Step 2: Calculate the position of each ball at t=5 seconds
The position of an object under uniform acceleration can be calculated using the equation:
s=ut+12at2
where:
- s is the displacement,
- u is the initial velocity,
- a is the acceleration (negative in this case, since it's downward),
- t is the time.
For Ball 1:
s1=u1t−12gt2
Substituting the values:
s1=40×5−12×9.81×(5)2
s1=200−12×9.81×25
s1=200−122.625
s1=77.375m
For Ball 2:
s2=u2t−12gt2
Substituting the values:
s2=60×5−12×9.81×(5)2
s2=300−12×9.81×25
s2=300−122.625
s2=177.375m
Step 3: Calculate the relative position
The relative position x of the second ball with respect to the first ball is given by:
x=s2−s1
Substituting the values:
x=177.375−77.375
x=100m
Final Answer
The relative position of the second ball with respect to the first ball at t=5 seconds is 100 m.
---
Both balls are projected upward with initial speeds:
- Ball 1 (first ball): u1=40m/s
- Ball 2 (second ball): u2=60m/s
The position of an object under uniform acceleration can be calculated using the equation:
s=ut+12at2
where:
- s is the displacement,
- u is the initial velocity,
- a is the acceleration (negative in this case, since it's downward),
- t is the time.
s1=u1t−12gt2
Substituting the values:
s1=40×5−12×9.81×(5)2
s1=200−12×9.81×25
s1=200−122.625
s1=77.375m
s2=u2t−12gt2
Substituting the values:
s2=60×5−12×9.81×(5)2
s2=300−12×9.81×25
s2=300−122.625
s2=177.375m
The relative position x of the second ball with respect to the first ball is given by:
x=s2−s1
Substituting the values:
x=177.375−77.375
x=100m
The relative position of the second ball with respect to the first ball at t=5 seconds is 100 m.
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINEBOOK - AAKASH INSTITUTECHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ASSIGNMENT (SECTION - B)
33 Videos
- MOTION IN A STRAIGHT LINEBOOK - AAKASH INSTITUTECHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ASSIGNMENT (SECTION - C)
37 Videos
- MOTION IN A STRAIGHT LINEBOOK - AAKASH INSTITUTECHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - EXERCISE
30 Videos
- MOTION IN A PLANEBOOK - AAKASH INSTITUTECHAPTER - MOTION IN A PLANEEXERCISE - Assignement section -J (Aakash Challengers Questions)
4 Videos
- MOTION IN STRAIGHT LINEBOOK - AAKASH INSTITUTECHAPTER - MOTION IN STRAIGHT LINEEXERCISE - Assignment (SECTION - J)
2 Videos
Similar Questions
Knowledge Check
A ball is projected vertically up with speed 20 m/s. Take g=10m/s2
AIt covers a distance of 5 m in 2nd second of its motionBThe displacement in 2nd and 3rd second are equalCThe distance covered in 2nd and 3rd second are equalDThe average speed for first 4 seconds is zeroTwo balls are projected simultaneously with the same speed from the top of a tower, one vertically up wards and the other vertically downwards. If the first bll strikes the ground with speed 20m/s then speed of second ball when it strikes the ground is.
A10m/sB20m/sC40m/sDData insufficientA ball projected from ground vertically upward is at same height at time t1andt2. The speed of projection of ball is [Neglect the effect of air resistance ]
Ag[t2−t1]Bg[t1+t2]2Cg[t2−t1]2Dg[t1+t2]
Two balls are thrown up simultaneously from the top of a 400m high tower with speed 10m/s and 55m/s. Sketch a graph showing the separation between the balls versus time.
View SolutionTwo balls of different masses are thrown vertically upwards with the same speed . They pass through the point of projection in their downward motion with the same speed ( Neglect air resistance ).
View SolutionTwo balls are projected simultaneously from the top of a tall building. The first ball is projected horizontally at speed u1=10m/s and the other one is projected at an angle θ=tan−1(43) to the horizontal with a velocity u2.[g=10m/s2]
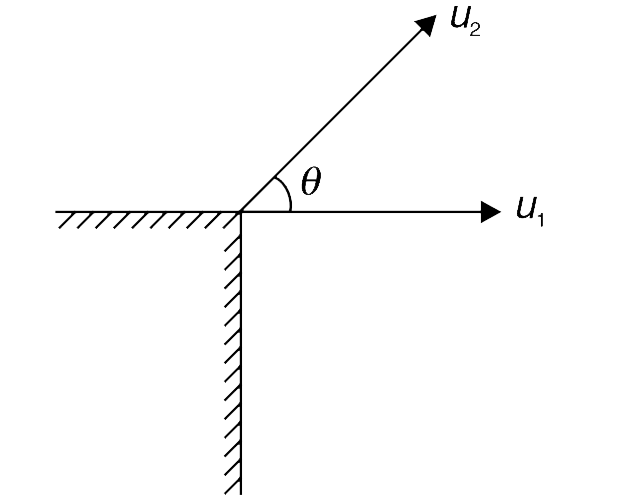
(a) Find minimum value of u2(=u0) so that the velocity vector of the two balls can get perpendicular to each other at some point of time during their course of flight. ltbr? (b) Find the time after which velocities of the two balls become perpendicular if the second one was projected with speed u0.
View SolutionTwo balls are thrown simultaneously, A vertically upwards with a speed of 20 m/s from the ground, and B vertically downwards from a height of 40 m with the same speed along the same line of motion. At what point do the two balls collide? Take g=9.8m/s2.
View SolutionA body is projected vertically upward with speed 40m/s. The distance travelled by body in the last second of upward journey is [take g=9.8ms2 and neglect eggect of air rresistance]:-
View Solution