A ball projected from ground vertically upward is at same height at time $t_{1} and t_{2}$ . The speed of projection of ball is [Neglect the effect of air resistance ]

A

$g [t_{2} - t_{1}]$

B

$\frac{g [t_{1} + t_{2}]}{2}$

C

$\frac{g [t_{2} - t_{1}]}{2}$

D

$g [t_{1} + t_{2}]$

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:B

To solve the problem of finding the speed of projection of a ball that is projected vertically upward and reaches the same height at times $t_{1}$ and $t_{2}$ , we can follow these steps:
1. Understanding the Motion:
- The ball is projected upwards from the ground with an initial speed $V$ .
- It moves upwards against the acceleration due to gravity $g$ until it reaches its maximum height, where its velocity becomes zero.
2. Time of Flight:
- The ball reaches the same height at two different times $t_{1}$ and $t_{2}$ . This implies that the time taken to go up to the maximum height and come back down to the same height is symmetrical.
- Let $Δ t$ be the time taken to go from the height $h$ to the maximum height and back down to the same height $h$ . Thus, we can express the times as:
$t_{2} = t_{1} + 2 Δ t$
- From this, we can derive:
$Δ t = \frac{t_{2} - t_{1}}{2}$
3. Using the First Equation of Motion:
- The first equation of motion states:
$v = u - g t$
- At the maximum height, the final velocity $v = 0$ , and the time taken to reach the maximum height from the initial position is $t_{1} + Δ t$ .
- Therefore, we can write:
$0 = V - g (t_{1} + Δ t)$
- Rearranging gives:
$V = g (t_{1} + Δ t)$
4. Substituting for $Δ t$ :
- Now, substitute $Δ t$ from step 2 into the equation:
$V = g (t_{1} + \frac{t_{2} - t_{1}}{2})$
- Simplifying this gives:
$V = g (\frac{2 t_{1} + t_{2} - t_{1}}{2}) = g (\frac{t_{1} + t_{2}}{2})$
5. Final Expression for Speed of Projection:
- Thus, the speed of projection $V$ can be expressed as:
$V = \frac{g (t_{1} + t_{2})}{2}$
Conclusion:
The speed of projection of the ball is given by:
$V = \frac{g (t_{1} + t_{2})}{2}$

Updated on:18/9/2024

|

Class 12 PHYSICS MOTION IN A STRAIGHT LINE

Topper's Solved these Questions

Similar Questions

Knowledge Check

A particle projected from ground moves at angle $45^{\circ}$ with horizontal one second after projection and speed is minimum two seconds after the projection The angle of projection of particle is [ Neglect the effect of air resistance )
A ${tan}^{- 1} (3)$
B ${tan}^{- 1} (2)$
C ${tan}^{- 1} (\sqrt{2})$
D ${tan}^{- 1} (4)$
A particle is projected vertically upward and is at a height h after $t_{1}$ seconds and again after $t_{2}$ seconds
A $h = {gt}_{1} t_{2}$
B $h = \frac{1}{2} {gt}_{1} t_{2}$
C $h = \frac{2}{g} t_{1} t_{2}$
D $h = \sqrt{{gt}_{1} t_{2}}$
Two stones are projected from level ground. Trajectory of two stones are shown in figure. Both stones have same maximum heights above level ground as shown. Let $T_{1}$ and $T_{2}$ be their time of flights and $u_{1}$ and $u_{2}$ be their speeds of projection respectively (neglect air resistance). then

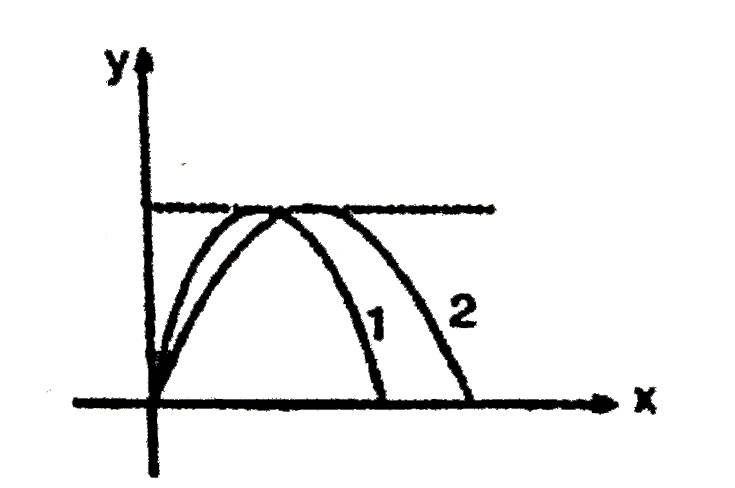A $T_{2} > T_{1}$
B $T_{1} = T_{2}$
C $u_{1} > u_{2}$
D $u_{1} = u_{2}$

AAKASH INSTITUTE-MOTION IN A STRAIGHT LINE-ASSIGNMENT (SECTION - A)

Home
Ask Doubt
Profile