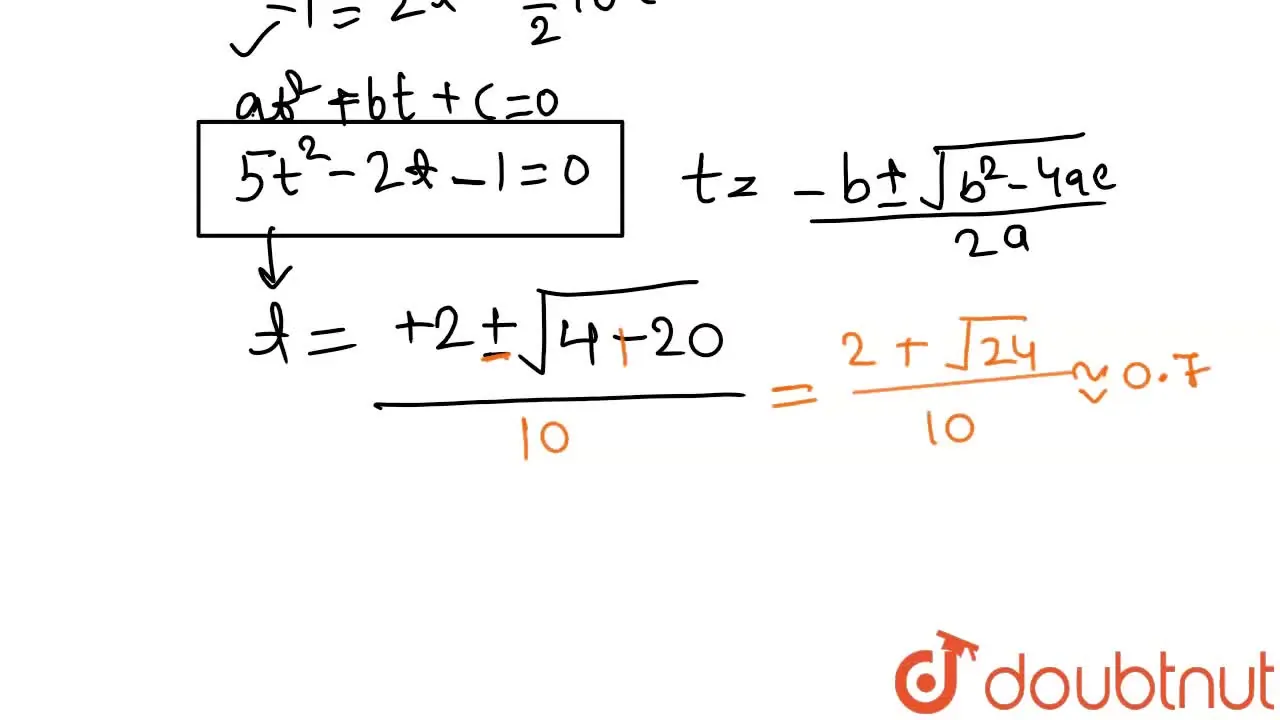
A balloon starts rising from ground from rest with an upward acceleration . Just after 1 s, a stone is dropped from it. The time taken by stone to strike the ground is nearly
0.3 s
0.7 s
1 s
1.4 s
The correct Answer is:B
Step by step video, text & image solution for A balloon starts rising from ground from rest with an upward acceleration 2m//s^(2). Just after 1 s, a stone is dropped from it. The time taken by stone to strike the ground is nearly by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - AAKASH INSTITUTECHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ASSIGNMENT (SECTION - B)33videos
MOTION IN A STRAIGHT LINE
- BOOK - AAKASH INSTITUTECHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ASSIGNMENT (SECTION - C)37videos
MOTION IN A STRAIGHT LINE
- BOOK - AAKASH INSTITUTECHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - EXERCISE30videos
MOTION IN A STRAIGHT LINE
- BOOK - AAKASH INSTITUTECHAPTER - MOTION IN A PLANEEXERCISE - Assignement section -J (Aakash Challengers Questions)4videos
MOTION IN A PLANE
- BOOK - AAKASH INSTITUTECHAPTER - MOTION IN STRAIGHT LINEEXERCISE - Assignment (SECTION - J)2videos
MOTION IN STRAIGHT LINE
Similar Questions
A balloon starts rising from the ground with an acceleration 2ms−2. After 5second,as→≠isre≤asedomtheball∞on.F∈dthetimetakenbythes→≠→reachthegroundafteritsre≤aseand→talheightaa∈edbyball∞onwhens→≠reachestheground.Takeg==10 ms^(-2).
View SolutionA balloon starts rising from the ground with a constant acceleration of 1.25m/s2. After 8 s, a stone is released from the balloon. Find the time taken by the stone to reach the ground. (Take g=10m/s2)
View SolutionA ballon starts risintg from ground from rest at some constant acceleration. After some time, a storne is dropped from it. If the stone reaches the ground in the same time in which balloon reached the dropping poing from ground, find the acceleration of the balloon.
View SolutionA balloon starts rising from the ground with an acceleration of 1.25ms−2. After 8 seconds, a stone is released from the balloon. After releasing, the stone will:
View SolutionA balloon starts from the state of rest from the ground with constant acceleration g/n. after time T, a stone is dropped from the balloon. If stone takes time T to reach the ground then calculate value of n.
View SolutionA balloon starts rising from ground with an acceleration of 1.25m/sec2. A 8 seconds a stone is released from the balloon. The maximum height from the ground reached by stone will be.
View SolutionA balloon starts rising from the ground with an acceleration of 1.25 m/s2 . A stone is released from the balloon after 10s. Determine
(1) maximum height of ston from ground
(2) time taken by stone to reach the groundView SolutionA balloon carrying a stone rises from rest on the ground with a constant acceleration 10m/s2. After 5 s, the stone is released and ultimately it strikes the ground. Sketch a v-t graph for the stone and the maximum height attained by it.
View SolutionA balloon is going vertically upwards with a velocity of 10m/s. When it is 75m above the ground, a stone is gently relesed from it. The time taken by the stone to reach the ground is (g=10m/s2)
View SolutionA balloon is rising vertically upwards with uniform acceleration 15.7m/s2 .A stone is dropped from it. After 4s another stone is dropped from it.Find the distance between the two stones 6 second after the second stone is dropped.
View Solution