A 100 mark examination was administered to a class of 50 students. Despite only integer marks being given, the everge score of the class was 47.5. Then , the maximum number of students who could get marks more than the class average is
25
35
45
49
The correct Answer is:D
To solve the problem step by step, we need to analyze the given information and derive the maximum number of students who could have scored more than the average score of the class.
Step 1: Calculate the total marks obtained by the class.
The average score of the class is given as 47.5, and there are 50 students. The total marks can be calculated using the formula:
Total Marks=Average×Number of Students
Substituting the values:
Total Marks=47.5×50=2375
Step 2: Determine the implications of the average score.
Since the average score is 47.5, this means that some students scored below this average while others scored above it. To maximize the number of students scoring above the average, we need to consider how the remaining students can score.
Step 3: Assume a scenario for maximum students scoring above average.
Let’s assume that x students scored more than 47.5 and the remaining 50−x students scored the minimum possible marks, which is 0 (since marks are integers).
If x students scored just above the average, we can assume they scored 48 marks each (the smallest integer above 47.5). Therefore, the total marks contributed by these x students would be:
Marks from x students=48x
The remaining 50−x students scored 0 marks, contributing:
Marks from (50−x) students=0
Step 4: Set up the equation for total marks.
The total marks from all students must equal the total marks calculated earlier (2375):
48x+0(50−x)=2375
This simplifies to:
48x=2375
Step 5: Solve for x.
Now, we can solve for x:
x=237548≈49.79
Since x must be an integer (as it represents the number of students), we take the maximum integer value less than or equal to 49.79, which is 49.
Conclusion:
Thus, the maximum number of students who could score more than the average of 47.5 is:
49
The average score of the class is given as 47.5, and there are 50 students. The total marks can be calculated using the formula:
Since the average score is 47.5, this means that some students scored below this average while others scored above it. To maximize the number of students scoring above the average, we need to consider how the remaining students can score.
Let’s assume that x students scored more than 47.5 and the remaining 50−x students scored the minimum possible marks, which is 0 (since marks are integers).
The total marks from all students must equal the total marks calculated earlier (2375):
Now, we can solve for x:
Thus, the maximum number of students who could score more than the average of 47.5 is:
Topper's Solved these Questions
Similar Questions
Knowledge Check
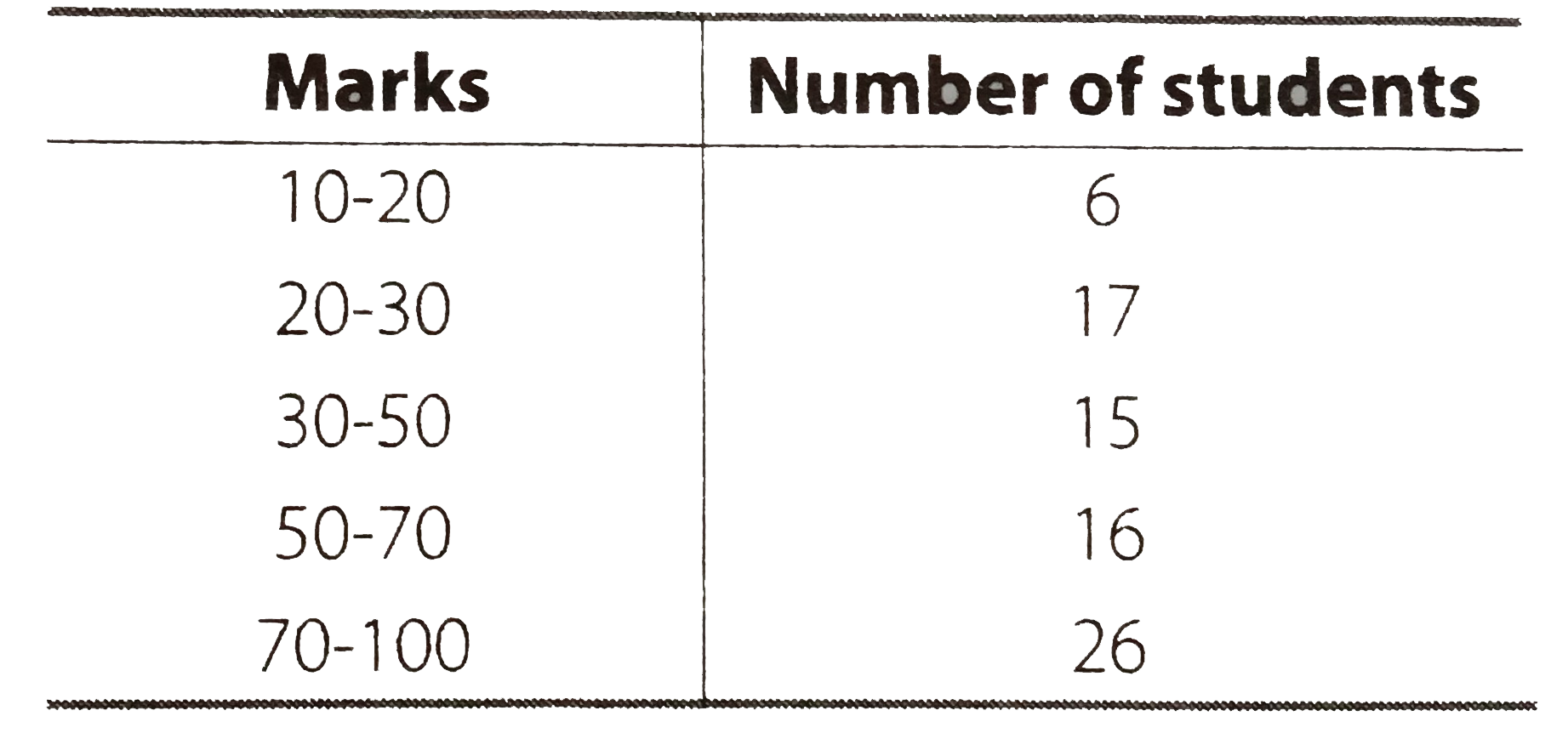
The class, in which the marks of maximum number of students lies, is
 A40.5 to 50.5B50.5 to 60.5C60.5 to 70.5D70.5 to 80.5
A40.5 to 50.5B50.5 to 60.5C60.5 to 70.5D70.5 to 80.5In a class of 100 students, there are 70 boys , whose average marks in a subject are 75. If the average marks of the complete class is 72, then what is the average marks of the girls?
A73B65C68D74In a class of 100 students, there are 70 boys whose average marks in a subject is 75. If the average marks of the complete class is 72, then what is the average of the girls ?
A73B65C68D74
In a class of 50 students, 60% are boys. The average of marks of the boys is 62, and that of the girls is 68. What is the average marks of the whole class ?
View Solution_2019_CBT6_E01_019_Q01.png)
The marks of 20 students in an examination are given in the following table. Average marks of these student is:
View SolutionIn an examination average marks obtained by the girls of a class is 85 and the average marks obtained by the boys of the same class is 87. If the girls and boys are in the ratio 4:5, average marks of the whole class (approximately) is closest to
View SolutionIn a class of 15 students, the total marks obtained by all the students in a test is 600. Find the average mark of the class.
View SolutionIn a class of 50 students 10 have failed and their average marks are 28. The total marks obtained by the entire class are 2800. The average marks of those who have passed is
View Solution