All the vertices of a rectangle are of the form (a, b) with a, b integers satisfying the equation (a−8)2−(b−7)2=5. Then the perimeter of the rectangle is
20
22
24
26
The correct Answer is:A
(a−8)2−(b−7)2=5
(a−b−1)(a+b−15)=5
l1l2Four casesl1l255−5−1−1−5
Case-1
a−b−1=5 & a+b−15=1
⇒a=11,b=5
Case-2
a−b−1=5 & a+b−15=−1
⇒a=5,b=9
Case-3
a−b−1=1 & a+b−15=5
⇒a=11,b=9
Case-4
a−b−1=−1 & a+b−15=−5
⇒a=5,b=5
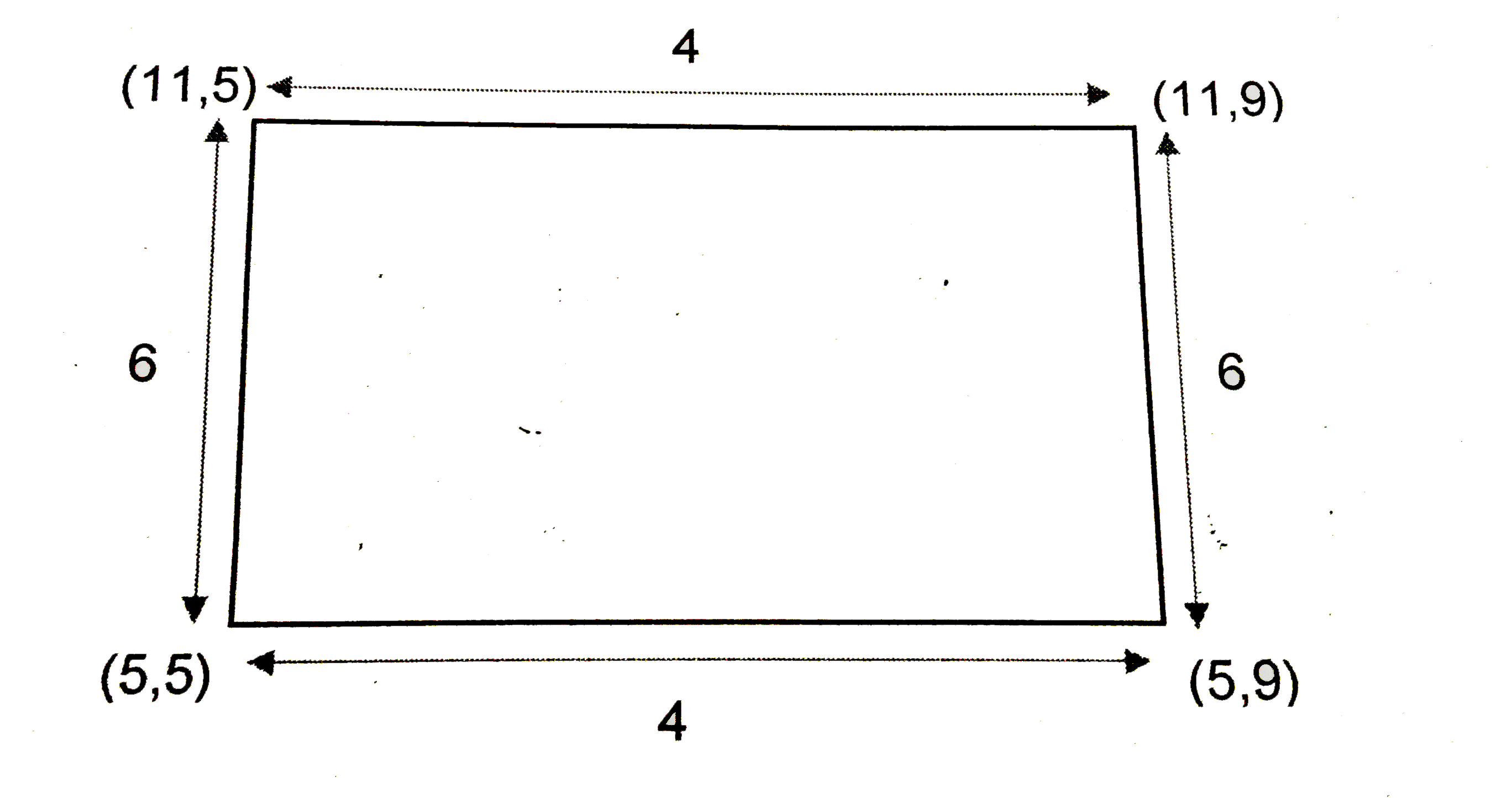
Perimeter=4+4+6+6=20
Topper's Solved these Questions
Similar Questions
The perimeter of a square = 4s
Area of a square = s×s
Perimeter of a rectangle = 2(l+b)
Area of a rectangle = l×b
If length is thrice of the breadth of a rectangular hall. Find the perimeter of the rectangle.
View SolutionThe sides of a rectangle are in the ratio 7:5 and its perimeter is 96 cm. the length of the rectangle is
View Solution
Knowledge Check
If the perimeters of a rectangle and a square are equal and the ratio of two adjacent sides of the rectangle is 1:2 then the ratio of area of the rectangle and that of the square is
A1:1B1:2C2:3D8:9If the perimeters of a rectangle and a square are equal and the ratio of two adjacent sides of the rectangle is 1:2 then the ratio of area of the rectangle and that of the square is
A1:1B1:2C2:3D8:9The perimeter of a rectangle with length l and breadth b is _______ .
A2(l×b)B2(l−b)C2(l+b)Dl+b
In the rectangle shown, the value of a−b is
View SolutionThe length of a rectangle is 8 cm and its area is 48 cm2. The perimeter of the rectangle is 14 cm (b) 24 cm 12 cm (d) 28 cm
View SolutionFind the dimension of the rectangle of area 96cm2 whose perimeter is the least, Also, find the perimeter of the rectangle
View SolutionFour equilateral triangles are described on the four sides of a rectangle with perimeter 12 cm. If the sum of the areas of the four triangles is 10√3 cm2 , what is the area of the rectangle? (a) 5 cm2 (b) 8 cm2 (c) 9 cm2 (d) 6.75 cm2
View SolutionThe area of a rectangle is 1.8 times the area of a square. The length of the rectangle is 5 times the breadth. The side of the square is 20 cm. What is the perimeter of the rectangle?
View Solution
