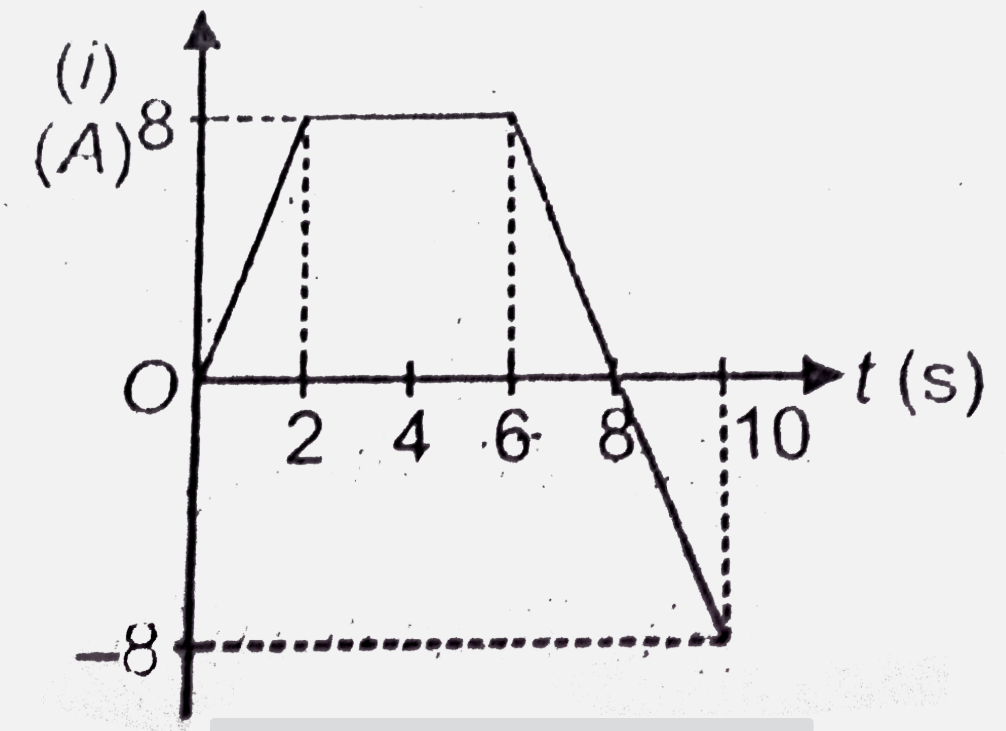
A (current vs time) graph of the current passing through a solenoid is shown in Fig. For which time is the back electromotive force (u) a maximum? If the back emf t = 3 s is e, find the back emf at t = 7 s, 15 s and 40 s OA, AB and BC are straight line segments. 
The back electromotive force in solenoid is (u) a maximum when there is maximum rate of change of current. This occurs is in AB part of the graph. So maximum back emf will be obtained between 5s<t<10s.
Since the back emf at t= 3s is e,
Also,
the rate of change of current at t=3,s= slope of OA from t=0s to t=5s=1/5A/s.
So, we have
If u=L1/5(fort=3s,dIdt=1/5) (L is a constant). Applying ε=−LdIdt
Similarly, we have for other values
For 5s<t<10s
u1=−L35=−35L=−3e
Thus, at t=7s,u1=−3e
For 10s<t<30s
u−2=L220=L10=12e
For t>30s,u2=0
Thus, the back emf at t=7s,15s and 30s are −3e,e/2 and 0 respectively .
Topper's Solved these Questions
Similar Questions
Knowledge Check
The velocity-time graph of a particle moving in a straight line is shown in figure. The mass of the particle is 2kg. Work done by all the forces acting on the particle in time interval between t=0 to t=10s is
 A(a) 300JB(b) −300JC(c) 400JD(d) −400J
A(a) 300JB(b) −300JC(c) 400JD(d) −400JDisplacement-time graph of a particle moving in a straight line is as shown in figure. s t a o b c
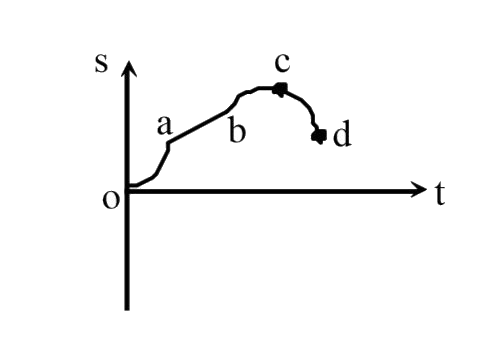
Q. Find the sign of velocity in regions oa, ab, bc and cdAnegative , positive, positive, negativeBpositive , positive, positive, negativeCpositive , negative, positive, positiveDpositive , positive, negative, negativeIn the circuit shown in figure switch S is closed at time t=0
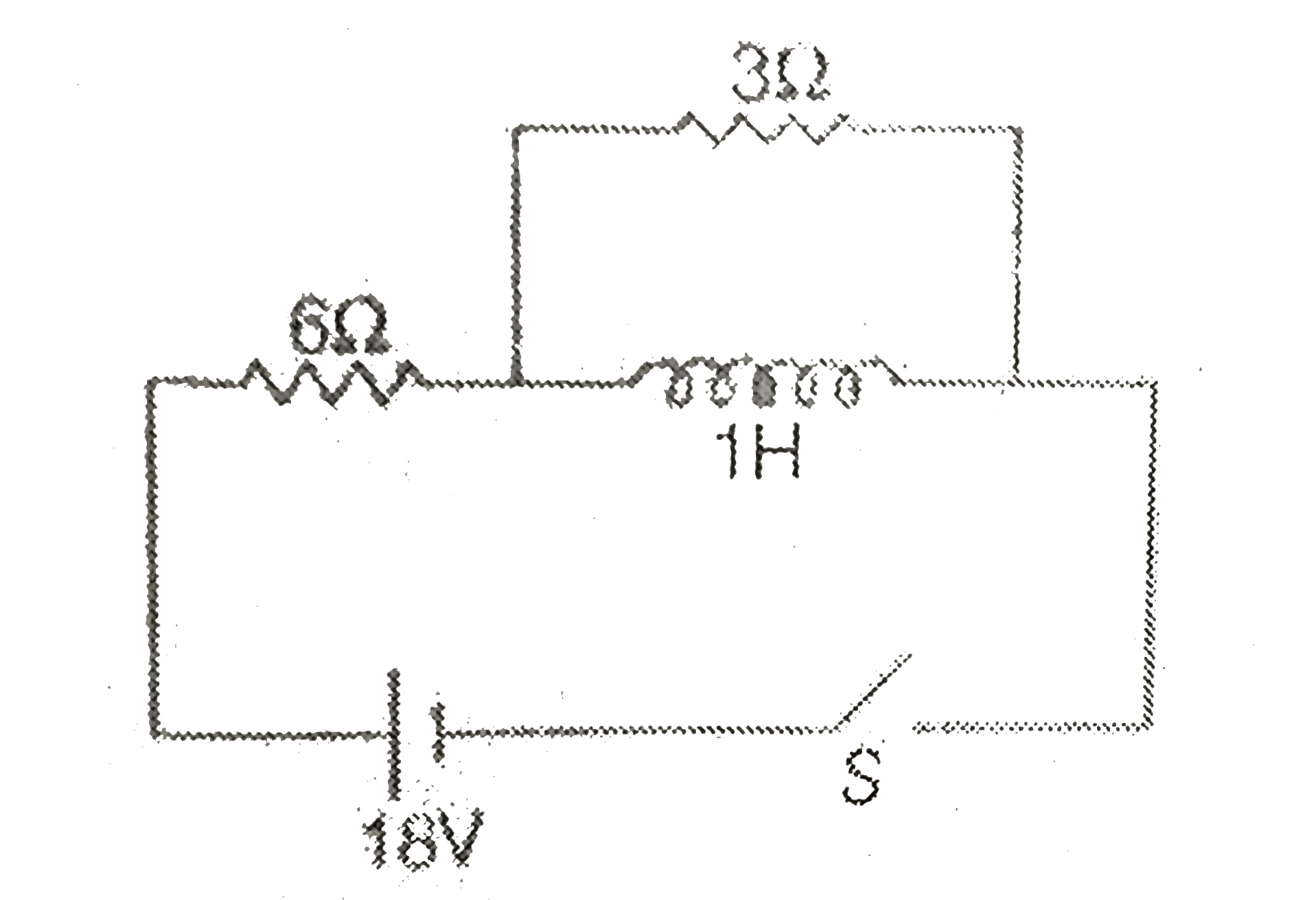
Current I from the battery at time t is given byA3(1−e−2t)B3+e−2t)C3(1-e^(-t/9))D3−e−2t
The velocity (u)-time (t) graph of an object moving along a straight line is as shown is Fig. 2 (b) . 30. Calculate the distance covered by object between (i)t=0→t=5s (ii) t=0→t=10s.
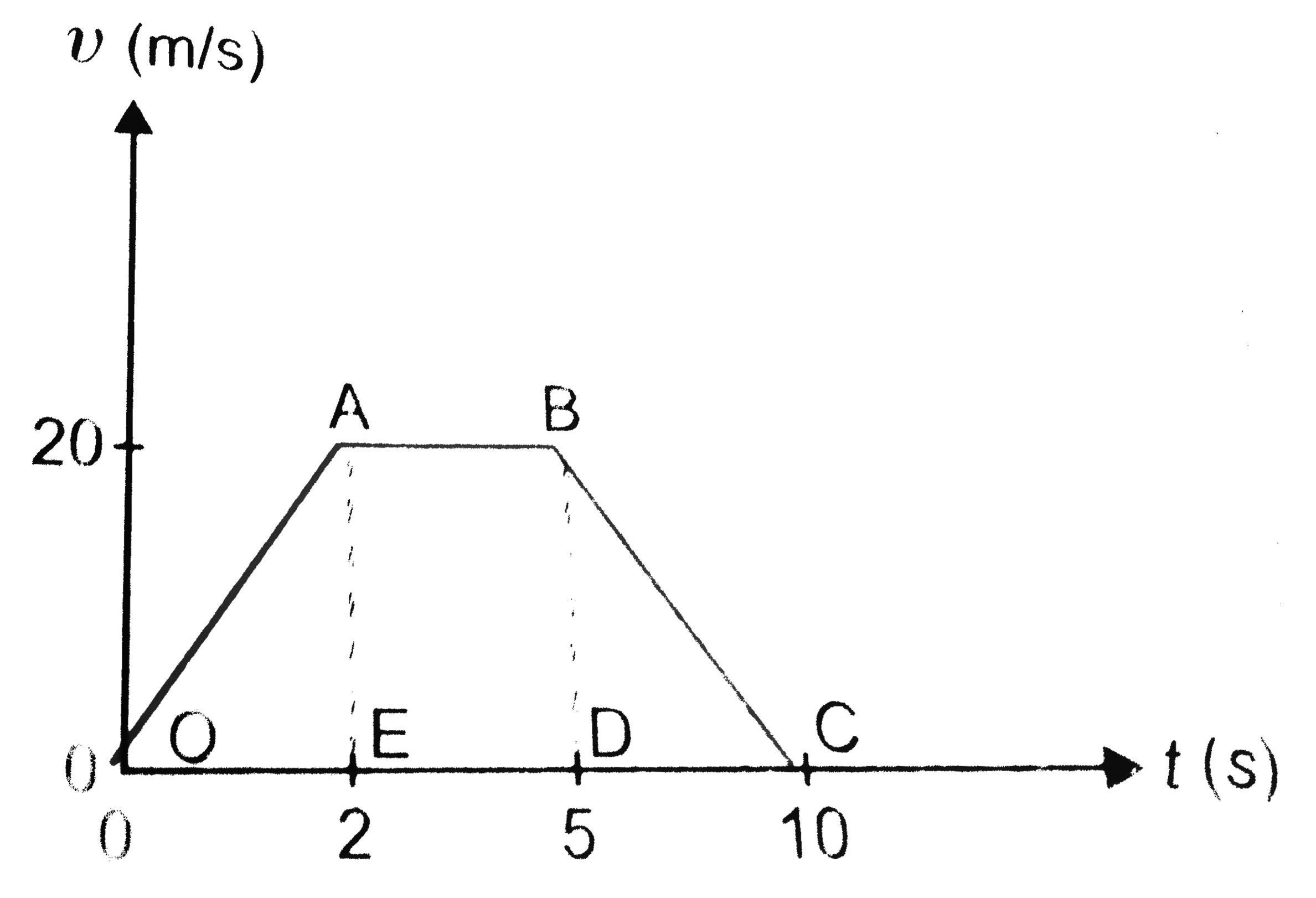 .
.
View SolutionThe velocity-time graph of an object moving along a straight line is as shown in Fig. 3.37. Find the net distance covered by the object in time interval between t=0 to t=10s. Also find the displacement in time 0 to 10 s.
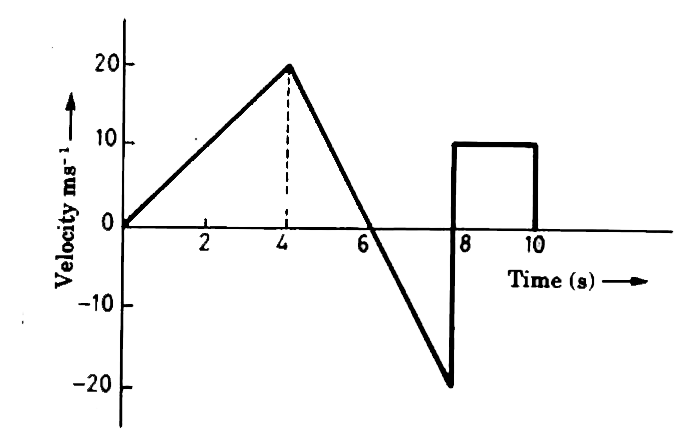
View SolutionThe velocity (u)-time (t) graph of an object moving along a straight line is as shown is Fig. 2 (b) . 30. Calculate the distance covered by object between (i)t=0→t=5s (ii) t=0→t=10s.
 .
.
View SolutionVelocity-time graph of a particle moving in a straight line is shown in figure. At time t=0.s=20m. Plot a-t and s-t graphs of the particle.
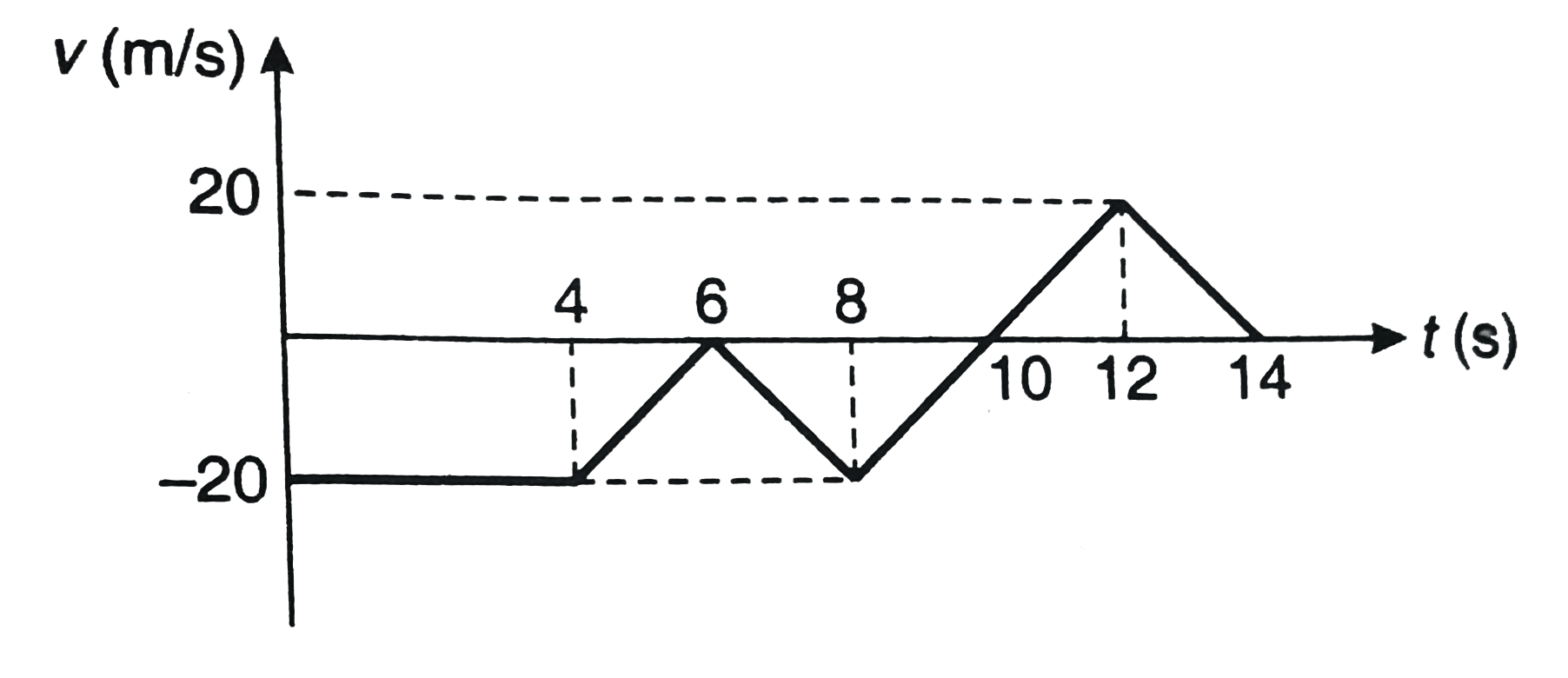
View SolutionVelocity-time graph of a particle moving in a straight line is shown in figure. In the time interval from t = 0 to t = 14 s, find:
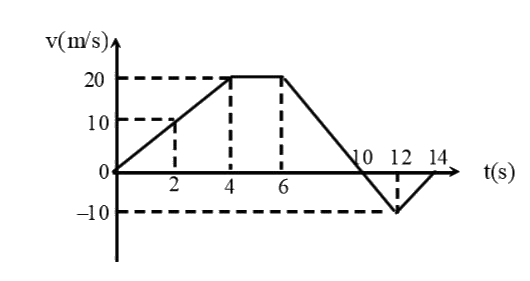
Q. average speed of the particle
View Solution