One end of a V-tube containing mercury is connected ot a suction pump and the other end to atmosphere. The two arms of the tube are inclined to horizontal at an angle of 45∘ each. A small pressure difference is created between two columns when the suction pump is removed. Will Neglect capillary and viscous forces. Find the time period of oscillation.
Consider the diagram shown below
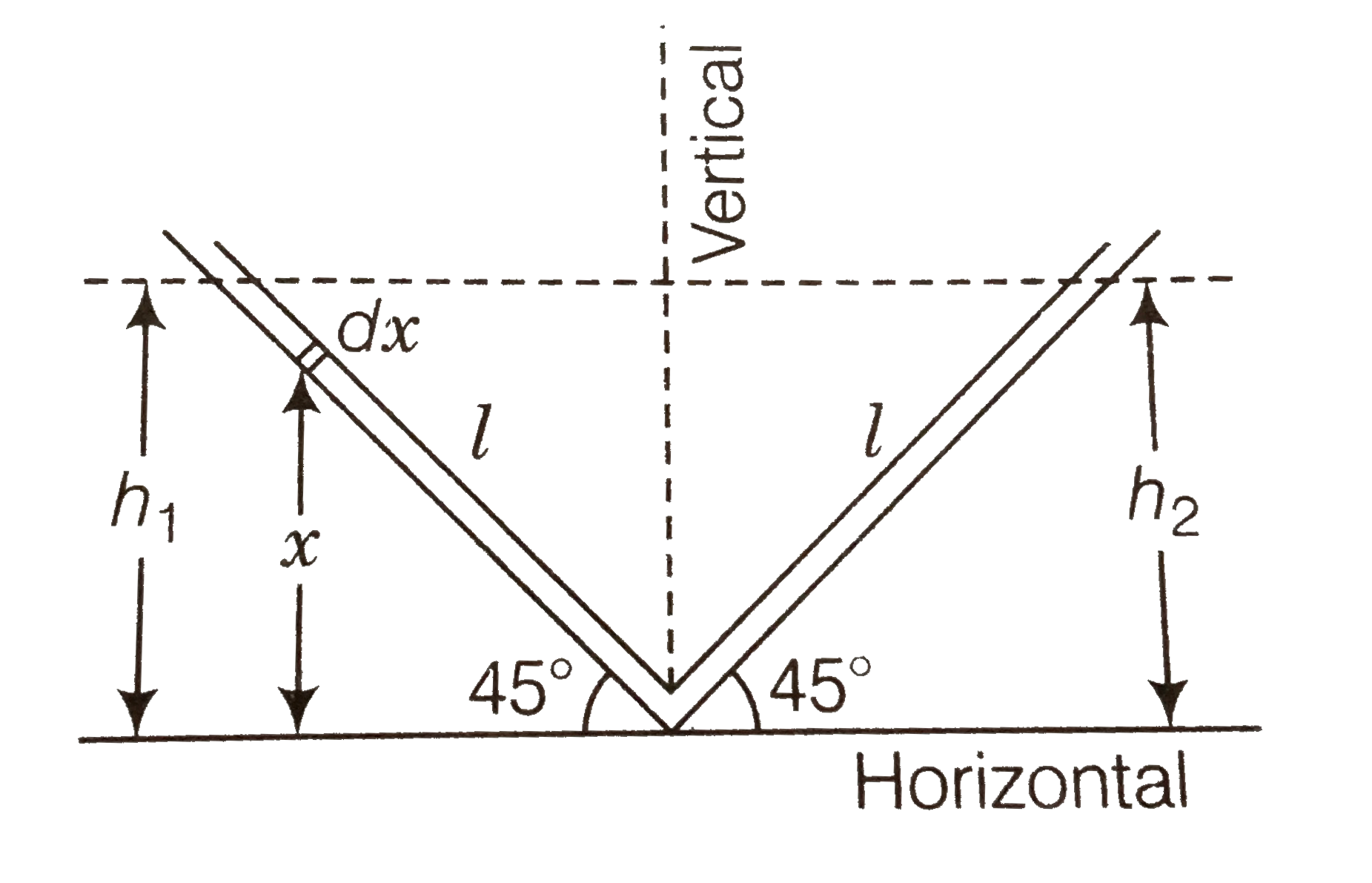
Let us consider an infinitesimal liquid column of length dx at a height x from the horizontal line.
If p = density of the liquid
A= cross-sectional area of V-tube
PE of element dx will be given as
PE=dmgx=(Apdx)gx [∴dm=pv=pAdx]
where Apdx=dm=mass of element dx
Total PE of the left column
h1∫oApgdx
=Apgh1∫oxdx
=Apg∣∣∣x22∣∣∣h1=Apgh212
But, h1=lsin45∘
∴PE=Apg2l2sin245∘.....(i)
In a similar way,
PE of right column=Apg2l2sin245∘ .....(ii)
Total PE=Apg2l2sin245∘+Apg2l2sin245∘
=2×12Apgl2(1√2)2=Apgl22 ....(iii)
If due to pressure difference created y element of left side moves on the side, then
liquid present in the left arm=l-y
liquid present in the right arm=l+y
∴Total PE=Apg(l−y)2sin245∘+Apg(l+y)2sin245∘
Changes in PE=(PE)final−(PE)initial
or ΔPE=Apgg[(l−y)2+(l+y)2−l2]
=Apg2[l2+y2−2ly+l2+y2+2ly−l2]
=Apg2[2(l2+y2)]
=Apg(l2+y2) ....(iv)
If v is the change in velocity of the total liquid column, then change in KE
ΔKE=12mv2
But m=Ap(2l)
ΔKE=12Apg2lv2=Aplv2 .....(v)
From Eqs. (iv)and (v),
ΔPE+KP=Apg(l2+y2)+Aplv2 .....(vi)
Sytem being conservative.
:. Change in total enregy =0
From Eq.(vi)
Apg(l2+y2)+Aplv2=0
Differentiating both sides with respect to time (t), we get
Apg[0+2ydydt]+Apl(2v)dvdt=0
But dydt=vanddvdt=a [accelecration]
⇒Apg(2u=yv)+Ap(2v)=0
⇒(gy+la)2Apv=0
2Apv=constantand2Apv≠0
∴la+gy=0
a+(gl)y=0
or d2ydt2+(gl)y=0
This is the standard differential equation for SHM of the form
d2ydt2+ω2y=0
∴ω=√gl
∴T=2πω=2π√lg
Topper's Solved these Questions
Similar Questions
One end of V-tube containing mercury is connected to a suction grouup and the other end to atmosphere. The two arms of the tube are inclined to horizontal at an angle of 45∘ each. A small pressure difference is created between two columns when the ssuction pump is removed. Will the column of mercuty in V-tube execute simple harmonic motion? Neglect capillary and viscous forces. Fing the time period of oscillation.
View SolutionOne end of a U-tube containing mercury is connected to a suction pump and the other end is connected to the atmosphere. A small pressure difference is maintained between the two columns. Show that when the suction pump is removed, the liquid in the U-tube executes SHM.
View Solution
Knowledge Check
one end of a U-tube of uniform bore (area A) containing mercury is connected to a suction pump. Because of it the level of liquid of density ρ falls in one limb. When the pump is removed, the restoring force in the other limb is:
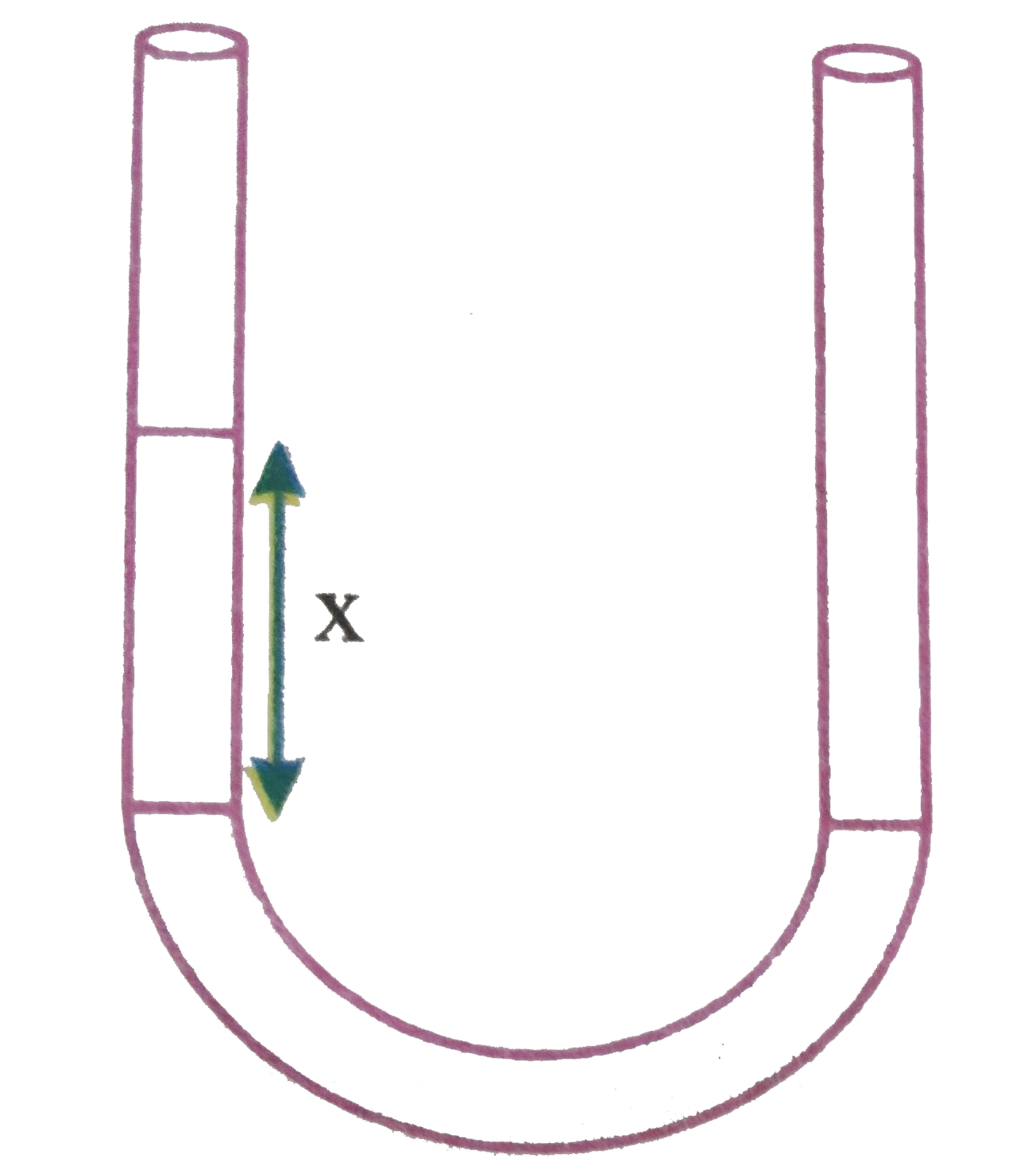
A2xρAgBxρgCAρgDxρAgOne end a U-tube of unifrom bore (area A) cpmtaining mercury is connected to sunction pump . Because of it the level of liquid of density ρ falls in one limb. When the pump is removed, the restoring force in the other limb is
 A2xρAgBxρgCAρgDxρAg
A2xρAgBxρgCAρgDxρAgA horizontal thin tube of length 2L completely filled with a liquid of density δ rotates about a vertical axis passing through one of its end and along the diameter of its side face with an constant angular velocity ω. Two vertical thin long tubes are fitted with the horizontal tube to measure the pressure difference between points A and B which are at same horizontal level as shown. Neglect viscous forces and surfaces tension of liquid.

The difference in the heights (h) of the liquid column in the two vertical thin tubes is :Aω2L22gB3ω2L22gC5ω2L22gDNone of these
One end of a U-tube containing mercury is connected to a suction pump and the other end to atmosphere. A small pressure difference is maintained between the two columns. Show that, when the suction pump is removed, the column of mercury in the U-tube executes simple harmonic motion.
View SolutionOne end of a U-tube containing mercury is connected to a suction pump and the other end to atmosphere. A small pressure difference is maintained between the two columns. Show that, when the suction pump is removed, the column of mercury in the U-tube executes simple harmonic motion.
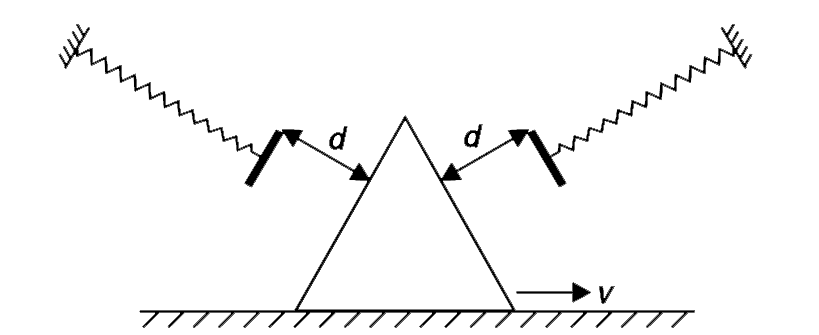
View SolutionAn equilateral prism of mass m is kept on a smooth table between two identical springs each having a force constant of k. The two springs have their lengths perpendicular to the inclined faces of the prism and are constrained to remain straight. The ends of the springs have light pads aligned parallel to the faces of the prism, and distance between pads and the incline faces is d. The prism is imparted a velocity v to the right. Find time period of its oscillation.
View SolutionTwo spherical soap bubbles of diameters 10 cm and 6 cm are formed, one at each end of a narrow horizontal glass tube. If the surface tension of the soap solution is 0.03Nm−1, then the pressure difference in pascal between the two ends of the tube is
View SolutionA horizontal thin tube of length 2L completely filled with a liquid of density δ rotates about a vertical axis passing through one of its end and along the diameter of its side face with an constant angular velocity ω. Two vertical thin long tubes are fitted with the horizontal tube to measure the pressure difference between points A and B which are at same horizontal level as shown. Neglect viscous forces and surfaces tension of liquid.

The graph of the pressure gradient (dPdx) with the distance x meaured from the axis of rotation is :
View Solution
