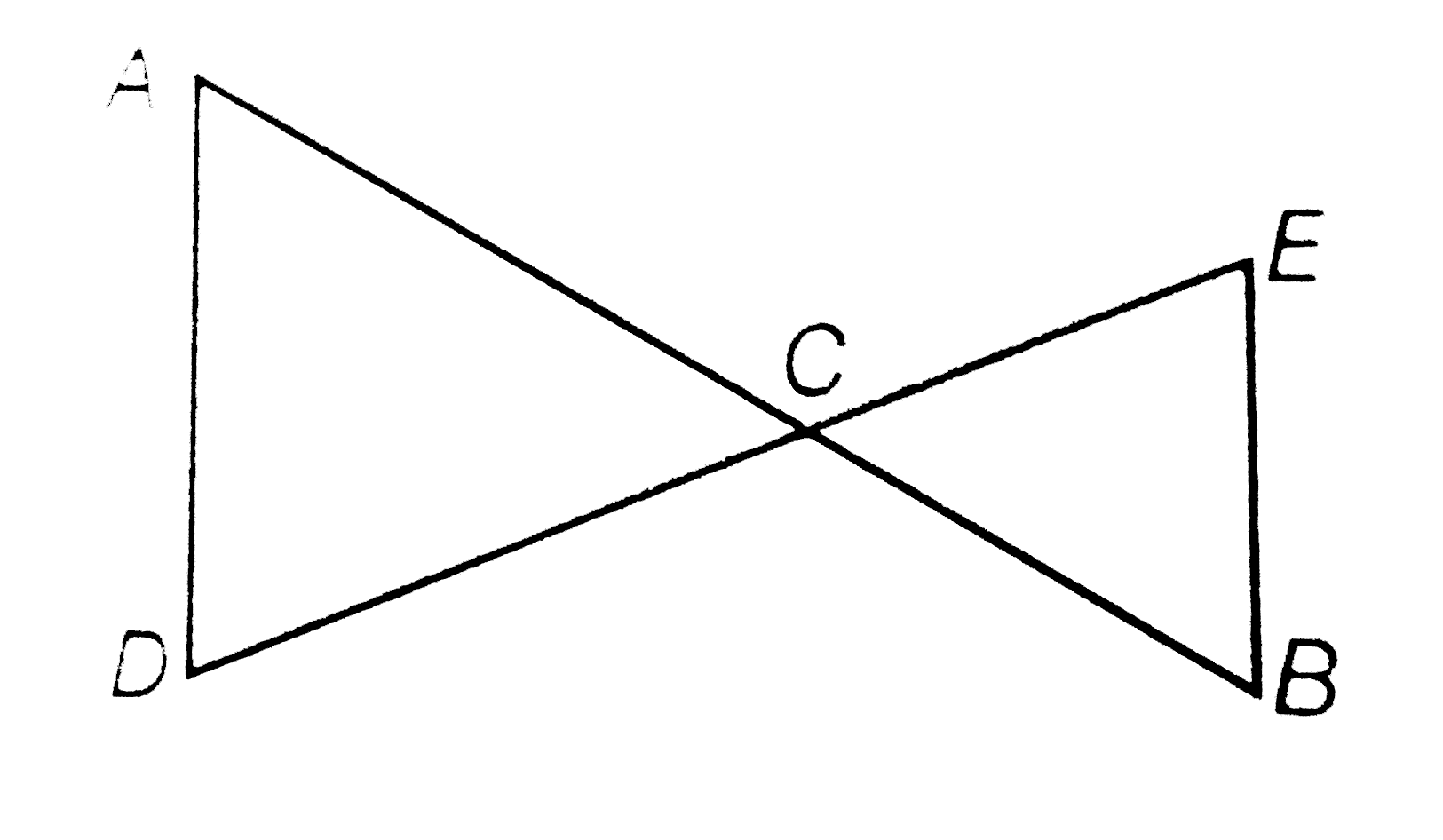
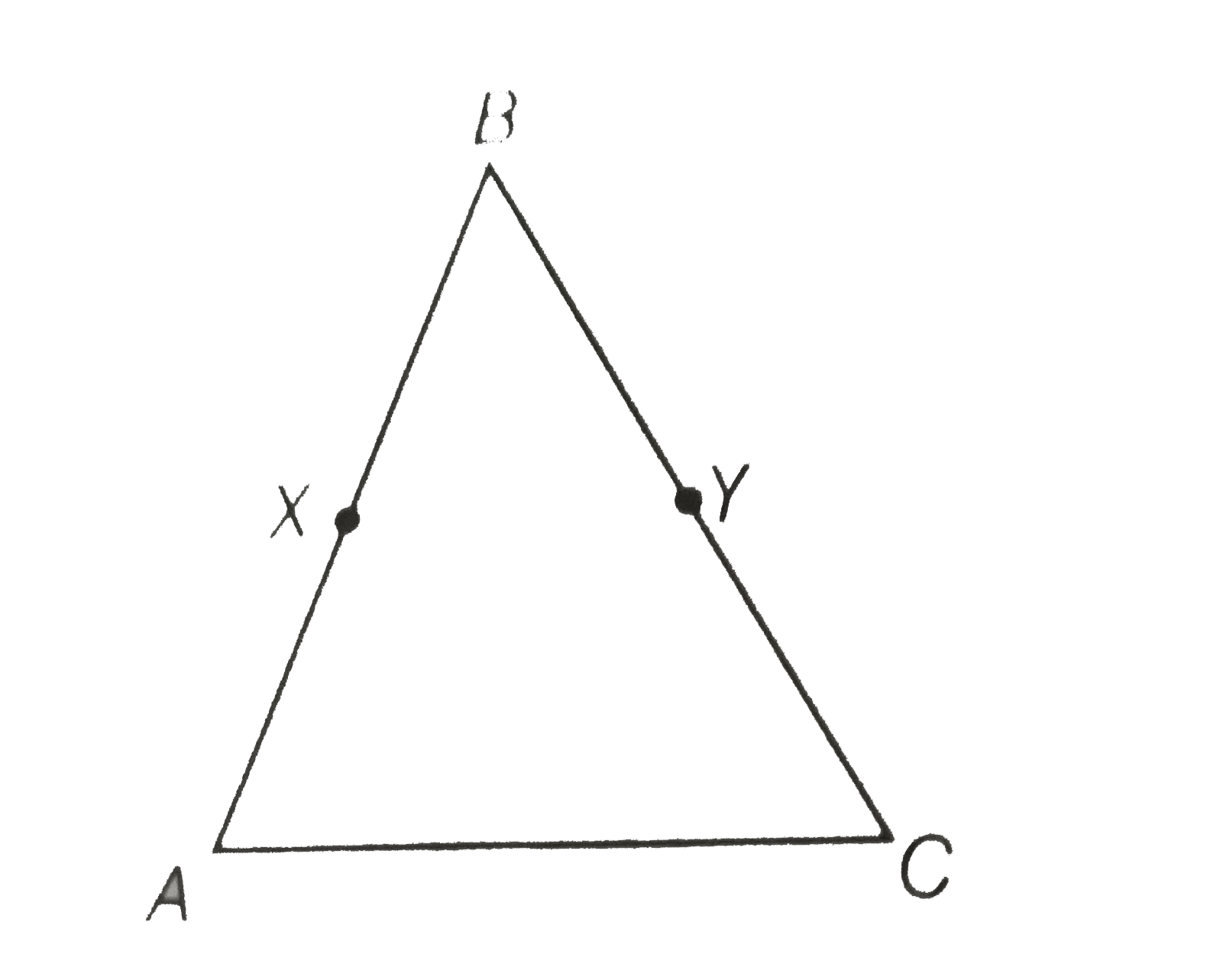
In the adjoining figure, if AB = BC and BX =BY, then show that AX=CY
Video Solution
Text Solution
we have , AB=BC …(i)
and BX =BY ...(ii)
According to Euclid's axiom , if equals are subtracted from equals , the remainders are equal. So . on Subtracting Eq. (i) , we get
AB -BX =BC -BY
⇒ AX=CY ["From figure"]
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
In the adjoining figure AB∣∣CDandPQ=SR, then :
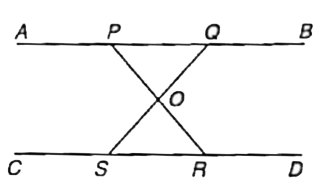 APQ=PSBSR=RPCPS=QRDAP=RD
APQ=PSBSR=RPCPS=QRDAP=RD
In the adjoining figure,AB=AC.Find the value of x
View SolutionIn the adjoining figure AE||CD and BC||ED, then find Y.
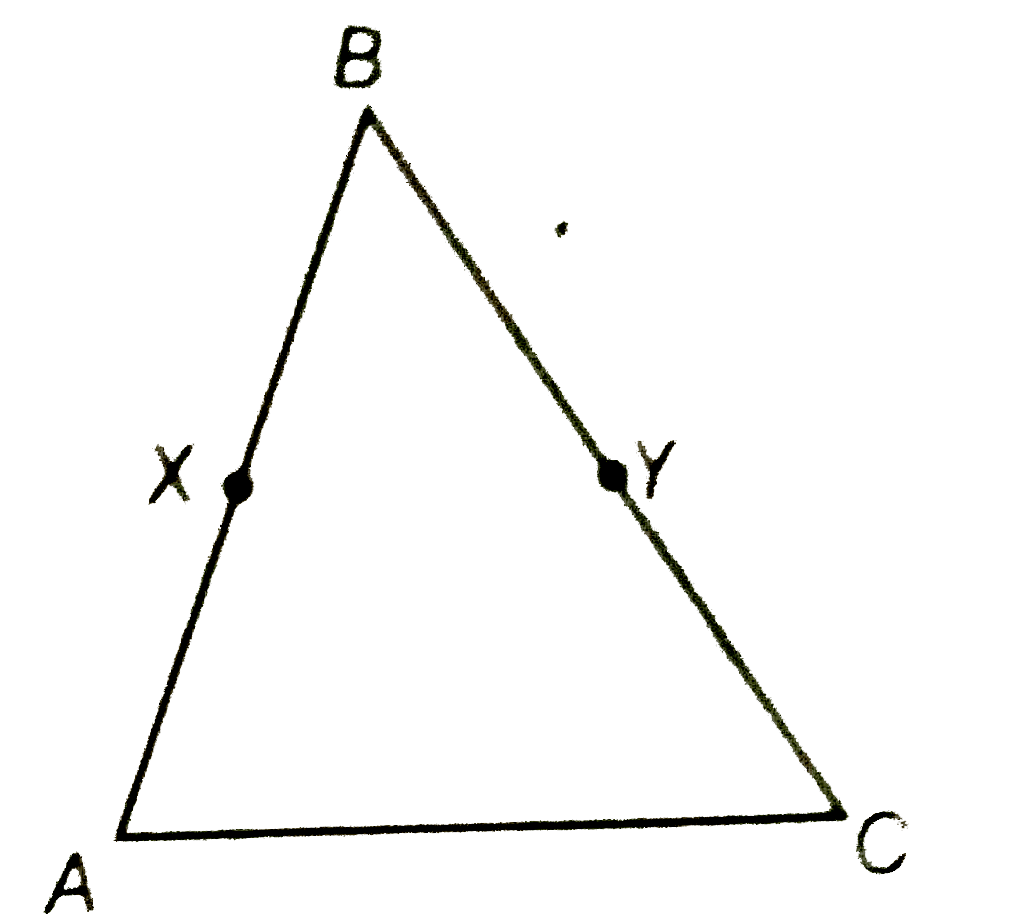
View SolutionIn the adjoining figure , if BX=12AB,BY=12BCandAB=BC, then show that BX =BY.
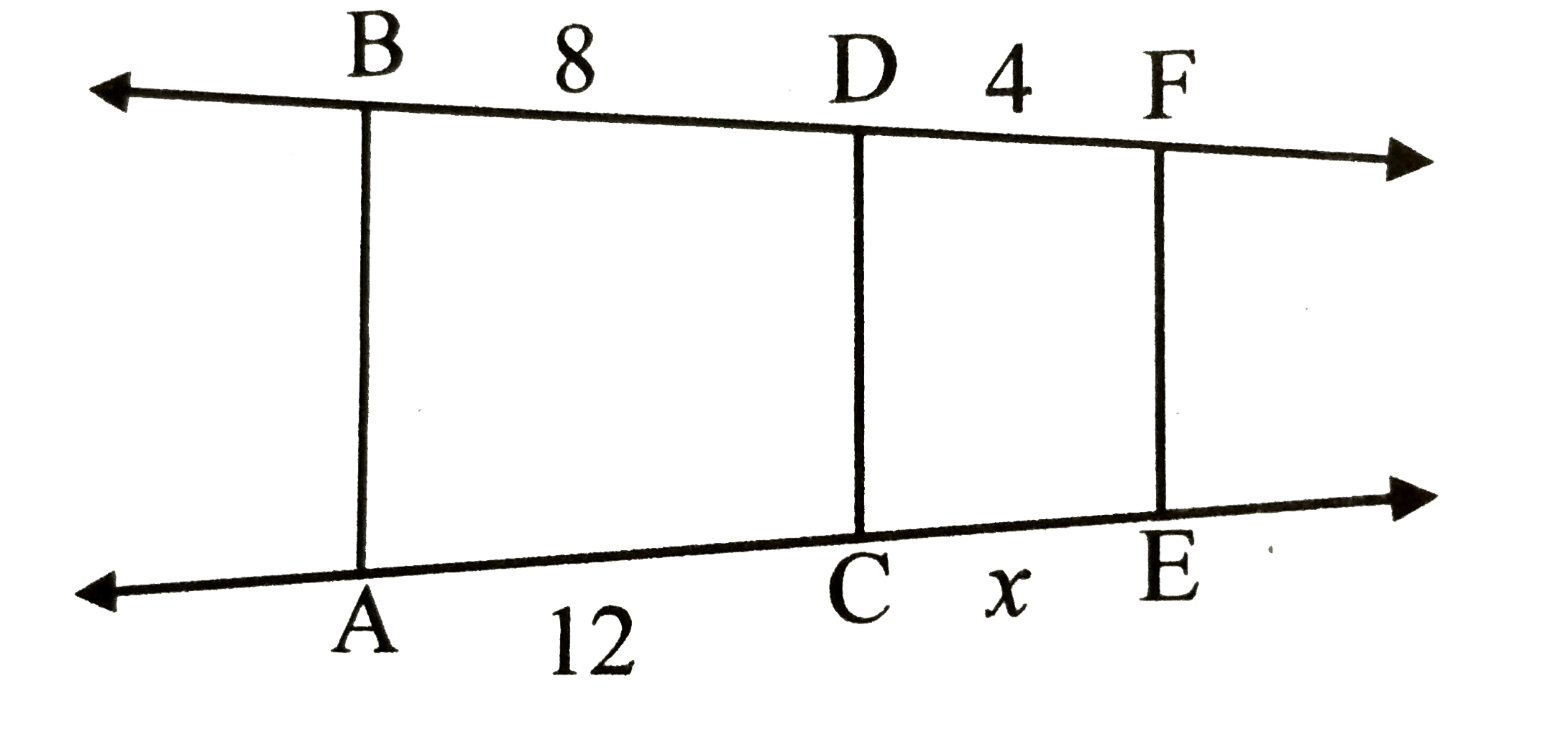
View SolutionIn the adjoining figure, if AB||CD||FE, then find x and AE.
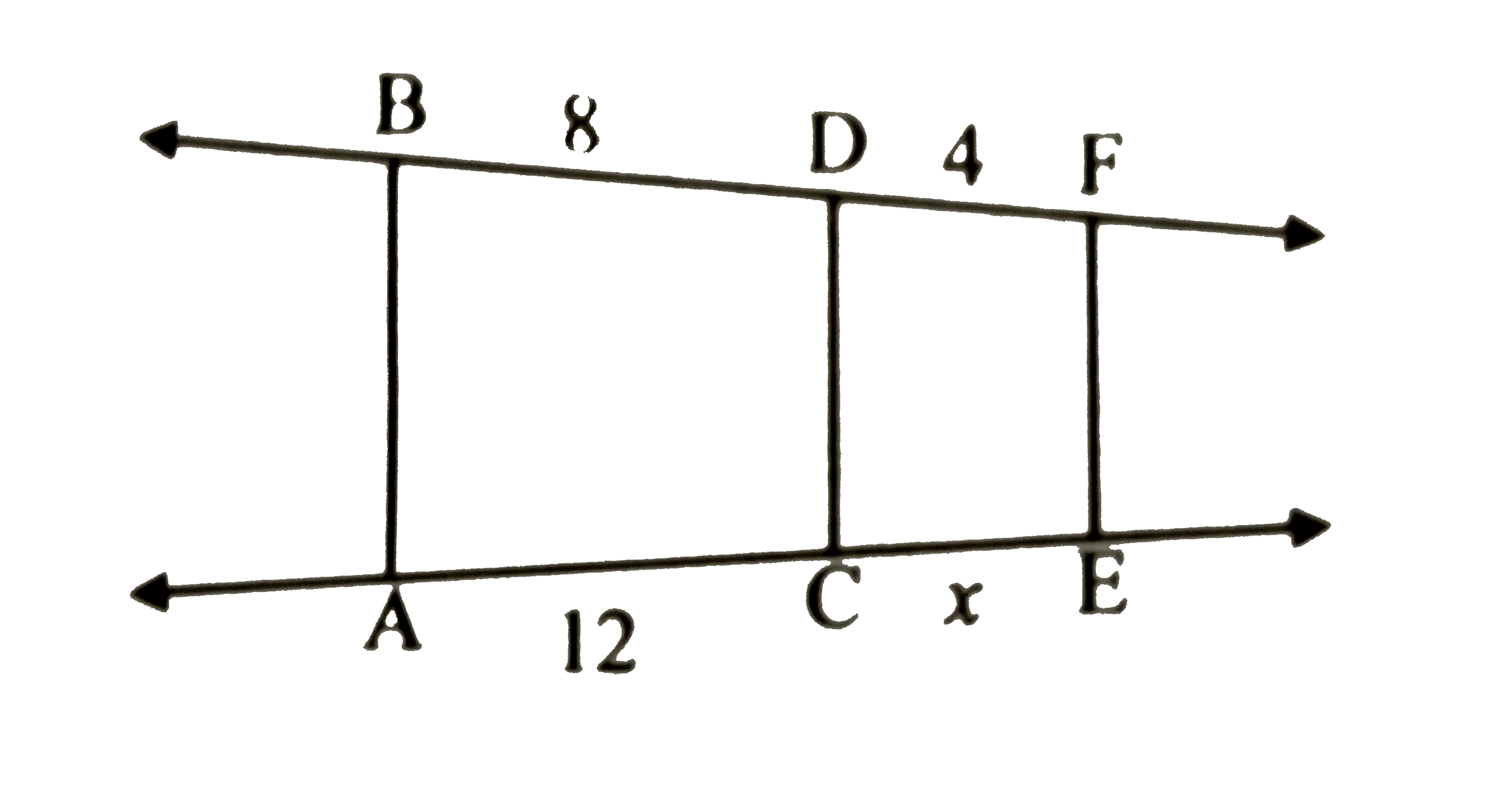
View SolutionIn the adjoining figure, if AB || CD || FE, then find x and AE.
View SolutionIn the adjoining figure, BC = ED, then, prove that ΔABC≅ΔFDE.
View SolutionIn the given figure, ∠BDC=∠BEA and AB=BC. Show that AE=CD.
View Solution