ABC is an isosceles triangle with AB=AC and D is a point on AC such that BC2=ACxCD. Prove that BD=BC
To prove that BD=BC in the isosceles triangle ABC where AB=AC and D is a point on AC such that BC2=AC⋅CD, we can follow these steps:
1. Given Information:
- Triangle ABC is isosceles with AB=AC.
- Point D is on line segment AC.
- The relation BC2=AC⋅CD holds.
2. Rearranging the Given Relation:
- From the equation BC2=AC⋅CD, we can rearrange it to express the ratio:
BCCD=ACBC
3. Identifying Similar Triangles:
- The ratio of the sides suggests that triangles ABC and BDC are similar by the Side-Angle-Side (SAS) similarity criterion. This is because:
- ∠ABC=∠BDC (common angle)
- ABAC=BCCD (from the rearranged relation)
4. Establishing Corresponding Angles:
- Since the triangles ABC and BDC are similar, their corresponding angles are equal:
- ∠ABC=∠BDC
- ∠ACB=∠DBC
- ∠BAC=∠BDC
5. Using the Properties of Similar Triangles:
- From the similarity of triangles, we can conclude that the sides opposite to equal angles are proportional:
BCBD=ACBC
6. Cross Multiplying:
- By cross-multiplying, we get:
BC2=AC⋅BD
7. Using the Given Condition:
- We know from the problem statement that BC2=AC⋅CD. Therefore, we can set the two expressions equal to each other:
AC⋅BD=AC⋅CD
8. Dividing by AC:
- Since AC≠0, we can divide both sides by AC:
BD=CD
9. Conclusion:
- Since BD=CD and BC=BD from the similarity, we conclude that:
BD=BC
Final Result:
Thus, we have proved that BD=BC.
- Triangle ABC is isosceles with AB=AC.
- Point D is on line segment AC.
- The relation BC2=AC⋅CD holds.
- From the equation BC2=AC⋅CD, we can rearrange it to express the ratio:
BCCD=ACBC
- The ratio of the sides suggests that triangles ABC and BDC are similar by the Side-Angle-Side (SAS) similarity criterion. This is because:
- ∠ABC=∠BDC (common angle)
- ABAC=BCCD (from the rearranged relation)
- Since the triangles ABC and BDC are similar, their corresponding angles are equal:
- ∠ABC=∠BDC
- ∠ACB=∠DBC
- ∠BAC=∠BDC
- From the similarity of triangles, we can conclude that the sides opposite to equal angles are proportional:
BCBD=ACBC
- By cross-multiplying, we get:
BC2=AC⋅BD
- We know from the problem statement that BC2=AC⋅CD. Therefore, we can set the two expressions equal to each other:
AC⋅BD=AC⋅CD
- Since AC≠0, we can divide both sides by AC:
BD=CD
- Since BD=CD and BC=BD from the similarity, we conclude that:
BD=BC
Thus, we have proved that BD=BC.
Topper's Solved these Questions
Similar Questions
ABC is a triangle. D is a point on AB such that D=14AB and E is a point on AC such that AE=14AC. Prove that DE=14BC
View SolutionABC is a triangle. D is a point on AB such that AD=14AB and E is a point on AC such that AE=14AC. Prove that DE=14BC.
View SolutionIf ABC is an isosceles triangle such that AB=AC and AD is an altitude from A on BC . Prove that (i) ∠B=∠C (ii) AD bisects BC (iii) AD bisects ∠A
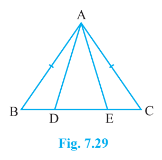
View SolutionIn an isosceles triangle ABC with AB=AC, D and E are points on BCsuch that BE=CD(see Fig. 7.29). Show that AD=AE
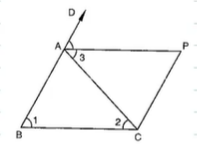
View SolutionIn Figure, ABC is an isosceles triangle in which AB=AC.CPparel≤lAB and AP is the bisector of exterior ∠CAD of ABC . Prove that ∠PAC=∠BCA and (ii) ABCP is a parallelogram.
View SolutionABCD is a parallelogram. E is a point on BA such that BE=2 EA and F is a point on DC such that DF=2 FC. Prove that AE CF is a parallelogram whose area is one third of the area of parallelogram AB CD.
View SolutionABCD is a parallelogram, G is the point on AB such that AG=2GB,E is a point of DC such that CE=2DE andF is the point of BC such that BF=2FC. Prove that: ar(EBG)=ar(EFC)
View SolutionA point D is taken on the side BC of a ABC such that BD=2dC. Prove that ar(ABD)=2ar(ADC).
View SolutionLet ABC be an isosceles triangle with AB=AC and let D, E, F be the mid points of BC, CA and AB respectively. Show that AD⊥FE and AD is bisected by FE
View SolutionABC is a triangle in which D is the mid-point of BC and E is the mid-point of AD. Prove that area of △BED=14areaof△ABC. GIVEN : A ABC,D is the mid-point of BC and E is the mid-point of the median AD. TO PROVE : ar(△BED)=14ar(△ABC).
View Solution
