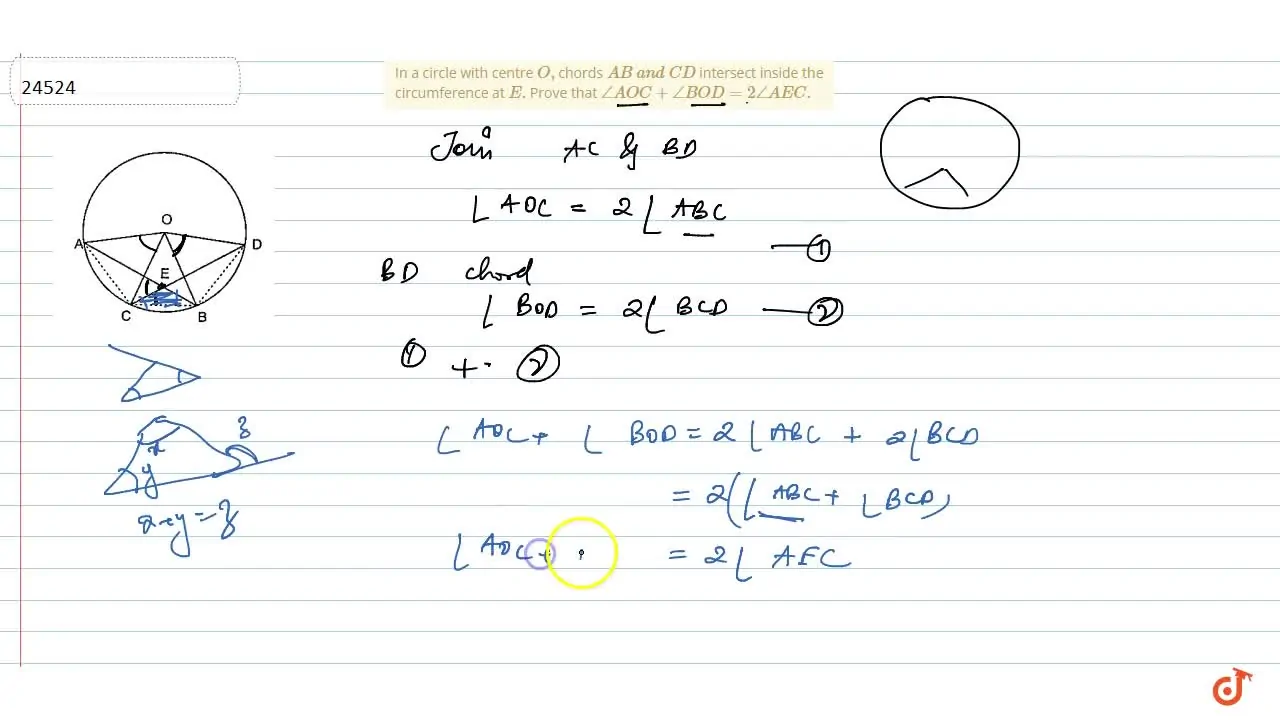
In a circle with centre O, chords ABandCD intersect inside the circumference at E. Prove that ∠AOC+∠BOD=2∠AEC.
In the figure, the same arc ACsubtends ∠AOC on the center and ∠ABC on the other part.
⇒∠AOC=2∠ABC [Angle subtended on the center of the circle is double the angle subtended on another part of the circle by the same arc.]
Similarly, arc BD subtends ∠BOD and ∠DCB.
⇒∠BOD=2∠DCB
On adding the above results, we get
∠AOC+∠BOD=2∠ABC+2∠DCB=2(∠ABC+∠DCB)... (1)
In triangle ECB, by exterior angle property, we have
∠AEC=∠ECB+∠EBC
⇒∠AEC=∠DCB+∠ABC... (2)
On putting the value of (2) in (1), we get
∠AOC+∠BOD=2∠AEC
[Hence proved]
Related Playlists
Similar Questions
In a circle with centre O, chords ABandCD intersect inside the circumference at E. Prove that ∠AOC+∠BOD=2∠AEC.
View SolutionIn a circle with centre O, chords AB and CD intersect inside the circumference at E. Prove that ∠AOC+ ∠BOD=2 ∠AEC
View SolutionIn a circle with centre O,chords AB and CD intersect inside the circumference at E.Prove that ∠AOC+∠BOD=2∠AEC
View SolutionIn a circel with centre O. chords AB and CD interest inside the circumference at E. Prove that ∠AOC+BOD=2∠AEC.
View SolutionIn a circle with centre O, chords AB and CD intersect inside the circumference at E. Find ∠AEC. If ∠AOC=40∘and∠BOD=50∘.
View SolutionIf O is the circumcentre of a ABC and OD⊥BC, prove that ∠BOD=∠A.
View SolutionIn the figure, two equal chords AB and CD of a circle with centre O, intersect each other at E, Prove that AD=CB
View SolutionIn a circle with centre O, AB is a chord and BC is a diameter. From centre O. perpendicular OD is drawn to chord AB. Prove that OD=12AC. .
View SolutionIf two chords AB and AC of a circle with centre O are such that the centre O lies on the bisector of ∠BAC; Prove that AB=AC
View SolutionThe common tangents AB and CD to two circles with centres O and O' intersect at E between the centres. Prove that the points O, E and O' are collinear.
View Solution