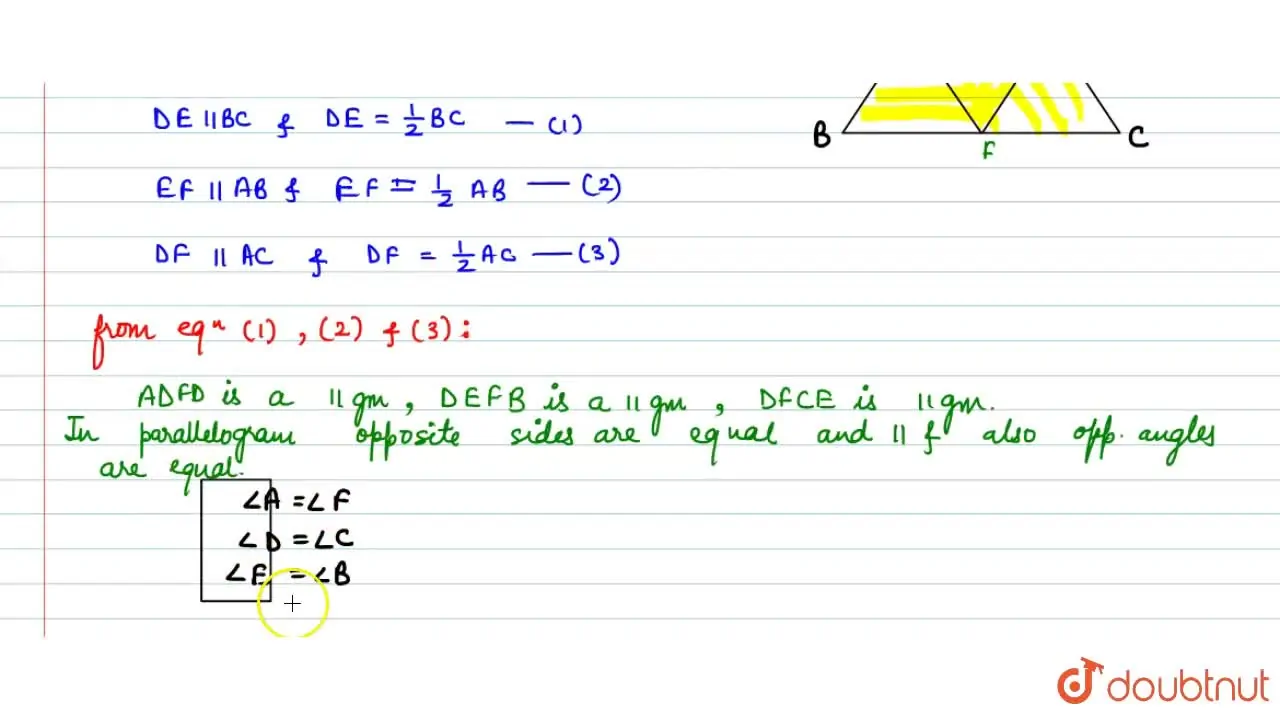
In a ABC , find the measures of the angles of the triangle formed by joining the mid-points of the sides of this triangle.
Given D,E,F are mid point AB,BC and CA
DE||AB,FE||BC and DF||CA
DE||AB and BC and CA tranverse
∠CDE=∠B
∠CED=∠A
EF||BC ∠AEF=∠C and ∠AFE=∠B
OF||CA ∠BDF=∠C and ∠BFD=∠A
∠BDF+∠FDE+∠EDC=180∘
∠C+∠FOE+∠B=180∘
∠FDE=180−(∠B+∠C)
∠FDE=∠A
∠DEF=180∘−(∠A+∠C)=∠B
∠EFD=180∘−(∠A+∠B)=∠A
∠D=∠A,∠E=∠B,∠F=∠C.
Related Playlists
Similar Questions
In a ABC , find the measures of the angles of the triangle formed by joining the mid-points of the sides of this triangle.
View SolutionIn a triangle ∠ABC, ∠A=50∘, ∠B=60∘and∠C=70∘ . Find the measures of the angles of the triangle formed by joining the mid-points of the sides of this triangle.
View SolutionAny two of the four triangles formed by joining the mid - points of the sides of a given triangle are :
View SolutionWrite the type of triangle formed by joining the midpoints of the sides of an equilateral triangle.
View SolutionAny two of the four triangles formed by joining the mid - points of the sides of a given triangle are :
View SolutionIn ΔABC,∠A=50∘,∠B=30∘and∠C=100∘ What are the angles of the triangle formed by joining the midpoints of the sides of this triangle?
View SolutionOne side of an equilateral triangle is 36 cm. The mid-points of its sides are joined to form another triangle. Again another triangle is formed by joining the mid-points of the sides of this triangle and the process is continued indefinitely. Determine the sum of areas of all such triangles including the given triangle.
View SolutionThe sides of a triangle are 3 cm, 4 cm and 5 cm. The area (in cm2) of the triangle formed by joining the mid-points of the sides of this triangle is 34 (b) 32 (c) 3 (d) 6
View SolutionFill in the blanks to make the following statements correct
The triangle formed by joining the mid-points of the sides of an isosceles triangle is ....
The triangle formed by joining the mid-points of the sides of a right triangle is ..........
The figure formed by joining the mid-points of consecutive sides of a quadrilateral is ....View SolutionO is orthocentre of a triangle PQR, which is formed by joining the mid- points of the sides of a △ABC, is :
View Solution