A tube filled with water and closed at both ends uniformly rotates in a horizontal plane about the axis. The manometers and fixed in the tube at distances and from rotational axis indicate pressure and respectively. Determine the angular velocity of rotation of the tube.
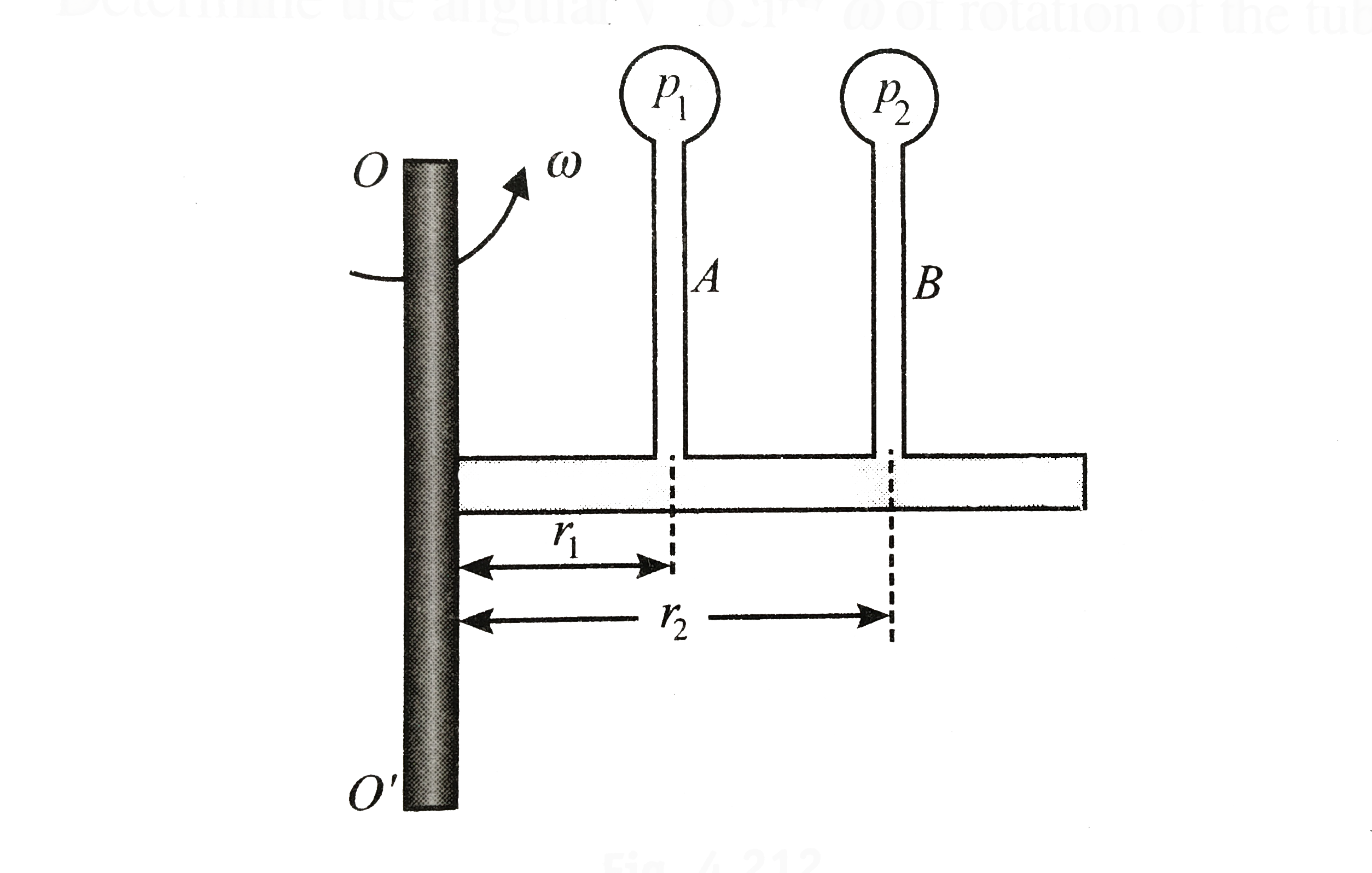
The correct Answer is:A
Similar Questions
A closed tube filled with water is rotating uniformly in a horizontal plane about the axis OO as shown in the figure. The manometers A and B which are fixed on the tube at distances r1andr2, indicate pressures P1andP2 respectively. The angular velocity (ω) of the tube is
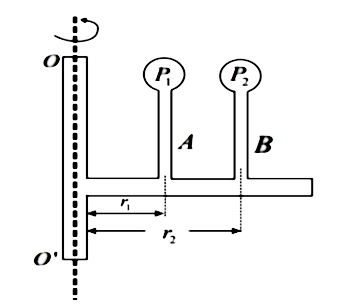
View SolutionA rod PQ of length L revolves in a horizontal plane about the axis YY´. The angular velocity of the rod is w. If A is the area of cross-section of the rod and r be its density, its rotational kinetic energy is
View Solution
Knowledge Check
Two particles A and B are located at distances rA and rB from the centre of a rotating disc such that rA>rB . In this case
(Angular velocity (ω) of rotation is constant)Aboth A and B do not have any accelerationBboth A and B have same accelerationCA has greater acceleration than BDB has greater acceleration than BA tube one metre long is filled with liquid of mass 1 kg. The tube is closed at both the ends and is revolved about one end in a horizontal plane at 2 rev/s. The force experienced by the liquid at the other end is
A4π2NB8π2NC16π2ND20π2NA uniform rod of mass m and length l rotates in a horizontal plane with an angular velocity ω about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
A12mω2xB12mω2(1−x2l)C12mω2l(1−x2l2)D12mω2l[1−xl]
Atube is completely filled with some incompressible liquid having total mass m. Length of the tube is L. Tube is rotated in horizontal plane, about one of its ends and force exerted by the tube on liquid on the other end is found to be 1nmLω2. Here ω is uniform angular velocity of tube. Calculate the value of n.
View SolutionA uniform material rod of length L is rotated in a horizontal plane about a vertical axis through one of its ends. The angular speed of rotation is w. Find increase in length of the rod. It is given that density and Young’s modulus of the rod are r and Y respectively.
View SolutionA thin uniform copper rod of length l and mass m rotates uniformly with an angular velocity ω in a horizontal plane about a vertical axis passing through one of its ends. Determine the tension in the rod as a function of the distance r from the rotation axis. Find the elongation of the rod.
View SolutionTwo particles P and Q are located at distance rpandrQ respectively form the centre of rotating disc such that rP>rQ. The disc is rotating with constant angular acceleration. We can say
View SolutionA coin is placed on a rotating platform at distance 'r' from its axis of rotation . To avoid the skidding of coin from rotating platform , the maximum angular velocity ω is
View Solution