A uniform circular disc of radius R, lying on a frictionless horizontal plane is rotating with an angular velocity omega about is its own axis. Another identical circular disc is gently placed on the top of the first disc coaxially. The loss in rotational kinetic energy due to friction between the two discs, as they acquire common angular velocity is (I is moment of inertia of the disc)Solution in Telugu
181ω2
141ω2
121ω2
1ω2
The correct Answer is:(b)
K.E of rotating circular disc =12Iω2
K.E of linear circular disc,
L=12mv2
Therefore, the resultant loss=12Iω2+12mv2=14Iω2
Topper's Solved these Questions
Similar Questions
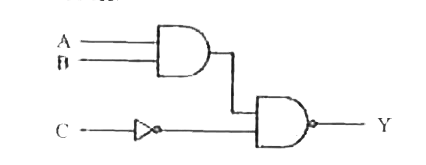
In the following circuit the output Y becomes zero for the input combinations
View SolutionThe specific heat of helium at constant volume is 12.6Jmol−1K−1. The specific heat of helium at constant pressure in Jmol−1K−1 is about
(Assume the temperature of the gas is moderate, universal gas constant, R=8.314Jmol−1K−1
View SolutionA uniform circular disc of radius R is rotating about its own axis with moment of inertia I at an angular velocity ω if a denser particle of mass m is gently attached to the rim of disc than its angular velocity is
View SolutionA circular disc is rotating without friction about its natural axis with an angular velocity omega . Another circular disc of same material and thickness but half the raduis is gently placed over it coaxially. The angular velocity of composite disc will be
View SolutionA thin uniform circular disc of mass M and radius R is rotating in a horizontal plane about an axis passing through its centre and perpendicular to the plane with angular velocity omega . Another disc of same mass but half the radius is gently placed over it coaxially. The angular speed ofthe composite disc will be:
View SolutionA thin uniform circular disc of mass M and radius R is rotating in a horizontal plane about an axis passing through its centre and perpendicular to its plane with an angular velocity omega . Another disc of same dimensions but of mass (1)/(4) M is placed gently on the first disc co-axially. The angular velocity of the system is
View SolutionA uniform disc of mass M and radius R is rotating in a horizontal plane about an axis perpendicular to its plane with an angular velocity ω. Another disc of mass M/3 and radius R/2 is placed gently on the first disc coaxial. Then final angular velocity of the system is
View SolutionA disc of moment of inertia la is rotating in a horizontal plane about its symmetry axis with constant angular speed co. Another discinitially at rest of moment of inertia I, is dropped coaxially on to the rotating disc. Then, both the discs rotate with same constant angular speed The loss of kinetic energy due to friction in this process is,
View SolutionA thin uniform circular disc of mass M and radius R is rotating in a horizontal plane about an axis perpendicular to the plane at an angular velocity ω . Another disc of mass M / 3 but same radius is placed gently on the first disc coaxially . the angular velocity of the system now is
View SolutionA disc of moment of inertia la is rotating in a horizontal plane about its symmetry axis with constant angular speed co. Another discinitially at rest of moment of inertia I, is dropped coaxially on to the rotating disc. Then, both the discs rotate with same constant angular speed The loss of kinetic energy due to friction in this process is,
View SolutionA circular disc is rotating about its natural axis with angular velocity of 10rads−1. A second disc of same mass is joined to it coaxially. If the radius of disc is half of the radius of the first, then they together rotate with an angular velocity of
View SolutionA thin uniform circular disc of mass M and radius R is rotating in a horizontal plane about an axis passing through its centre and perpendicular to the plane with n angular velocity ω another disc of one forth mass and same dimension is gently placed over it coaxially, the angular speed of the composite disc will be ............
View SolutionA circular disc is rotating about its natural axis with angular velocity of 10 rads-l. A second disc of same mass is joined to it coaxially. If the radius of disc is half of the radius of the first, then they together rotate with an angular velocity of
View SolutionA thin uniform circular disc of mass M and radius R is rotating in a horizontal plane about an axis passing through its centre and perpendicular to its plane with an angular velocity omega . Another disc of same dimensions but of mass M/4 is placed gently on the first disc coaxially. The angular velocity of the system now is
View SolutionA thin and circular disc of mass m and radius R is rotating in a horizontal plane about an axis passing through its centre and perpendicular to its plane with an angular velocity ω . If another disc of same dimension but mass ml4 is placed gently on the first disc co-axially, then the new angular velocity of the system is
View Solution
