A rod of length / is held vertically stationary with its lower end located at a point P, on the horizontal plane. When the rod is released to topple about P, the velocity of the upper end of the rod with which it hits the ground is Solution in Telugu
√gl
√gl
3√gl
√3gl
The correct Answer is:(b)
From the law of conservation of energy, the potential energy of the metre stick is converted into rotational kinetic energy.
P.E of metre stick= mgl2
Rotational K.E of a body = 12Iω2
⇒mg(1)2=12Iω2
Where, I=Moment of inertia of metre stick= (ml)23
⇒mg(l2)=12(ml)23(vl)2 (∵ω=vr=vl)
3gl=v2⇒v=√3gl
Topper's Solved these Questions
Similar Questions
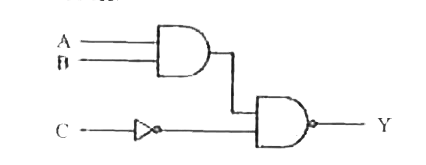
In the following circuit the output Y becomes zero for the input combinations
View SolutionThe specific heat of helium at constant volume is 12.6Jmol−1K−1. The specific heat of helium at constant pressure in Jmol−1K−1 is about
(Assume the temperature of the gas is moderate, universal gas constant, R=8.314Jmol−1K−1
View SolutionA rod of length l is pivoted about a horizontal , frictionless pin through one end. The rod is released from ret in a vertical position. Find the velocity of the CM of the rod when the rod is inclined at an angle theta from the vertical.
View SolutionA uniform rod AB hinged about a fixed point P is initially vertical. A rod is released from vertical position. When rod is in horizontal position: The acceleration of end B of the rod is
View SolutionA rod of length l whose lower end is fixed on a horizontal plane, starts toppling from the vertical position. The velocity of the upper end when it hits the ground is.
View SolutionA uniform rod smoothly pivoted at one of its ends is released from rest. If it swings in vertical plane, the maximum speed of the end P of the rod is. .
View SolutionA uniform rod of length l is held vertically on a horizontal floor fixing its lower end, the rod is allowed to fall onto the ground. Find (i) its angular velocity at that instant of reaching the ground (ii) The linear velocity with which the tip of rod hits the floor.
View SolutionConsider a rod of length l resting on a wall and the floor. If the velocity of the upper end of a rod A is v_(0) in downward direction, find the velocity of the lower end B at this iinstant, when the rod makes an angle theta with horizontal
View SolutionAB is a mass less rigid rod of length 2l. It is free to rotate in vertical plane about a horizontal axis passing through its end A. Equal point masses (m each) are stuck at the centre C and end Bof the rod. The rod is released from horizontal position. Write the tension in the rod when it becomes vertical.
View SolutionA rod of length L whose lower end is fixed along the horizontal plane starts to topple from the vertical position. The velocity of the upper end of the rod when it hits the ground is
View SolutionA thin rod MN free to rotation in the vertical plane about the fixed end N is held horizontal when the end M is released the speed of this end when the rod makes an angle alpha with the horizontal will be proportional to : (see figure)
View Solutionद्र्व्यमान m तथा लंबाई l की एक समरूप छड़ ऊर्ध्वाधर स्थिति के अपने निचले छोर पर क्लैप की गयी है । यह छड़ एक ऊर्ध्वाधर तल में मुक्त रूप में घूम सकती है । प्रारम्भ में छड़ को ऊपरी सिरे से पकड़कर रखा जाता है तथा इस स्थिति से इसे थोड़ा-सा घुमाकर छोड़ दिया जाता है । जब छड़ अपने सबसे नीचे की स्थिति में आए, उस समय छड़ का कोणीय वेग एवं उसके दूसरे सिरे पर रेखीय वेग निकालें ।
View SolutionA rod of length l is released from the vertical position on a smooth horizontal plane. What is the nature of motion of the free end of the rod ?
View SolutionA thin rod MN free to rotation in the vertical plane about the fixed end N is held horizontal when the end M is released the speed of this end when the rod makes an angle alpha with the horizontal will be proportional to : (see figure)
View SolutionA rod of length 1 is held vertically stationary with its lower end located at a point .P., on the horizontal plane. When the rod is released to topple about .P., the velocity of the upper end of the rod with which it hits the ground is
View Solution