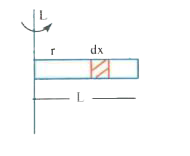
A uniform rod of length L is rotated in a horizontal plane about a vertical axis through one of its ends. The angular speed of rotation is $ω$ . Find increase in length of the rod, if $ρ$ and Y are the density and Young's modulus of the rod respectively,Solution in Telugu

A

$\frac{ρ o m η^{3} Y}{4 L^{2}}$

B

$\frac{4 ρ ω^{2} L^{3}}{3 Y}$

C

$\frac{ρ ω^{2} L^{3}}{3 Y}$

D

$\frac{ρ ω^{3} L^{3}}{8 Y}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

A uniform rod is rotated in a horizontal plane about a vertical axis through one of its ends.
Length of rod = L
Angular speed of rotation $= ω$
Density of rod $= ρ$
Young's modulus of rod $= Y$
The expression for Young's mondulus is given by,
$Y = \frac{Stress}{Strain}$
$Y = \frac{\frac{d F}{A}}{\frac{d l}{x}}$

$Y = \frac{d F . x}{d l . A}$
$d F = \frac{Y . A d l}{x} ... (1)$
But, centrifugal force due to mass is given by,
$d F = d m, x ω^{2}$
$= \frac{m d x}{L} x ω^{2} ... (2)$
From equations (1) and (2),
$\frac{Y A d l}{x} = \frac{m d x}{L} x ω^{2}$
$d l = \frac{m d x . x ω^{2}}{L . Y . A}$
Integration on both sides,
$\int d l = \int_{a}^{L} \frac{m d x x^{2} ω^{2}}{L . Y . A}$
$l = \frac{m ω^{2}}{L . Y . A} \int_{a}^{1} d x x^{2}$
$= \frac{m ω^{2}}{L . Y . A} \int_{a}^{L} d x x^{2}$
$= \frac{m ω^{2}}{L . Y . A} {[\frac{x^{3}}{3}]}_{0}^{L}$
$= \frac{m ω^{2} L^{3}}{3 L . Y . A}$
$= \frac{m}{A L} \frac{ω^{2} L^{3}}{3 Y}$
Increase in length of rod $= \frac{ρ ω^{2} L^{3}}{3 Y}$

Updated on:21/07/2023

|

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Similar Questions

SIA PUBLICATION-QUESTION PAPER -PHYSICS

Home
Ask Doubt
Profile