A cannon on a level plane is aimed at an angle θ above the horizontal and a shell is fired with a muzzle velocity v0 towards a vertical cliff a distance D away. Then the height from the bottom at which the shell strikes the side walls of the cliff is
Rsinα−12gR2v20sin2α
Rcosα−12gR2v20cos2α
Rtanα−12gR2v20sin2α
Rtanα−12gR2v20sin2α
The correct Answer is:C
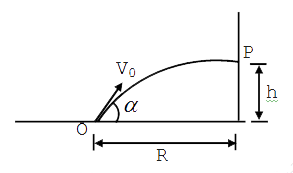
P should satisfy equation of trajectory
h=Rtanα−gR22v20cos2α
Similar Questions
A cannon of miass 5m (including a shell of mass m ) is at rest on a smooth horizontal ground. It fires the shell, with its barrel at an angle θ with the horizontal at a velocity u relative to itself. The horizontal' diśtance of the point where the shell strikes the ground from the initial position of the cannon is mu2sin2θng. Find (m+n),
View SolutionA target situated on a hill can be seen from the foot at angle α above the horizontal .The distance of the target along the horizonal is R. if a shell is fired from the foot at an angle β with the horizontal, find the velocity required for it to strike the target.
View Solution
Knowledge Check
A cannon of mass 5m (including a shell of mass m) is at rest on a smooth horizontal ground, fires the shell with its barrel at an angle θ with the horizontal at a velocity u relative to cannon. Find the horizontal distance of the point where shell strikes the ground from the initial position of the cannon :-
A4u2sin2θ5gBu2sin2θ5gC3u2sin2θ5gD8u2sin2θ5gA small ball thrown at an initial velocity v0 at an angle α to the horizontal strikes elastically with a smooth vertical wall moving towards it at a horizontal velocity v and returns to the point from which it was thrown. Determine the time t from the beginning of motions to the moment of impact with vertical wall. Neglect friction losses.
At=v0sinα(v0cosα+2v)g(v0cosα+v)Bt=v0sinα(v0cosα−2v)g(v0cosα+v)Ct=v0sinα(v0cosα+2v)g(v0cosα−v)Dt=v0cosα(v0sinα+2v)g(v0sinα+v)A projectile, thrown with velocity v0 at an angle α to the horizontal, has a range R. it will strike a vertical wall at a distance R/2 from the point of projection with a speed of
Av0Bv0sinαCv0cosαD√gR2
A shell is fired from a gun from the bottom of a hill along its slope. The slope of the hill is ∝=30∘ and the angle of the barrel to the horizontal β=60∘. The initial velocity v of the shell is 21ms−1. Then find the distance of point from the gun at which the shell will fall.
View SolutionA cannot fires from under a shelter inclined at an angle α to the horizontal (Fig.). The cannot is at point A at a (distance l from the base of the shelter (point B). The initial velocity of the shell is v0, and its trajectory lies in the plane of the figure. Determine the maximum range Lmax of the shell.

View SolutionA shell, which is initially located at a distance of 40.4 m above a horizontal plane, is fired horizontally with a muzzle velocity of 285 m/s to strike a target on the horizontal plane. (a) How long does the projectile remain in the air? (b) At what horizontal distance from the firing point does the shell strike the plane? What are the magnitudes of the (c ) horizontal and (d) vertical components of its velocity as it strikes the ground?
View SolutionA shot is fired with a velocity u at a very high vertical wall whose distance from the point of projection is x.The greatest height above the level of the point of projection at which the bullet can hit the wall is.
View SolutionTwo identical shells are fired from a point on the ground with same muzzle velocity at angle of elevation α=45∘ and β=tan−13 towards top of a cliff, 20m away from the point of firing. If both the shells reach the top simultaneously, then
height of the cliff is
View Solution
