A target situated on a hill can be seen from the foot at angle α above the horizontal .The distance of the target along the horizonal is R. if a shell is fired from the foot at an angle β with the horizontal, find the velocity required for it to strike the target.
The correct Answer is:√Rgcosα2sin(β−α)cosβ
Step by step video, text & image solution for A target situated on a hill can be seen from the foot at angle alpha above the horizontal .The distance of the target along the horizonal is R. if a shell is fired from the foot at an angle beta with the horizontal, find the velocity required for it to strike the target. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
The surface of a hill is inclined at an angle α to the horizontal .A stone is thrown from the summit of the hill at an angle β to the vertical with velocity v0.How far from the summit will the stone strike the ground ?
View SolutionThe surface of a hill is inclined at an angle α to the horizontal (Fig.). A stone is thrown from the summit of the hill at an initial speed v0 at an angle β to the vertical. How far from the summit will the stone strike the ground?
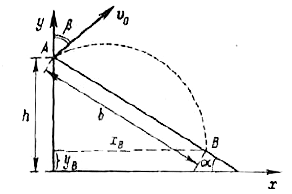
View Solution
Knowledge Check
A shot is fired at an angle α to the horizontal up a hill of inclination β to the horizontal. Then it strikes the hill horizontally if
Atanα=2tanβBtanα=tanβCtanα.tanβ=1DNoneA ladder rests against a vertical wall at angle α to the horizontal . If is foot is pulled away from the wall through a distance 'a' so that it slides a distance 'b' down the wall making the angle β with the horizontal , then a =
Aa=b tanα+β2Ba=b cotα+β2Catan(α−β)2DNoneA ladder rests against a vertical wall at angle α to the horizontal . If is foot is pulled away from the wall through a distance 'a' so that it slides a distance 'b' down the wall making the angle β with the horizontal , then a =
Abtan(α−β2)Bbtan(α+β2)Cbcot(α−β2)Dnone of these
A particle is thrown with speed u at an angle α with horizontal from the ground. After how much time, the velocity of particle will make an angle β with horizontal.
View SolutionA ladder rests against a wall making an angle alpha with the horizontal. The foot of the ladder is pulled away from the wall through a distance x so that it slides a distance y down the wall making an angle beta with the horizontal.The correct relation is (A) x=y tan(alpha+beta)/(2) (B) y=x tan(alpha+beta)/(2) (C) x=y tan(alpha+beta) (D) y=x tan(alpha+beta) (in)
View SolutionA ladder rests against a wall making an angle α with the horizontal. The foot of the ladder is pulled away from the wall through a distance ‘a’ so that it slides a distance ‘b’ down the wall making an angle beta with the horizontal then the value of ab is
View SolutionA shot is fired at an angle θ to the horizontal such that it strikes the hill while moving horizontally. Find the initial angle of projection θ.

View SolutionA particle is projected at an angle α with the horizontal from the foot of an inclined plane making an angle β with horizontal . Which of the following expressions holds good if the particle strikes the inclined plane normally?
View Solution
