A portion of length L is cut out of a conical solid wire the two ends of this portion have circular cross - sections of radii r1andr2(r2>r1). It is connected lengthwise to a circuit and a current I is flowing in it The resistivity of the material of the wire is ρ . Calculate the resistance of the considered portion and the voltage developed across it .
To solve the problem of finding the resistance of a conical solid wire and the voltage developed across it, we can follow these steps:
Step 1: Understand the Geometry of the Wire
We have a conical wire with a length L and two circular cross-sections at the ends with radii r1 and r2 (where r2>r1). The wire is connected to a circuit with current I flowing through it.
Step 2: Determine the Area of Cross-Sections
The area of a circular cross-section is given by:
A=πr2
Thus, the areas at the two ends of the wire are:
- At r1: A1=πr21
- At r2: A2=πr22
Step 3: Establish the Relationship Between Radius and Length
Since the wire is conical, the radius changes linearly from r1 to r2 as we move along the length L. We can express the radius r at a distance x from the smaller end as:
r(x)=r2−r1Lx+r1
Step 4: Calculate the Resistance of a Differential Element
The resistance dR of a small element dx of the wire can be expressed as:
dR=ρdxA
Substituting for A:
dR=ρdxπr(x)2
Substituting r(x):
dR=ρdxπ(r2−r1Lx+r1)2
Step 5: Integrate to Find Total Resistance
To find the total resistance R, we integrate dR from x=0 to x=L:
R=∫L0dR=∫L0ρdxπ(r2−r1Lx+r1)2
Step 6: Change of Variables for Integration
Let u=r2−r1Lx+r1. Then, du=r2−r1Ldx or dx=Lr2−r1du. The limits change as follows:
- When x=0, u=r1
- When x=L, u=r2
Thus, the integral becomes:
R=∫r2r1ρLπ(u2)(r2−r1)du
Step 7: Solve the Integral
The integral of 1u2 is:
∫1u2du=−1u
Evaluating from r1 to r2:
R=ρLπ(r2−r1)(−1u)r2r1=ρLπ(r2−r1)(−1r2+1r1)
This simplifies to:
R=ρLπ(1r1−1r2)1r2−r1
Step 8: Calculate Voltage Across the Wire
Using Ohm's law, the voltage V across the wire is given by:
V=I⋅R
Substituting the expression for R:
V=I⋅ρLπ(1r1−1r2)1r2−r1
Final Result
Thus, the resistance R of the conical wire is:
R=ρLπ(1r1−1r2)1r2−r1
And the voltage V developed across it is:
V=I⋅R
We have a conical wire with a length L and two circular cross-sections at the ends with radii r1 and r2 (where r2>r1). The wire is connected to a circuit with current I flowing through it.
The area of a circular cross-section is given by:
A=πr2
Thus, the areas at the two ends of the wire are:
- At r1: A1=πr21
- At r2: A2=πr22
Since the wire is conical, the radius changes linearly from r1 to r2 as we move along the length L. We can express the radius r at a distance x from the smaller end as:
r(x)=r2−r1Lx+r1
The resistance dR of a small element dx of the wire can be expressed as:
dR=ρdxA
Substituting for A:
dR=ρdxπr(x)2
Substituting r(x):
dR=ρdxπ(r2−r1Lx+r1)2
To find the total resistance R, we integrate dR from x=0 to x=L:
R=∫L0dR=∫L0ρdxπ(r2−r1Lx+r1)2
Let u=r2−r1Lx+r1. Then, du=r2−r1Ldx or dx=Lr2−r1du. The limits change as follows:
- When x=0, u=r1
- When x=L, u=r2
R=∫r2r1ρLπ(u2)(r2−r1)du
The integral of 1u2 is:
∫1u2du=−1u
Evaluating from r1 to r2:
R=ρLπ(r2−r1)(−1u)r2r1=ρLπ(r2−r1)(−1r2+1r1)
This simplifies to:
R=ρLπ(1r1−1r2)1r2−r1
Using Ohm's law, the voltage V across the wire is given by:
V=I⋅R
Substituting the expression for R:
V=I⋅ρLπ(1r1−1r2)1r2−r1
Thus, the resistance R of the conical wire is:
R=ρLπ(1r1−1r2)1r2−r1
And the voltage V developed across it is:
V=I⋅R
Topper's Solved these Questions
- CURRENT ELECTRICITYBook:MOTIONChapter:CURRENT ELECTRICITYExercise:EXERCISE -1 (SECTION -A Definition of Current , Current Density , Drift Velocity )
Explore 8 Videos
- CURRENT ELECTRICITYBook:MOTIONChapter:CURRENT ELECTRICITYExercise:EXERCISE -1(SECTION B- Resistance & resistivity , Ohms law +VI (Volt - curr.) Characteristics + Colour Code + Temp . Dependence
Explore 8 Videos
- CURRENT ELECTRICITYBook:MOTIONChapter:CURRENT ELECTRICITYExercise:EXERCISE -3 SECTION -B (PREVIOUS YEAR PROBLEMS JEE MAIN)
Explore 42 Videos
- CONSTRAINED MOTION Book:MOTIONChapter:CONSTRAINED MOTION Exercise:EXAMPLES
Explore 12 Videos
- ELASTICITYBook:MOTIONChapter:ELASTICITYExercise:EXERCISE -3
Explore 60 Videos
Similar Questions
A uniform wire of length 1 and radius r has resistance 100Ω. it is recast into a thin wire of (i) length 2l (ii) radius r/2. Calculate the resistance of new wire in each case.
View SolutionTwo wires of lengths l and 2l with resistivity of ρ and 3ρ respectively are connected in parallel.What is the equivalent resistance of the two wires if they have the same area of cross-section A
View Solution
Knowledge Check
The length and readius of a wire is l and r respectively. Its resistance is R. After hammering the radius of the wire is made (r/2) then the new resistance will be
A4RB8RC12RD16RA uniform current I is flowing in a long wire of radius R. If the current is uniformly distributed across the cross-sectional area of the wire, then
Amagnetic field increases linearly from centre to surfaceBmagnetic field decays inversely with distance r from the centre of wire for r>RCmagnetic field at the centre of wire is zeroDNone of the aboveLet us consider a copper wire having radius r and length l. Let its resistance be R. If the radius of another copper wire is 2r and the length is l/2 then the resistance of this, wire will be
ARB2RCR/4DR/8
Two wires of lengths l and 2l with resistivity of rho and 3 rho respectively are connected in parallel.What is the equivalent resistance of the two wires if they have the same area of cross- section A ?
View SolutionTwo wires of lengths _(l) and 2l with resistivity of rho and 3 rho respectively are connected in parallel.What is the equivalent resistance of the two wires if they have the same area of cross-section
View SolutionTwelve wires of equal length and same cross-section are connected in the form of a cube. If the resistance of each of the wires is R , then the effective resistance between the two diagonal ends would be
View SolutionA current i passes through a wire of length l , radius of cross-section r and resistivity ρ . The rate of heat generation is
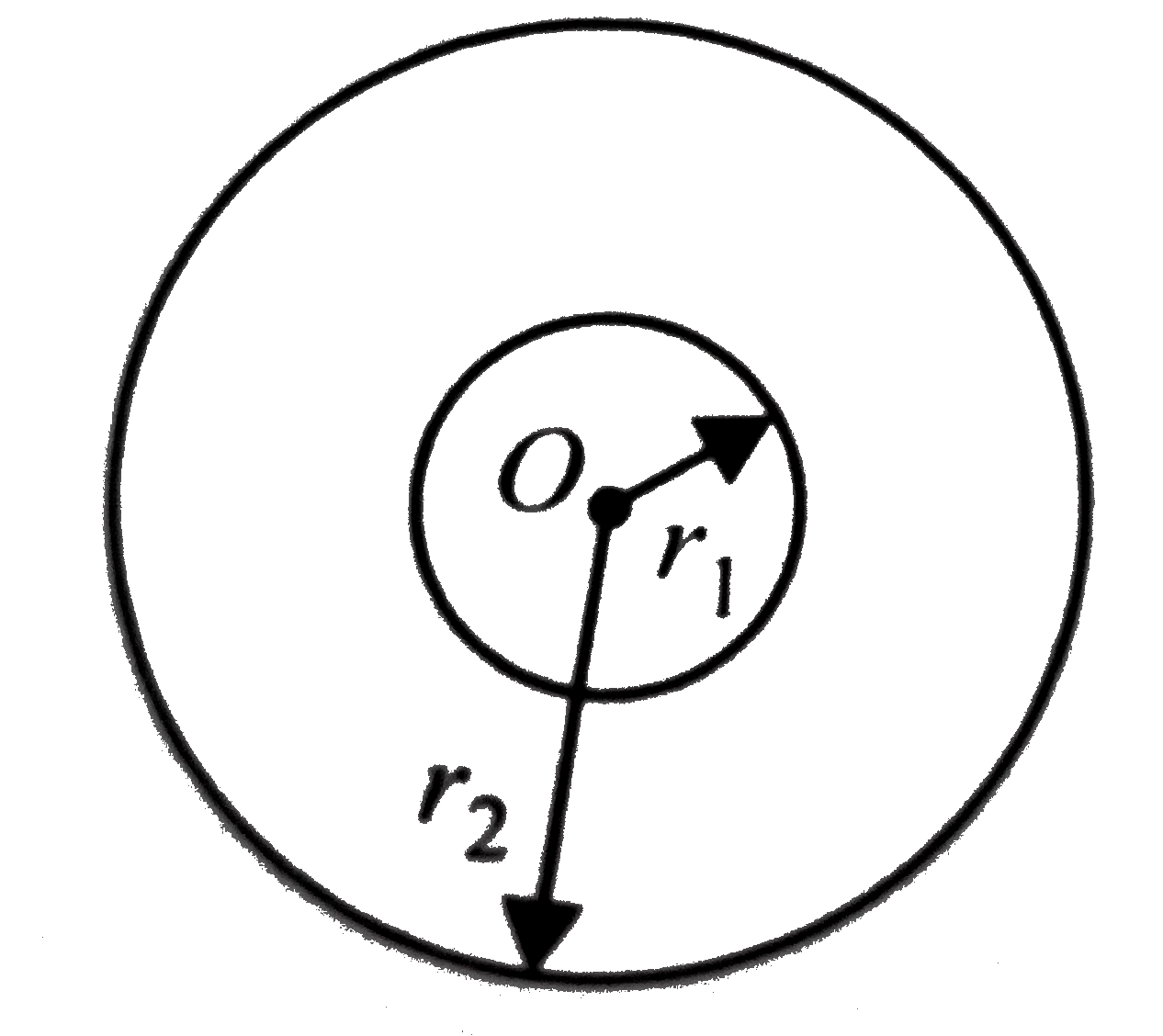
View SolutionSpace between tow concentric spheres of radii r1andr2 such that r1<r2 is filled with a material of resistivity ρ. Find the resistance between inner and outer surface of the material
View Solution