A 10 kg stone is suspended with a rope of breaking strength 30 kg-wt. The minimum time in which the stone can be raised through a height 10 m starting from rest is (Take, g=10Nkg−1).
0.5 s
1.0 s
√23s
2.0 s
The correct Answer is:B
Tension in the string T = mg
=30×10=300N
T−Mg=Ma
From the figure, 300−10×10=10a
∴ a=20ms−2
Thus, the maximum acceleration with which the stone can be raised is 20ms−2
Given, s = 10 m and u = 0
∴ 10=12(20)t2
⇒ t=1s
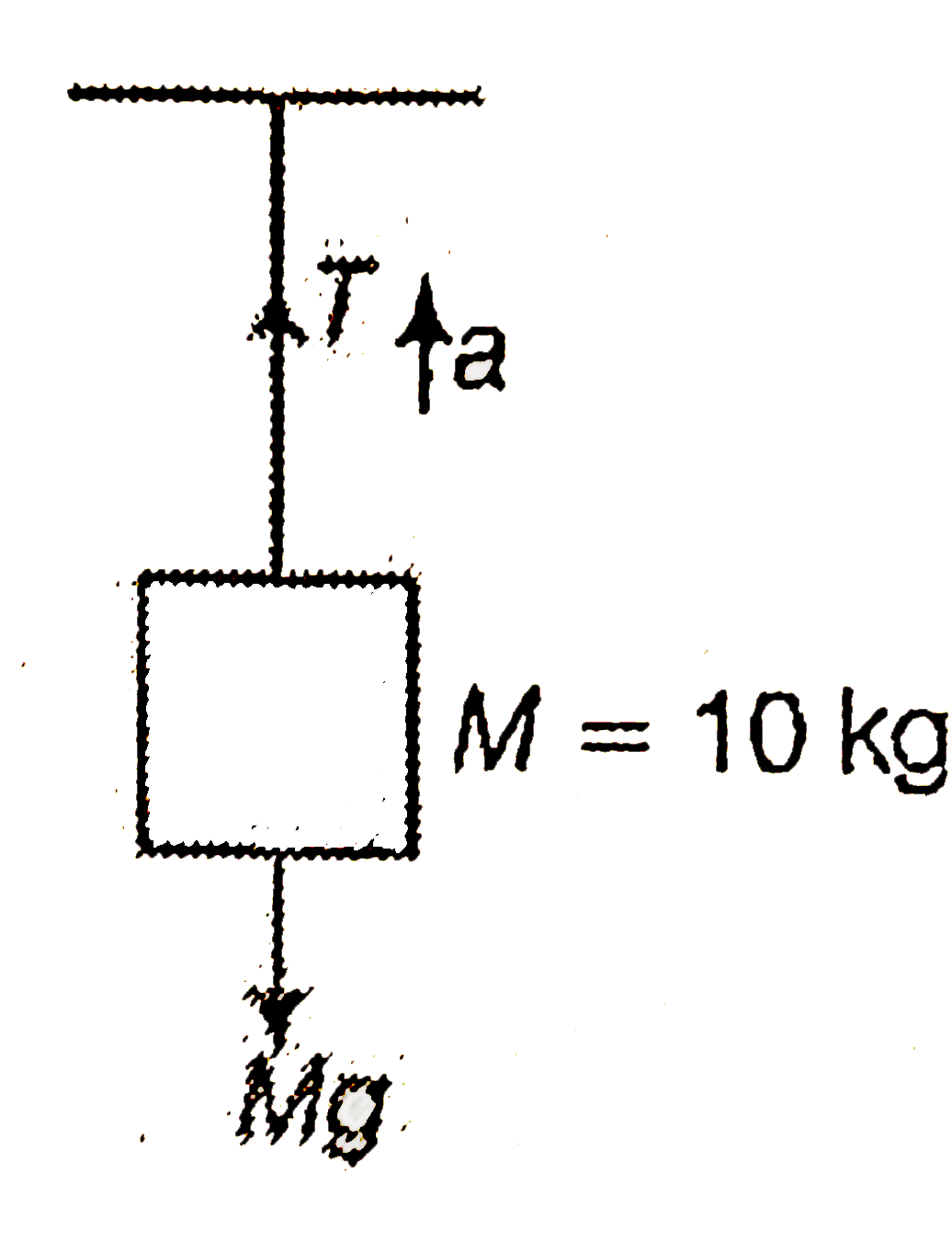
Related Playlists
- BOOK - BITSAT GUIDECHAPTER - NEWTONS LAWS OF MOTION AND FRICTIONEXERCISE - BITSAT Archives23videos
NEWTONS LAWS OF MOTION AND FRICTION
- BOOK - BITSAT GUIDECHAPTER - MOTION IN !,2,2 DIMENSIONS AND POJECTILE MOTIONEXERCISE - All Questions86videos
MOTION IN !,2,2 DIMENSIONS AND POJECTILE MOTION
- BOOK - BITSAT GUIDECHAPTER - ROTATIONAL MOTIONEXERCISE - BITSAT Archives9videos
ROTATIONAL MOTION
Similar Questions
A 10 kg stone is suspended with a rope of breaking strength 30 kg-wt. The minimum time in which the stone can be raised through a height 10 m starting from rest is (Take, g=10Nkg−1).
View SolutionRamesh raises on object of 12 kg through a height of 2.0m. The work done try him is (take g=10m/s2):
View SolutionA monkey of 25 kg is holding a vertical rope. The rope does not break if a body of mass 30 kg is suspended with the rope exceeds 30 kg. What will be the maximum acceleration with which the monkey can climb up along the rope ? ( Take ,=10ms−2)
View SolutionA monkey of mass 40 kg climbs on a massless rope of breaking strength 600 N. The rope will break if the monkey. (Take g=10m/s2)
View SolutionA balloon is going up with a uniform speed 20 m/s. It was at a height of 100 m from ground, when a stone is dropped from its basket. Find the time taken by the stone to reach the groimd and the height of the balloon from the ground, when stone hits the ground. (Take g=10m/s2)
View SolutionA 5kg stone of relative density 3 is resting at the bed of a lake. It is raised through a height of 5m in the lake. If g=10m/s2, then work done is
View SolutionA body of mass 60 kg is holding a vertical rope . The rope can break when a mass of 75 kg is suspended from it. The maximum acceleration with which the boy can climb the rope without breaking it is :(g=10m/s2)
View SolutionA monkey of mass 40kg climbs on a massless rope of breaking strenght 600N. The rope will break if the monkey (Take g=10m/s2) .
View SolutionA stone of mass 4 kg is attached to a string of 10 m length and is whirled in a horizontal circle . Calculate the max . Velocity with which the stone can be whirled if the string can withstand a maximum tension of 160 N .
View SolutionA stone of mass 5 kg is tied to a string of length 10 m is whirled round in a horizontal circle. What is the maximum speed with which the stone can be whirled around if the string can withstand a maximum tension of 200 N?
View Solution


