The length, a rod of aluminium is 1.0 m and its area of cross-section is 5.0cm2. Its one end is kept at 250∘C and the at 50∘C. How much heat will flow in the rod in 5.0 minutes . K for Al =2.0×10−1kJs−1m−1.∘C−1 and J=4.18cal−1
The correct Answer is:1435.4 cal
To solve the problem, we will use the formula for heat transfer through conduction:
Q=K⋅A⋅(T1−T2)L⋅t
Where:
- Q = heat transferred (in Joules)
- K = thermal conductivity (in kJ/s/m/°C)
- A = area of cross-section (in m²)
- T1 = temperature at one end (in °C)
- T2 = temperature at the other end (in °C)
- L = length of the rod (in m)
- t = time (in seconds)
Step 1: Convert the area of cross-section from cm² to m²
Given:
- Area A=5.0cm2
Convert to m²:
A=5.0cm2×(1m100cm)2=5.0×10−4m2
Step 2: Identify the given values
- Length of the rod L=1.0m
- Temperature difference T1−T2=250−50=200°C
- Thermal conductivity K=2.0×10−1kJ/s/m/°C=200J/s/m/°C (since 1kJ=1000J)
- Time t=5.0minutes=5×60=300s
Step 3: Substitute the values into the formula
Now, substituting the values into the heat transfer equation:
Q=K⋅A⋅(T1−T2)L⋅t
Q=200J/s/m/°C⋅5.0×10−4m2⋅200°C1.0m⋅300s
Step 4: Calculate Q
Calculating step-by-step:
1. Calculate K⋅A:
200⋅5.0×10−4=0.1J/°C
2. Calculate (T1−T2)L:
2001=200°C/m
3. Combine the results:
Q=0.1⋅200⋅300
Q=6000J
Step 5: Convert Joules to calories
Using the conversion 1cal=4.18J:
Q=6000J4.18J/cal≈1435.4cal
Final Answer
The total heat that will flow in the rod in 5.0 minutes is approximately 1435.4cal.
- Q = heat transferred (in Joules)
- K = thermal conductivity (in kJ/s/m/°C)
- A = area of cross-section (in m²)
- T1 = temperature at one end (in °C)
- T2 = temperature at the other end (in °C)
- L = length of the rod (in m)
- t = time (in seconds)
Given:
- Area A=5.0cm2
A=5.0cm2×(1m100cm)2=5.0×10−4m2
- Length of the rod L=1.0m
- Temperature difference T1−T2=250−50=200°C
- Thermal conductivity K=2.0×10−1kJ/s/m/°C=200J/s/m/°C (since 1kJ=1000J)
- Time t=5.0minutes=5×60=300s
Now, substituting the values into the heat transfer equation:
Q=K⋅A⋅(T1−T2)L⋅t
Q=200J/s/m/°C⋅5.0×10−4m2⋅200°C1.0m⋅300s
Calculating step-by-step:
1. Calculate K⋅A:
200⋅5.0×10−4=0.1J/°C
2001=200°C/m
Q=0.1⋅200⋅300
Q=6000J
Using the conversion 1cal=4.18J:
Q=6000J4.18J/cal≈1435.4cal
The total heat that will flow in the rod in 5.0 minutes is approximately 1435.4cal.
Topper's Solved these Questions
- THERMODYNAMICSBOOK - SL ARORACHAPTER - THERMODYNAMICSEXERCISE - Exercise
342 Videos
- THERMODYNAMICSBOOK - SL ARORACHAPTER - THERMODYNAMICSEXERCISE - Problems from competitive examinations
22 Videos
- Thermal Properties of MatterBOOK - SL ARORACHAPTER - Thermal Properties of MatterEXERCISE - Exercise
449 Videos
- Units and MeasurementsBOOK - SL ARORACHAPTER - Units and MeasurementsEXERCISE - Exercise
499 Videos
Similar Questions
Heat is flowing through a rod of length 25.0cm having cross-sectional area 8.80cm2. The coefficient of thermal conductivity for the material of the rod is K=9.2×10−2kcals−1m−1.∘C−1. The Temperatures of the ends of the rod are 125∘C and 0∘C in the steady state. Calculate (i) temeprature gradient in the rod (ii) temperature of a point at a distance of 10.0cm from the hot end and (iii) rate of flow of heat.
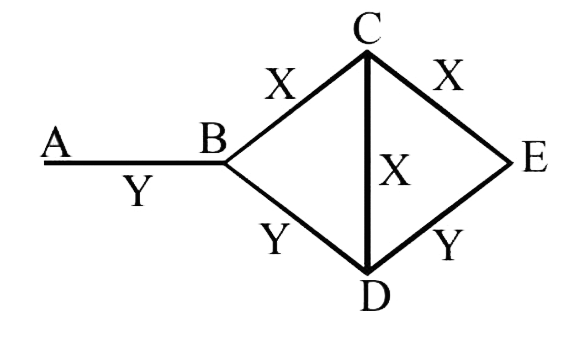
View SolutionThree rods of material X and three rods of material Y are connected as shown in the figure. All the rods are of identical length and cross-sectional area. If the end A is maintained at 60∘C and the junction E at 10∘C. Calculate the temperature of the junction B, C and D. The thermal conductivity of X is 0.92cal/sec−cm∘C and that of Y is 0.46cal/sec−cm−∘C.
View Solution
Knowledge Check
The heat is flowing through a rod of length 50 cm and area of cross-section 5cm2 . Its ends are respectively at 25∘C and 125∘C . The coefficient of thermal conductivity of the material of the rod is 0.092kcal/m×s×.∘C . The temperature gradient in the rod is
A2∘C/cmB2∘C/mC20∘C/mD20∘C/cmThe temperature of a rod of length 1 meter and area of cross-section 1cm2 is increased from 0∘C to 100∘C. If the rod is ot allowed to increase in length, the force required will be:
(α=10−6/∘CandY=1011N/m2)A103NB104NC105ND109NThe temperature of a wire of length 1 metre and area of cross-section 1cm2 is increased from 0∘C to 100∘C. If the rod is not allowed to increased in length, the force required will be (α=10−5/.∘CandY=1011N/m2)
A103NB104NC105ND109N
Find the thermal resistance of an aluminium rod of length 0.20 m and area of cross section 1×10−4m2. The heat current is along the length of the rod. [Thermal conductivity of aluminium=200Wm−1K−1]
View SolutionA uniform steel rod of length 50 cm is insulated on its sides. There is a layer of water of thick ness 0.2 mm at each end of the rod. If the ends of the rod are exposed to ice at 0∘C, and steam at 100∘C, calculate the temperature gradient in the rod. Thermal conductivities of steel and water are 0.11calcm−1s−1∘C−1and1.5×10−3calcm−1s−1∘C−1
View SolutionA metal rod has length of 100 cm and cross-section of 1.0cm2. By raising its temperture from 0∘C to 100∘C and holding it so that it is not permitted to expand or bend, the force developed is (Given Y=1012 dyne cm−2 and α=10−5.∘C−1)
View SolutionThe temperature of a wire length 1 m and area of cross-section 1cm2 is increased from 0∘C to 100∘C. If the rod is not allowed to increase in length, then the force, required will be (α=10−5∘C−1 and Y=1011Nm−2)
View SolutionA rod of 1m length and area of cross-section 1cm2 is connected across two heat reservoirs at temperature 100∘C and 0∘C as shown. The heat flow per second through the rod in steady state will be [Thermal conductivity of material of rod =0.09kilocalm−1s−1('∘C)]

View Solution
