Show that the centre of mass of uniform rod of mass M and length L lies at the middle point of the rod.
To show that the center of mass of a uniform rod of mass M and length L lies at the midpoint of the rod, we can follow these steps:
Step 1: Define the Rod and Coordinate System
We consider a uniform rod of length L lying along the x-axis. We can place one end of the rod at the origin (0, 0) and the other end at (L,0). The mass of the rod is M, and since it is uniform, the mass is evenly distributed along its length.
Step 2: Identify a Small Element of the Rod
To find the center of mass, we will consider a small element of the rod. Let’s denote a small segment of the rod with a length dx located at a distance x from the origin. The mass of this small element dm can be expressed as:
dm=ML⋅dx
This is because the mass per unit length (linear density) of the rod is ML.
Step 3: Set Up the Integral for the Center of Mass
The center of mass xcm of the rod can be calculated using the formula:
xcm=1M∫L0xdm
Substituting dm from the previous step, we have:
xcm=1M∫L0x(MLdx)
Step 4: Simplify the Integral
Now, we can simplify the expression:
xcm=1M⋅ML∫L0xdx
The M cancels out:
xcm=1L∫L0xdx
Step 5: Calculate the Integral
Now we compute the integral:
∫L0xdx=(x22)L0=L22
Substituting this back into the equation for xcm:
xcm=1L⋅L22=L2
Step 6: Conclusion
Thus, we find that the center of mass of the uniform rod is located at:
xcm=L2
This confirms that the center of mass lies at the midpoint of the rod.
We consider a uniform rod of length L lying along the x-axis. We can place one end of the rod at the origin (0, 0) and the other end at (L,0). The mass of the rod is M, and since it is uniform, the mass is evenly distributed along its length.
To find the center of mass, we will consider a small element of the rod. Let’s denote a small segment of the rod with a length dx located at a distance x from the origin. The mass of this small element dm can be expressed as:
dm=ML⋅dx
This is because the mass per unit length (linear density) of the rod is ML.
The center of mass xcm of the rod can be calculated using the formula:
xcm=1M∫L0xdm
Substituting dm from the previous step, we have:
xcm=1M∫L0x(MLdx)
Now, we can simplify the expression:
xcm=1M⋅ML∫L0xdx
The M cancels out:
xcm=1L∫L0xdx
Now we compute the integral:
∫L0xdx=(x22)L0=L22
Substituting this back into the equation for xcm:
xcm=1L⋅L22=L2
Thus, we find that the center of mass of the uniform rod is located at:
xcm=L2
This confirms that the center of mass lies at the midpoint of the rod.
Topper's Solved these Questions
- ROTATIONAL MOTIONBOOK - SL ARORACHAPTER - ROTATIONAL MOTIONEXERCISE - Problem
24 Videos
- ROTATIONAL MOTIONBOOK - SL ARORACHAPTER - ROTATIONAL MOTIONEXERCISE - Problem for self practice
90 Videos
- PROPERTIES OF SOLIDSBOOK - SL ARORACHAPTER - PROPERTIES OF SOLIDSEXERCISE - All Questions
97 Videos
- System of particles & rotational MotionBOOK - SL ARORACHAPTER - System of particles & rotational MotionEXERCISE - EXERCISE
353 Videos
Similar Questions
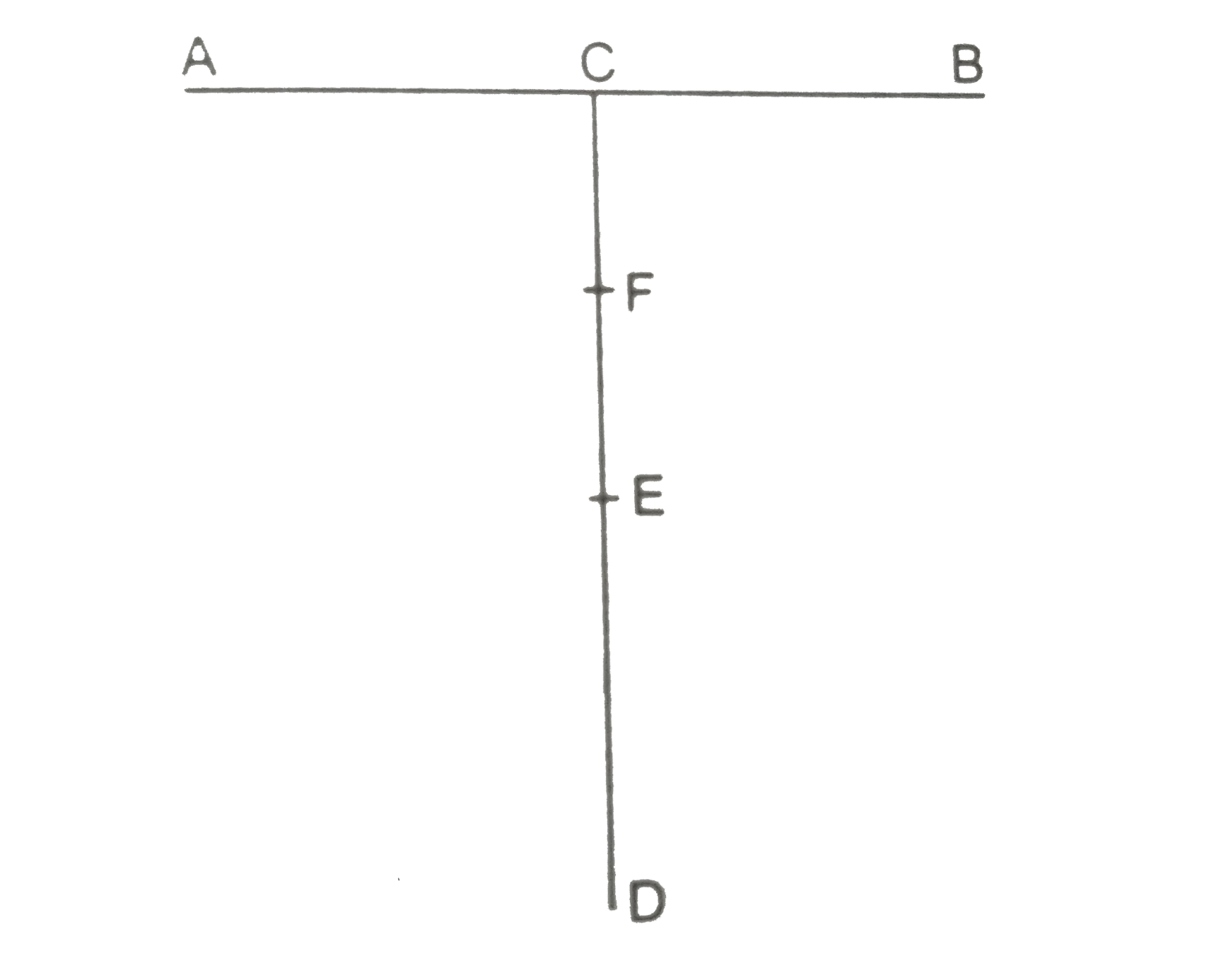
Two identical uniform rods AB and CD, each of length L are jointed to form a T-shaped frame as sown in figure. Locate the centre of mass of the frame. The centre of mass of a uniform rod is at the middle point of the rod.
View SolutionFind the M.I. of thin uniform rod of mass M and length L about the axis as shown.

View Solution
Knowledge Check
The moment of inertia of a thin uniform rod of mass M and length L about an axis perpendicular to the rod, through its centre is I. The moment of inertia of the rod about an axis perpendicular to rod through its end point is
AI4BI2C2ID4IThe moment of inertia of a thin uniform rod of mass M and length l about an axis perpendicular to the rod through its centre is I. The moment of inertia of the rod through its end point is
AI4BI2C2ID4IMoment of inertia of a thin rod of mass 'M' and length 'L' about the axis perpendicular to the rod and passing through its centre is ............
AML24BML26CML212DML22
Calculate the moment of inertia of a uniform rod of mass M and length l about an axis passing through an end and perpendicular to the rod. The rod can be divided into a number of mass elements along the length of the rod.

View SolutionFind the frequency of small oscillations of thin uniform vertical rod of mass m and length l hinged at the point O.

View Solutionthe moment of inertia of a uniform rod of mass 0.50 kg and length 1 m is 0.10 kg m^2 about a line perpendicular to the rod. Find the distance of this line from the middle point of the rod.
View SolutionMoment of inertia of a thin rod of mass 'M' and length 'L' perpendicular to rod at mid point is
View SolutionMoment of inertia of a uniform rod of mass m and length l is 712ml2 about a line perpendicular to the rod. Find the distance of this line from the middle point of the rod.
View Solution
