A ball of mass 100 g is suspended by a string 40cm long. Keeping the string taut, the ball describes a horizontal circle of radius 10 cm. Find the angular speed.
The correct Answer is:1.59rads−1
To find the angular speed of the ball suspended by a string while it describes a horizontal circle, we can follow these steps:
Step 1: Understand the Problem
We have a ball of mass m=100g=0.1kg suspended by a string of length L=40cm=0.4m. The ball is moving in a horizontal circle with a radius r=10cm=0.1m.
Step 2: Identify the Forces
The forces acting on the ball are:
1. The gravitational force Fg=mg acting downwards.
2. The tension T in the string acting at an angle θ from the vertical.
Step 3: Set Up the Geometry
From the geometry of the situation, we can relate the radius of the circle r, the length of the string L, and the angle θ:
- The vertical component of the tension must balance the weight of the ball:
Tcos(θ)=mg
- The horizontal component of the tension provides the centripetal force necessary for circular motion:
Tsin(θ)=mv2r
Step 4: Use Trigonometric Relationships
From the right triangle formed by the string, we can express sin(θ) and cos(θ):
- The vertical leg is Lcos(θ) and the horizontal leg is Lsin(θ).
- The radius r=Lsin(θ) gives us:
sin(θ)=rL=0.10.4=14
- Using cos2(θ)+sin2(θ)=1:
cos(θ)=√1−(14)2=√1−116=√1516=√154
Step 5: Substitute into the Equations
Substituting sin(θ) and cos(θ) into the equations:
1. For the vertical force balance:
T⋅√154=mg
T=mg⋅4√15
2. For the horizontal force balance:
T⋅14=mv2r
Step 6: Equate the Tension
Setting the two expressions for T equal:
mg⋅4√15⋅14=mv2r
This simplifies to:
mg√15=mv2r
Cancelling m and rearranging gives:
v2=g⋅r√15
Step 7: Calculate Angular Speed
The relationship between linear speed v and angular speed ω is:
v=rω⟹ω=vr
Substituting for v2:
ω2=g⋅r√15⋅r2=gr√15
Thus,
ω=√gr√15
Step 8: Plug in the Values
Using g≈9.81m/s2 and r=0.1m:
ω=√9.810.1⋅√15=√9.810.1⋅3.872=√9.810.3872≈√25.35≈5.035rad/s
Final Answer
The angular speed ω of the ball is approximately 5.035rad/s.
---
We have a ball of mass m=100g=0.1kg suspended by a string of length L=40cm=0.4m. The ball is moving in a horizontal circle with a radius r=10cm=0.1m.
The forces acting on the ball are:
1. The gravitational force Fg=mg acting downwards.
2. The tension T in the string acting at an angle θ from the vertical.
From the geometry of the situation, we can relate the radius of the circle r, the length of the string L, and the angle θ:
- The vertical component of the tension must balance the weight of the ball:
Tcos(θ)=mg
- The horizontal component of the tension provides the centripetal force necessary for circular motion:
Tsin(θ)=mv2r
From the right triangle formed by the string, we can express sin(θ) and cos(θ):
- The vertical leg is Lcos(θ) and the horizontal leg is Lsin(θ).
- The radius r=Lsin(θ) gives us:
sin(θ)=rL=0.10.4=14
- Using cos2(θ)+sin2(θ)=1:
cos(θ)=√1−(14)2=√1−116=√1516=√154
Substituting sin(θ) and cos(θ) into the equations:
1. For the vertical force balance:
T⋅√154=mg
T=mg⋅4√15
2. For the horizontal force balance:
T⋅14=mv2r
Setting the two expressions for T equal:
mg⋅4√15⋅14=mv2r
This simplifies to:
mg√15=mv2r
Cancelling m and rearranging gives:
v2=g⋅r√15
The relationship between linear speed v and angular speed ω is:
v=rω⟹ω=vr
Substituting for v2:
ω2=g⋅r√15⋅r2=gr√15
Thus,
ω=√gr√15
Using g≈9.81m/s2 and r=0.1m:
ω=√9.810.1⋅√15=√9.810.1⋅3.872=√9.810.3872≈√25.35≈5.035rad/s
The angular speed ω of the ball is approximately 5.035rad/s.
Topper's Solved these Questions
- ROTATIONAL MOTIONBOOK - SL ARORACHAPTER - ROTATIONAL MOTIONEXERCISE - Problem
24 Videos
- PROPERTIES OF SOLIDSBOOK - SL ARORACHAPTER - PROPERTIES OF SOLIDSEXERCISE - All Questions
97 Videos
- System of particles & rotational MotionBOOK - SL ARORACHAPTER - System of particles & rotational MotionEXERCISE - EXERCISE
353 Videos
Similar Questions
A ball of mass 0.1kg is suspended by a string 30cm long Keeping the string always taut the ball describe a horizontal circle of radius 15cm Calculate the angular speed of the ball .
View SolutionA particle of mass 21 g attached to a string of 70cm length Keeping the string always taut the ball describes a horizontal circle of radius 15cm Calculate the angular speed of the ball .
View Solution
Knowledge Check
A particle of mass m is suspended from the ceiling through a string of length L. The particle moves in a horizontal circle of radius r. The speed of the particle is
Arg√L2−r2Br√g(L2−r2)14Cr√g(L2−r2)12DmgL(L2−r2)12A body of mass 1 kg is suspended from a string 1 m long it is rotated in a vertical circle , what is the tension in the string when it is horizontal and the speed of the body is 2m/s ?
A4 NB3NC2 ND1NA ball of mass 500g tied to one end of string is revolved in a horizontal circle of radius 10cm with a speed 1/πrev/s in gravity-free space, then the linear velocity, acceleration and tension in the string will be
A0.1m/s,0.4m/s2,0.2NB0.1m/s,04m/s2,0.1NC0.2m/s,0.4m/s2,0.2ND0.2m/s,0.3m/s2,0.2N
Consider a conical pendulum having bob is mass m is suspended from a ceiling through a string of length L. The bob moves in a horizontal circle of radius r. Find (a) the angular speed of the bob and (b) the tension in the string.
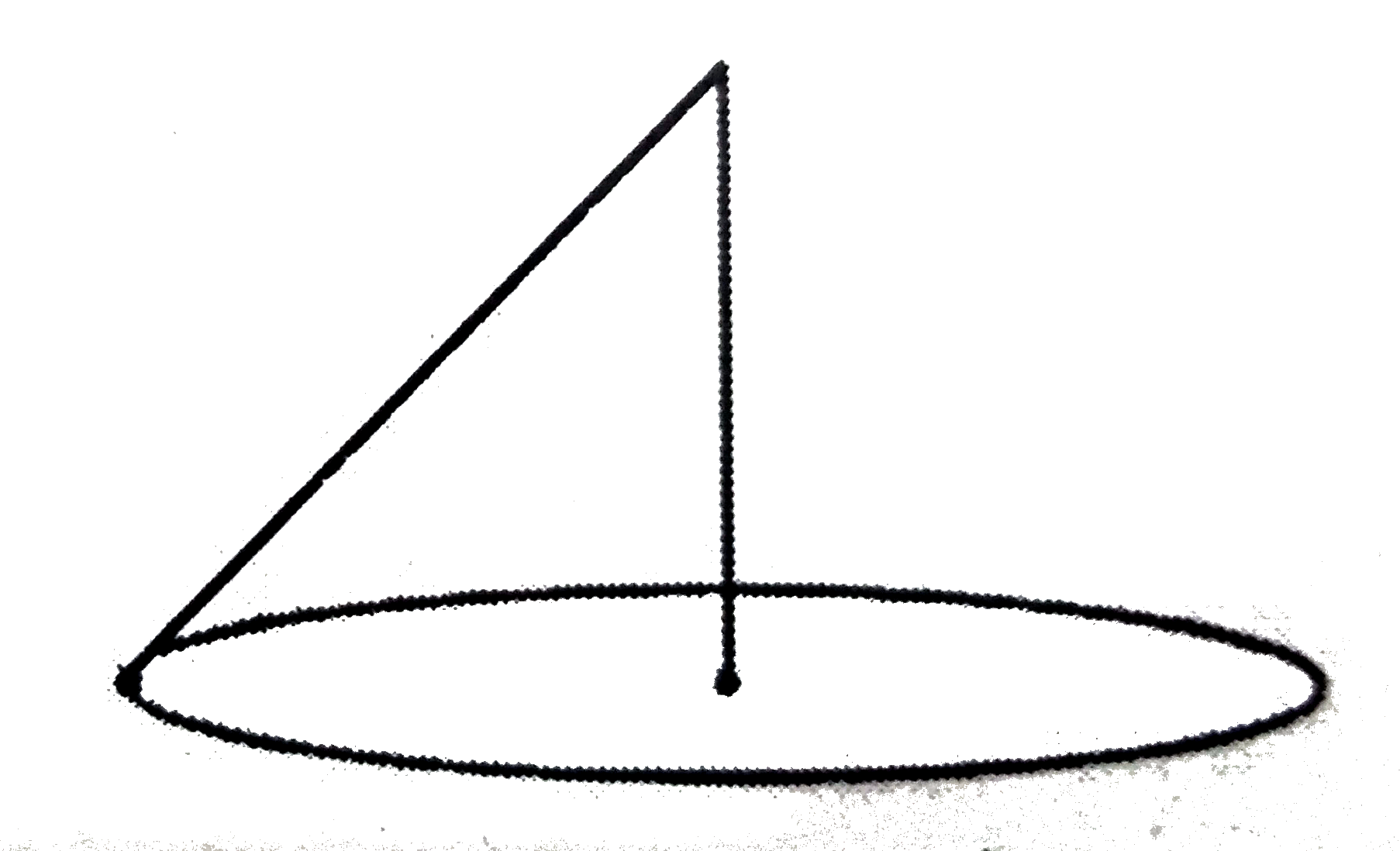
View SolutionA ball of mass m is suspended from a rope of length L. It describes a horizontal circle of radius r with speed v. the rope makes an angle θ with vertical given by sinθ=r/2. Determine (a) the tension in the rope and (b) the speed of the ball. (c) time period of ball.
View SolutionA body of mass 200g tied to one end of string is revolved in a horizontal circle of radius 50cm with angular speed 60 revolution per minute (rpm) on a smooth horizontal surface. Find (a) linear speed, (b) the acceleration and ( c) tension in the sting. what will happen if string is broken? (Take π2=10)
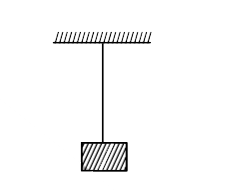
View SolutionA 'block' of mass 10 kg is suspended with string as shown in figure. Find tension in the string. (g=10m/s2)
View SolutionA body of mass 100g is tied to one end of a 2m long string. The other end of the string is at the centre of the horizontal circle. The maximum revolution in one minute is 200. The maximum tensible strength of the string is approx
View Solution
