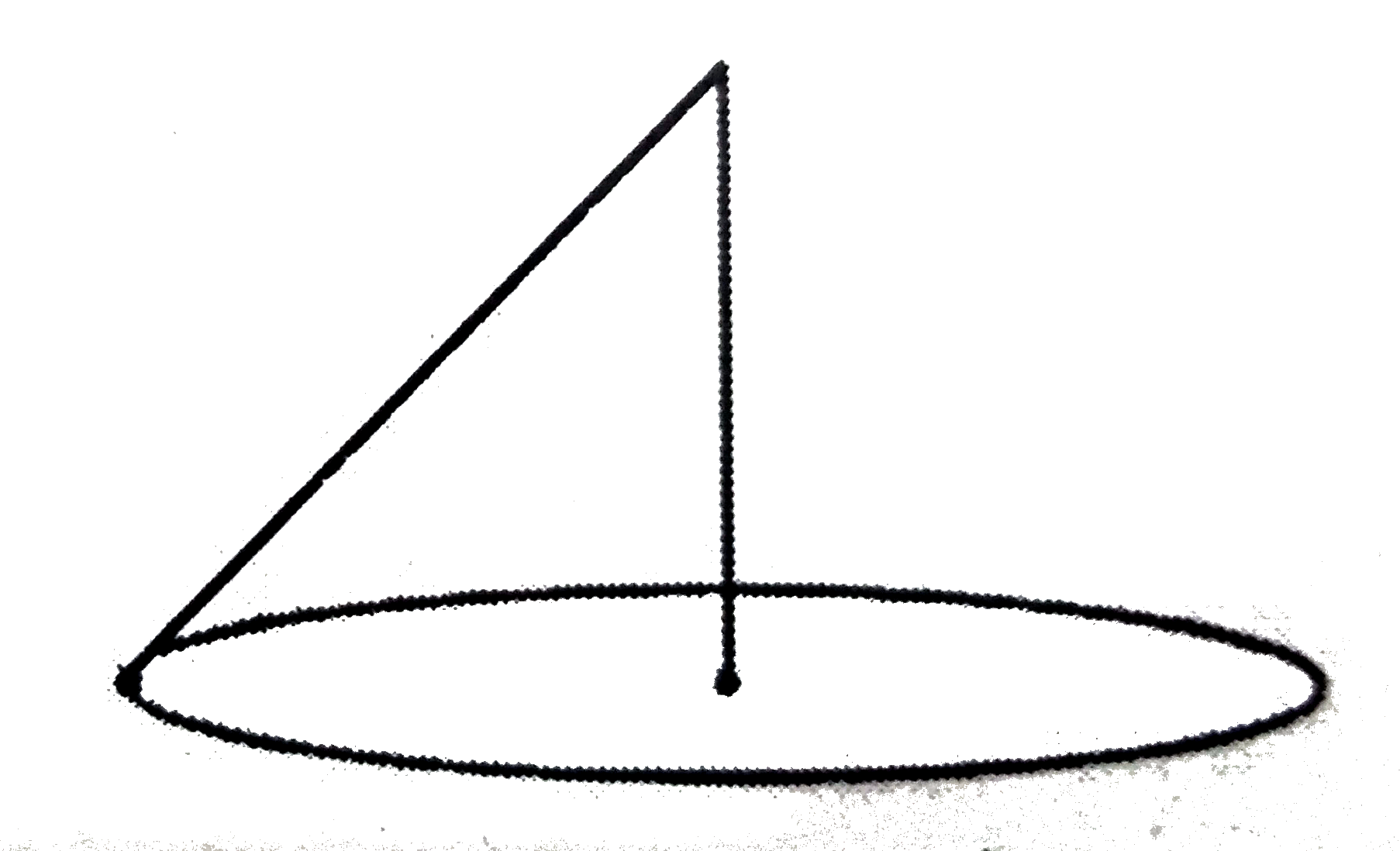
A ball of mass 0.1kg is suspended by a string 30cm long Keeping the string always taut the ball describe a horizontal circle of radius 15cm Calculate the angular speed of the ball .
The correct Answer is:6.14rad/s.
To calculate the angular speed of the ball, we can follow these steps:
Step 1: Understand the Geometry of the Problem
The ball is suspended by a string of length L=30cm=0.3m and describes a horizontal circle of radius r=15cm=0.15m. The string makes an angle θ with the vertical.
Step 2: Use Trigonometry to Find the Angle
Using the right triangle formed by the string, the vertical line, and the radius of the circle:
- The vertical side (adjacent to the angle θ) is Lcosθ.
- The horizontal side (opposite to the angle θ) is r.
From the geometry, we can write:
cosθ=rL
Substituting the values:
cosθ=0.150.3=12
Thus, θ=60∘.
Step 3: Apply Forces in the Vertical Direction
The forces acting on the ball are:
- The tension T in the string acting upwards.
- The weight mg acting downwards.
From the vertical component of the forces, we have:
Tcosθ=mg
Step 4: Apply Forces in the Horizontal Direction
In the horizontal direction, the tension provides the centripetal force required for circular motion:
Tsinθ=mv2r
Where v is the linear speed of the ball.
Step 5: Relate Linear Speed to Angular Speed
The relationship between linear speed v and angular speed ω is given by:
v=rω
Substituting this into the horizontal force equation gives:
Tsinθ=m(rω)2r=mrω2
Step 6: Solve for Tension
From the vertical force equation, we can express T:
T=mgcosθ
Step 7: Substitute Tension into the Horizontal Equation
Substituting T into the horizontal equation:
mgcosθsinθ=mrω2
Cancelling m from both sides:
gsinθcosθ=rω2
Using tanθ=sinθcosθ:
gtanθ=rω2
Step 8: Solve for Angular Speed ω
Rearranging gives:
ω2=gtanθr
Taking the square root:
ω=√gtanθr
Step 9: Substitute Known Values
Using g=9.81m/s2 and tan60∘=√3:
ω=√9.81⋅√30.15
Step 10: Calculate the Result
Calculating the above expression:
ω=√9.81⋅1.7320.15≈√113.14≈10.65rad/s
Final Answer
The angular speed of the ball is approximately 10.65rad/s.
---
The ball is suspended by a string of length L=30cm=0.3m and describes a horizontal circle of radius r=15cm=0.15m. The string makes an angle θ with the vertical.
Using the right triangle formed by the string, the vertical line, and the radius of the circle:
- The vertical side (adjacent to the angle θ) is Lcosθ.
- The horizontal side (opposite to the angle θ) is r.
cosθ=rL
Substituting the values:
cosθ=0.150.3=12
Thus, θ=60∘.
The forces acting on the ball are:
- The tension T in the string acting upwards.
- The weight mg acting downwards.
Tcosθ=mg
In the horizontal direction, the tension provides the centripetal force required for circular motion:
Tsinθ=mv2r
Where v is the linear speed of the ball.
The relationship between linear speed v and angular speed ω is given by:
v=rω
Substituting this into the horizontal force equation gives:
Tsinθ=m(rω)2r=mrω2
From the vertical force equation, we can express T:
T=mgcosθ
Substituting T into the horizontal equation:
mgcosθsinθ=mrω2
Cancelling m from both sides:
gsinθcosθ=rω2
Using tanθ=sinθcosθ:
gtanθ=rω2
Rearranging gives:
ω2=gtanθr
Taking the square root:
ω=√gtanθr
Using g=9.81m/s2 and tan60∘=√3:
ω=√9.81⋅√30.15
Calculating the above expression:
ω=√9.81⋅1.7320.15≈√113.14≈10.65rad/s
The angular speed of the ball is approximately 10.65rad/s.
Topper's Solved these Questions
- LAWS OF MOTIONBook:PRADEEPChapter:LAWS OF MOTIONExercise:JEE (Main and Advanced)/ Medical Entrance Special
Explore 93 Videos
- LAWS OF MOTIONBook:PRADEEPChapter:LAWS OF MOTIONExercise:Integer Type Questions
Explore 7 Videos
- LAWS OF MOTIONBook:PRADEEPChapter:LAWS OF MOTIONExercise:Fill in the blanks
Explore 20 Videos
- KINEMATICSBook:PRADEEPChapter:KINEMATICSExercise:1 NCERT Comprehension
Explore 4 Videos
- MATHEMATICAL TOOLSBook:PRADEEPChapter:MATHEMATICAL TOOLSExercise:Fill in the blanks
Explore 5 Videos
Similar Questions
A particle of mass 21 g attached to a string of 70cm length Keeping the string always taut the ball describes a horizontal circle of radius 15cm Calculate the angular speed of the ball .
View SolutionA ball of mass 100 g is suspended by a string 40cm long. Keeping the string taut, the ball describes a horizontal circle of radius 10 cm. Find the angular speed.
View Solution
Knowledge Check
A body of mass 1 kg is suspended from a string 1 m long it is rotated in a vertical circle , what is the tension in the string when it is horizontal and the speed of the body is 2m/s ?
A4 NB3NC2 ND1NA ball of mass 0.1 kg is suspended by a string. It is displaced through an angle of 60∘ and left. When the ball passes through the mean position, the tension in the string is
A19.6NB1.96NC9.8NDZeroA ball of mass (m)0.5kg is attached to the end of a string having length (L)0.5m. The ball is rotated on a horizontal circular path about vertical axis. The maximum tension that the string can bear is 324N. The maximum possible value of angular velocity of ball (in radian//s) is -
 .A9B18C27D36
.A9B18C27D36
Consider a conical pendulum having bob is mass m is suspended from a ceiling through a string of length L. The bob moves in a horizontal circle of radius r. Find (a) the angular speed of the bob and (b) the tension in the string.
View SolutionA ball of mass m is suspended from a rope of length L. It describes a horizontal circle of radius r with speed v. the rope makes an angle θ with vertical given by sinθ=r/2. Determine (a) the tension in the rope and (b) the speed of the ball. (c) time period of ball.
View SolutionA ball of mass 0.5 kg is attached to a light string of length 2m. The ball is whirled on la horizontal smooth surface is a circle of rdius 2 m as shown in fig. If the string can withstand tension up to 100 N, what is the maximum speed at which the bal can be whirled.
View SolutionA ball of mass 1kg is suspended by an inextensible string 1m long attached to a point O of a smooth horizontal bar resting on fixed smooth supports A and B. The ball is released from rest from the position when the string makes an angle 30∘ with the vertical. The mass of the bar is 4kg. The displacement of bar when ball reaches the other extreme position (in m) is

View SolutionA ball of mass 500g tied to one end of string is revolved in a horizontal circle of radius 10cm with a speed 1/πrev/s in gravity-free space, then the linear velocity, acceleration and tension in the string will be
View Solution