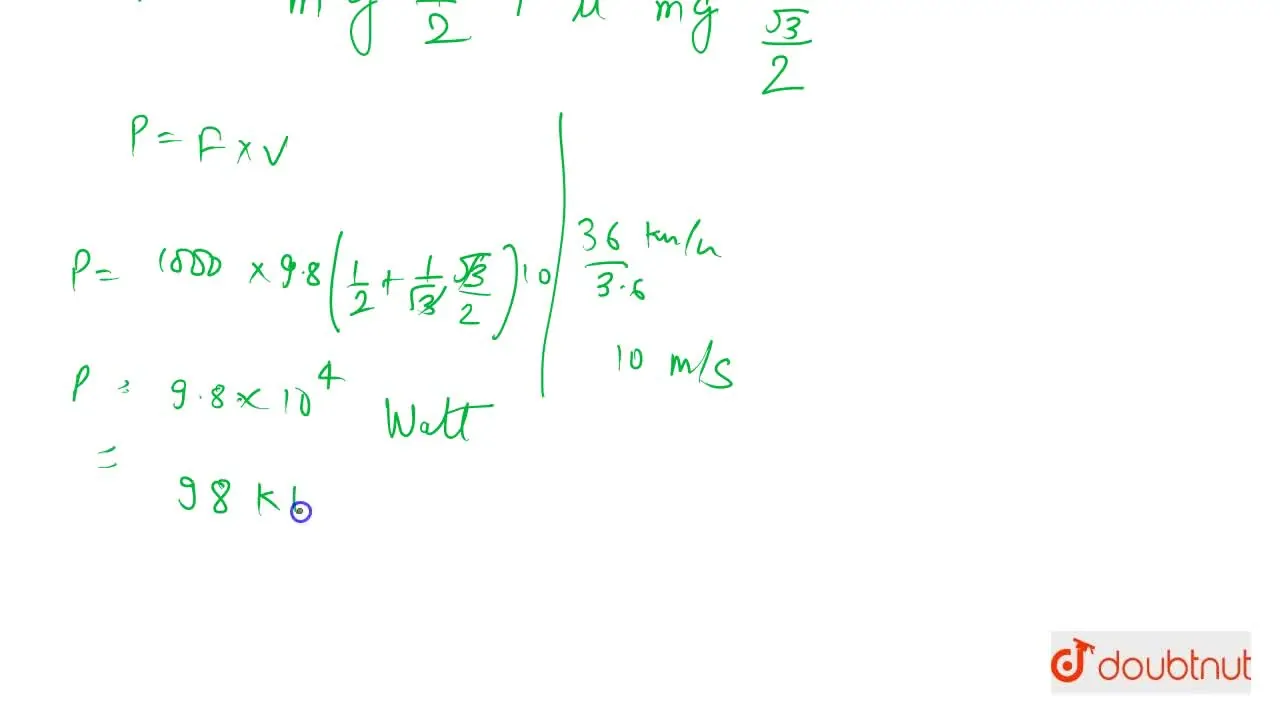An engine of one metric ton is going up an inclined plane of slope 1 in 2 at the rate of 36kmh−1 If the coefficient of friction is 1/√3 calculate the power of the engine in k W .
The correct Answer is:98kW .
Here, m=1 metric ton =103kg
sinθ=1/2,υ=36km/h=36×100060×60m/s=10m/s
μ=1√3P=?
cosθ=√1−sin2θ=√1−14=√32
As is known force required
F=mgsinθ+f=mgsinθ+μR
=mgsinθ+μmgcosθ
=mg(sinθ+μcosθ)
=103×9.8(12+1√3√32)
F=9.8×103×10watt
P=98kW .
Related Playlists
- BOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - JEE (Main and Advanced)/ Medical Entrance Special93videos
LAWS OF MOTION
- BOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Integer Type Questions7videos
LAWS OF MOTION
- BOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Fill in the blanks20videos
LAWS OF MOTION
- BOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 1 NCERT Comprehension4videos
KINEMATICS
- BOOK - PRADEEPCHAPTER - MATHEMATICAL TOOLSEXERCISE - Fill in the blanks5videos
MATHEMATICAL TOOLS
Similar Questions
An engine of weight 6.5 metric ton is going up an inclined plane of 5 in 13 at the rate of 9km/h. If the coefficient of friction is 1120. Calculate the power of the engine. Take g=9.8m/s2
View SolutionAn engine of mass 500kg is going up an inclined plane,3 in 5 at the rate of 36kmph.If the co-efficient of friction is 0.5.The power of engine is
View SolutionA train of mass 100 metric tons is drawn up an incline of 1 in 49 at the rate of 36 kph by a engine. If the resistance due to friction be 10N per metric ton, calculate the power of the engine if the steam is shut off, how far will the train move before it comes to rest ?
View SolutionAn engine draws a train up an incline of 1 in 100 at the rate 36km/h If the resistance due to friction is 5kg per metric ton calculate the power of the engine given mass of train and engine is 100 metic ton .
View SolutionA train of mass 106 kg is going up an incline plane with incline 1 in 49 at a rate of 10m/s if the resistance due to friction be 10 N per metric ton, calculate the power of the engine. If the engine is shut off, how far will the train move before it comes to rest ? take g=9.8m/s2
View SolutionAn engine of mass 6.5 metric ton is going up an incline of 5 in 13 at the rate of 9km/h Calculate the power of the engine if μ=1/2 and g=9.8m/s2.
View SolutionAn engine of 100H.P draws a train of mass 100 metic ton with a velocity of 36kmh−1 Find the coefficient of friction.
View SolutionAn engine of power 58.8kW pulls a train of mass 2×105kg with a velocity of 36kmh−1. The coefficient of static friction is
View SolutionFind the power of an engine which can draw a train of 400 metric ton up the inclined plane of 1 in 98 at the rate 10ms−1. The resistance due to friction acting on the train is 10N per ton.
View SolutionA 10 metric ton truck drives up a hilly road of gradient 1 in 50 at a speed of 36kmh−1 if the conefficient of kinetic friction between the road and years is 0.2, calculate the power delivered by the engine (g=10ms−2)
View Solution